Elastic Solver: Balancing Solution Time and Energy Consumption

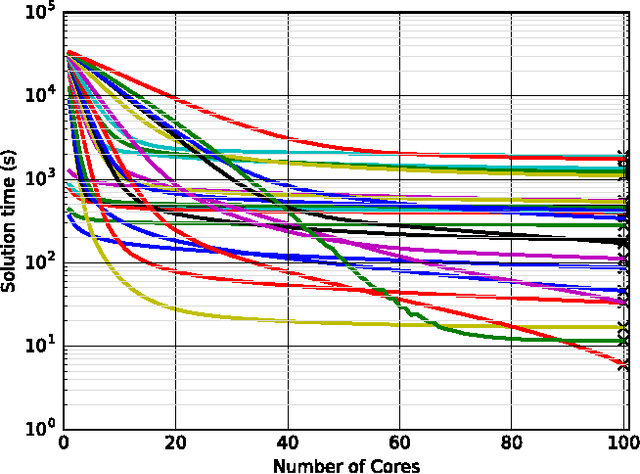
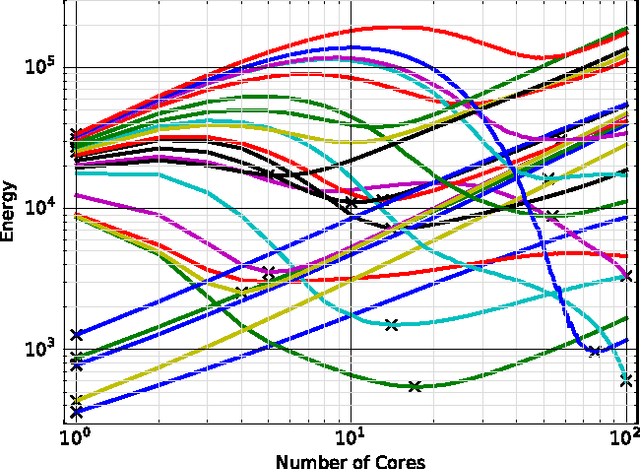
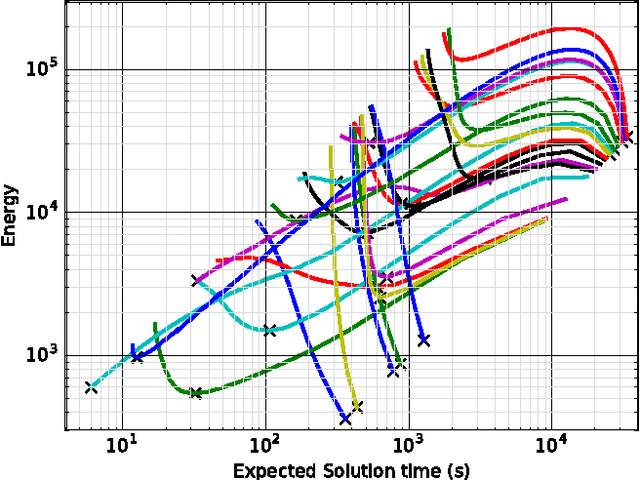
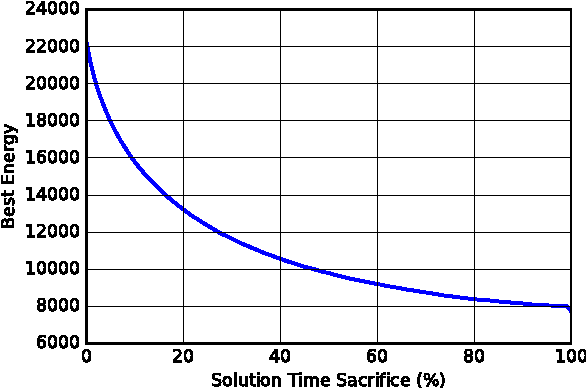
Combinatorial decision problems arise in many different domains such as scheduling, routing, packing, bioinformatics, and many more. Despite recent advances in developing scalable solvers, there are still many problems which are often very hard to solve. Typically the most advanced solvers include elements which are stochastic in nature. If a same instance is solved many times using different seeds then depending on the inherent characteristics of a problem instance and the solver, one can observe a highly-variant distribution of times spanning multiple orders of magnitude. Therefore, to solve a problem instance efficiently it is often useful to solve the same instance in parallel with different seeds. With the proliferation of cloud computing, it is natural to think about an elastic solver which can scale up by launching searches in parallel on thousands of machines (or cores). However, this could result in consuming a lot of energy. Moreover, not every instance would require thousands of machines. The challenge is to resolve the tradeoff between solution time and energy consumption optimally for a given problem instance. We analyse the impact of the number of machines (or cores) on not only solution time but also on energy consumption. We highlight that although solution time always drops as the number of machines increases, the relation between the number of machines and energy consumption is more complicated. In many cases, the optimal energy consumption may be achieved by a middle ground, we analyse this relationship in detail. The tradeoff between solution time and energy consumption is studied further, showing that the energy consumption of a solver can be reduced drastically if we increase the solution time marginally. We also develop a prediction model, demonstrating that such insights can be exploited to achieve faster solutions times in a more energy efficient manor.

Developing Approaches for Solving a Telecommunications Feature Subscription Problem

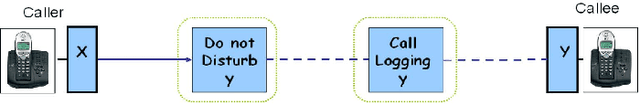
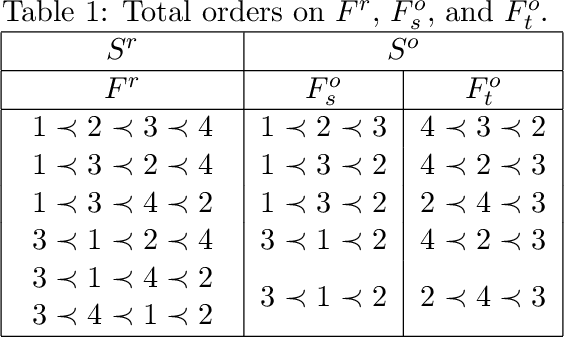
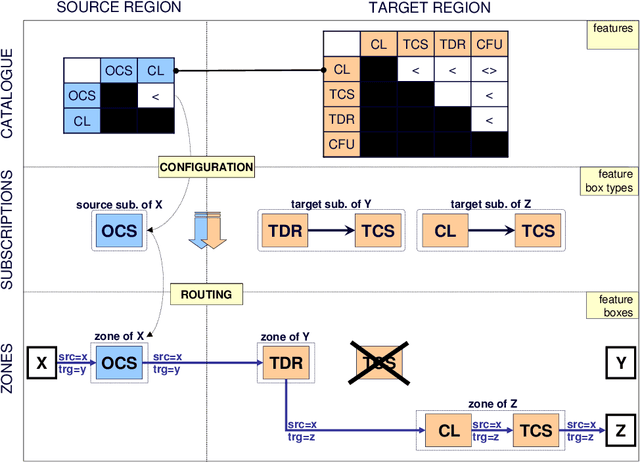
Call control features (e.g., call-divert, voice-mail) are primitive options to which users can subscribe off-line to personalise their service. The configuration of a feature subscription involves choosing and sequencing features from a catalogue and is subject to constraints that prevent undesirable feature interactions at run-time. When the subscription requested by a user is inconsistent, one problem is to find an optimal relaxation, which is a generalisation of the feedback vertex set problem on directed graphs, and thus it is an NP-hard task. We present several constraint programming formulations of the problem. We also present formulations using partial weighted maximum Boolean satisfiability and mixed integer linear programming. We study all these formulations by experimentally comparing them on a variety of randomly generated instances of the feature subscription problem.

A Combinatorial Optimisation Approach to Designing Dual-Parented Long-Reach Passive Optical Networks

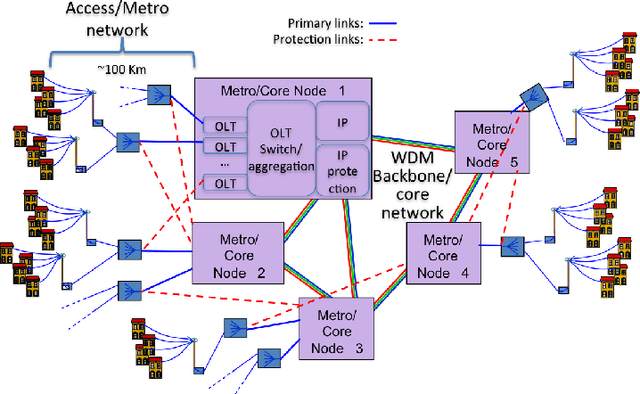
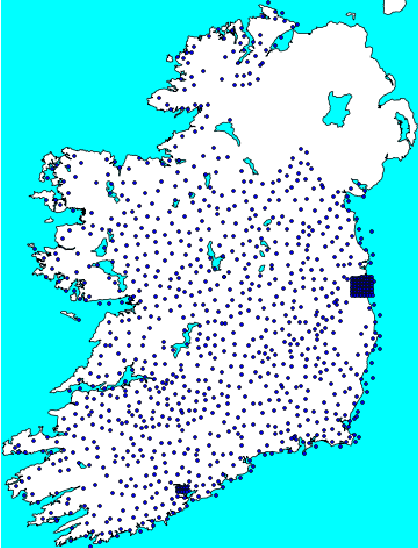
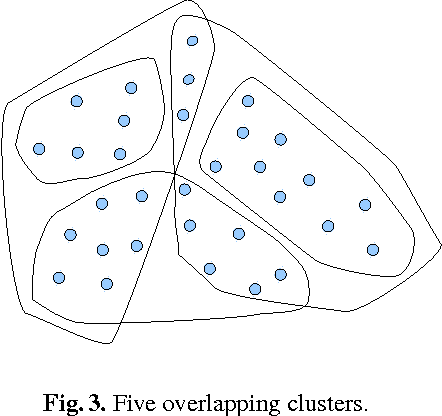
We present an application focused on the design of resilient long-reach passive optical networks. We specifically consider dual-parented networks whereby each customer must be connected to two metro sites via local exchange sites. An important property of such a placement is resilience to single metro node failure. The objective of the application is to determine the optimal position of a set of metro nodes such that the total optical fibre length is minimized. We prove that this problem is NP-Complete. We present two alternative combinatorial optimisation approaches to finding an optimal metro node placement using: a mixed integer linear programming (MIP) formulation of the problem; and, a hybrid approach that uses clustering as a preprocessing step. We consider a detailed case-study based on a network for Ireland. The hybrid approach scales well and finds solutions that are close to optimal, with a runtime that is two orders-of-magnitude better than the MIP model.