Debprakash Patnaik
Streaming Algorithms for Pattern Discovery over Dynamically Changing Event Sequences
May 21, 2012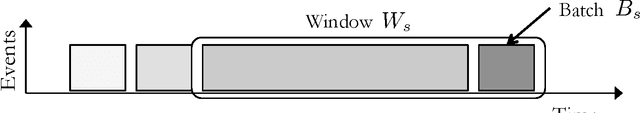
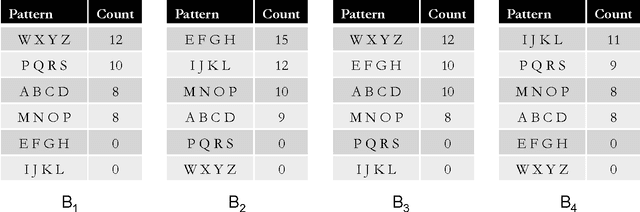
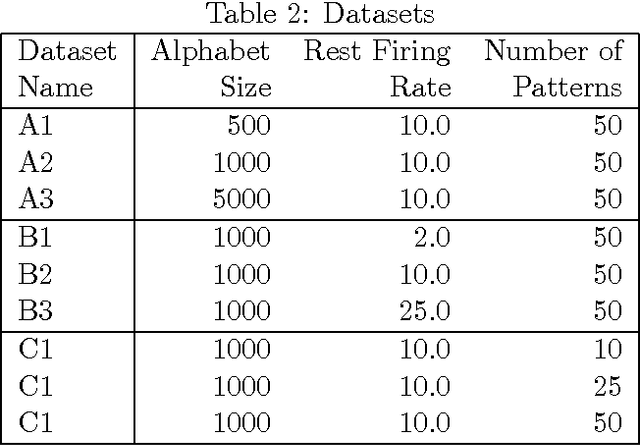
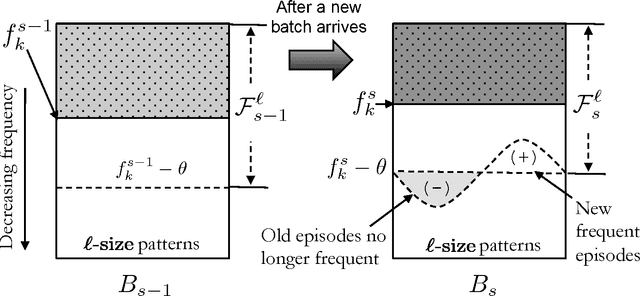
Abstract:Discovering frequent episodes over event sequences is an important data mining task. In many applications, events constituting the data sequence arrive as a stream, at furious rates, and recent trends (or frequent episodes) can change and drift due to the dynamical nature of the underlying event generation process. The ability to detect and track such the changing sets of frequent episodes can be valuable in many application scenarios. Current methods for frequent episode discovery are typically multipass algorithms, making them unsuitable in the streaming context. In this paper, we propose a new streaming algorithm for discovering frequent episodes over a window of recent events in the stream. Our algorithm processes events as they arrive, one batch at a time, while discovering the top frequent episodes over a window consisting of several batches in the immediate past. We derive approximation guarantees for our algorithm under the condition that frequent episodes are approximately well-separated from infrequent ones in every batch of the window. We present extensive experimental evaluations of our algorithm on both real and synthetic data. We also present comparisons with baselines and adaptations of streaming algorithms from itemset mining literature.
Inferring Dynamic Bayesian Networks using Frequent Episode Mining
Apr 14, 2009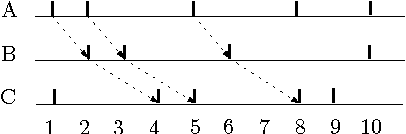
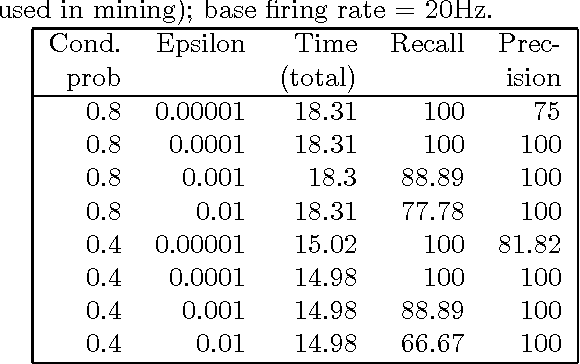
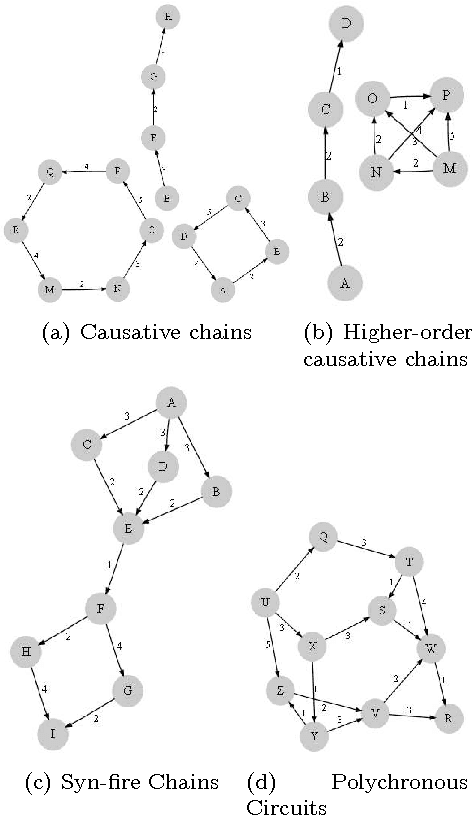
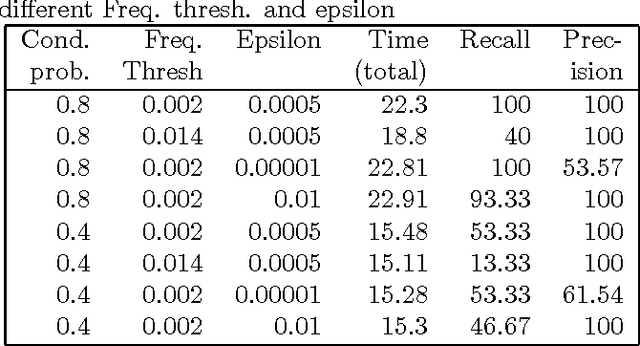
Abstract:Motivation: Several different threads of research have been proposed for modeling and mining temporal data. On the one hand, approaches such as dynamic Bayesian networks (DBNs) provide a formal probabilistic basis to model relationships between time-indexed random variables but these models are intractable to learn in the general case. On the other, algorithms such as frequent episode mining are scalable to large datasets but do not exhibit the rigorous probabilistic interpretations that are the mainstay of the graphical models literature. Results: We present a unification of these two seemingly diverse threads of research, by demonstrating how dynamic (discrete) Bayesian networks can be inferred from the results of frequent episode mining. This helps bridge the modeling emphasis of the former with the counting emphasis of the latter. First, we show how, under reasonable assumptions on data characteristics and on influences of random variables, the optimal DBN structure can be computed using a greedy, local, algorithm. Next, we connect the optimality of the DBN structure with the notion of fixed-delay episodes and their counts of distinct occurrences. Finally, to demonstrate the practical feasibility of our approach, we focus on a specific (but broadly applicable) class of networks, called excitatory networks, and show how the search for the optimal DBN structure can be conducted using just information from frequent episodes. Application on datasets gathered from mathematical models of spiking neurons as well as real neuroscience datasets are presented. Availability: Algorithmic implementations, simulator codebases, and datasets are available from our website at http://neural-code.cs.vt.edu/dbn
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge