Diverse M-Best Solutions by Dynamic Programming

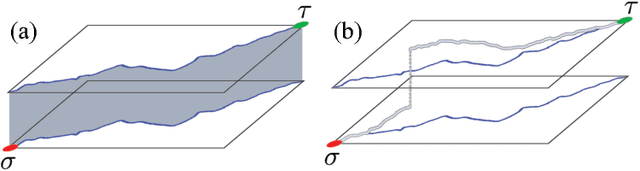
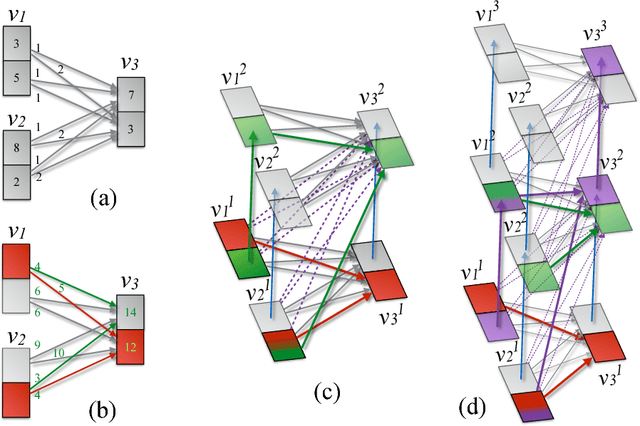
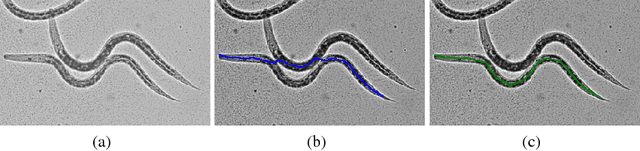
Many computer vision pipelines involve dynamic programming primitives such as finding a shortest path or the minimum energy solution in a tree-shaped probabilistic graphical model. In such cases, extracting not merely the best, but the set of M-best solutions is useful to generate a rich collection of candidate proposals that can be used in downstream processing. In this work, we show how M-best solutions of tree-shaped graphical models can be obtained by dynamic programming on a special graph with M layers. The proposed multi-layer concept is optimal for searching M-best solutions, and so flexible that it can also approximate M-best diverse solutions. We illustrate the usefulness with applications to object detection, panorama stitching and centerline extraction. Note: We have observed that an assumption in section 4 of our paper is not always fulfilled, see the attached corrigendum for details.

Globally Optimal Cell Tracking using Integer Programming

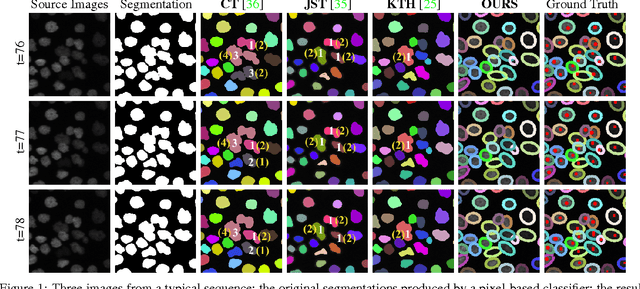
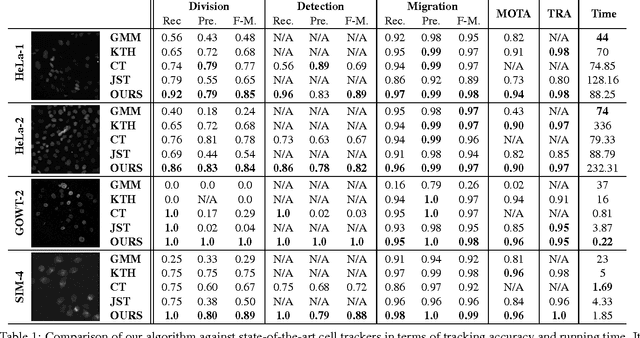
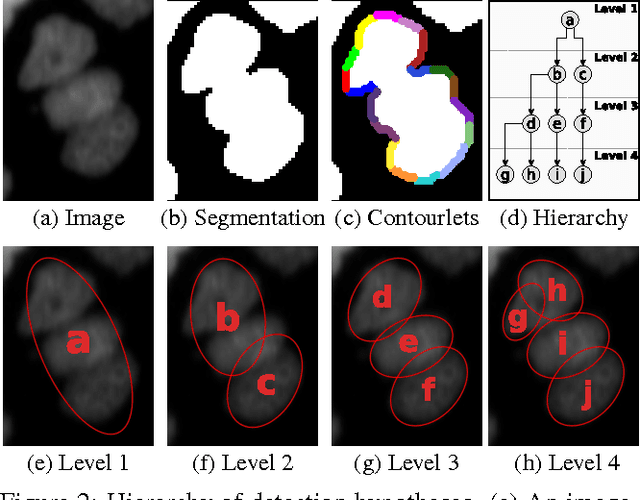
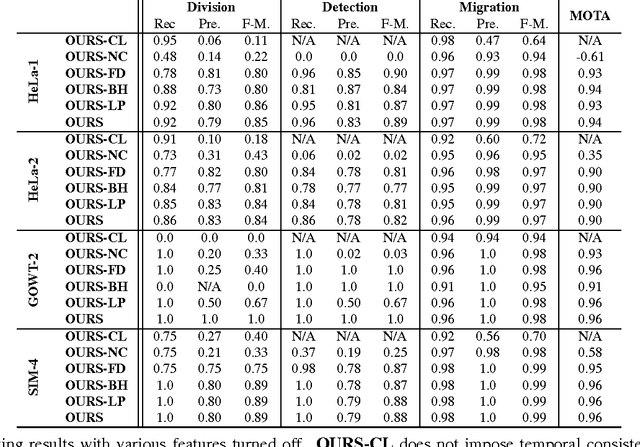
We propose a novel approach to automatically tracking cell populations in time-lapse images. To account for cell occlusions and overlaps, we introduce a robust method that generates an over-complete set of competing detection hypotheses. We then perform detection and tracking simultaneously on these hypotheses by solving to optimality an integer program with only one type of flow variables. This eliminates the need for heuristics to handle missed detections due to occlusions and complex morphology. We demonstrate the effectiveness of our approach on a range of challenging sequences consisting of clumped cells and show that it outperforms state-of-the-art techniques.