Membership-Mappings for Practical Secure Distributed Deep Learning

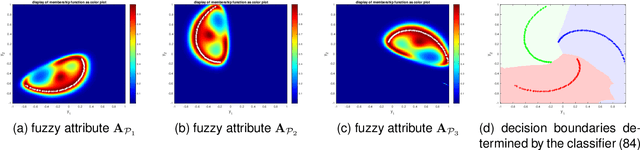
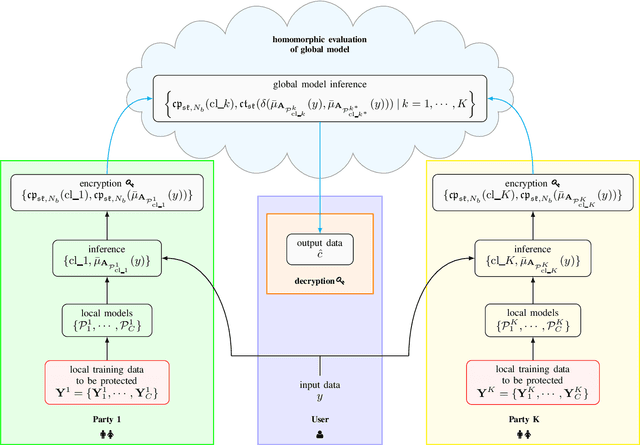
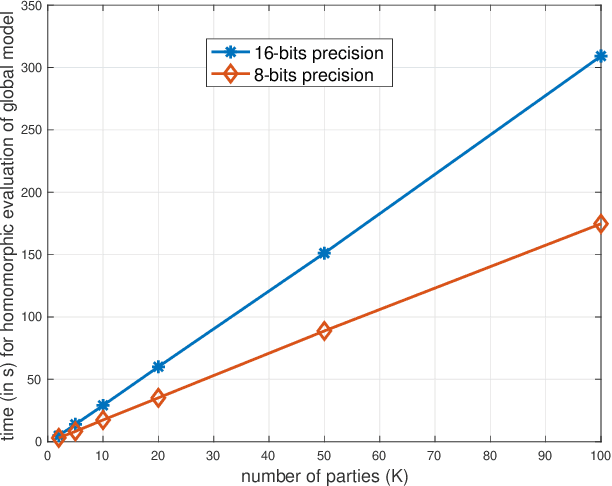
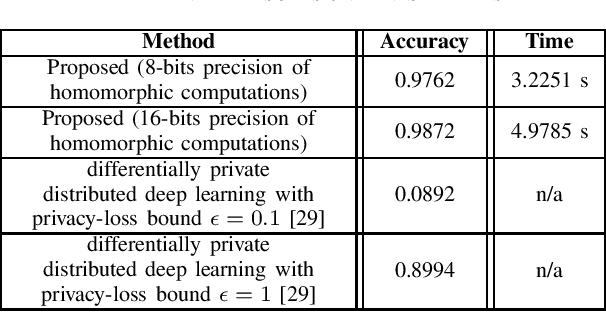
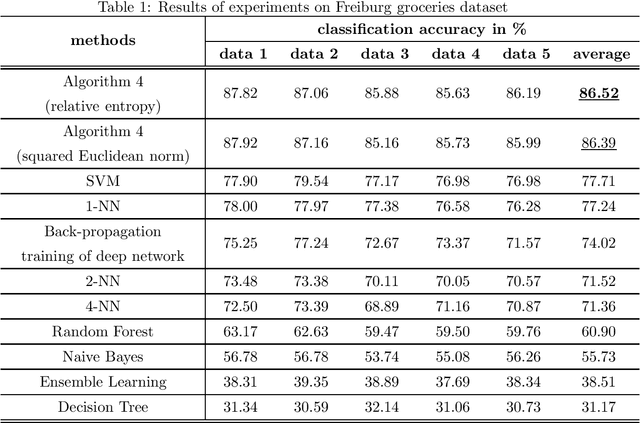
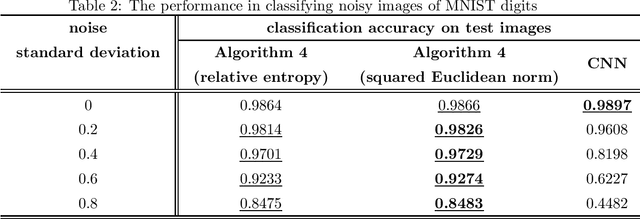
This study leverages the data representation capability of fuzzy based membership-mappings for practical secure distributed deep learning using fully homomorphic encryption. The impracticality issue of secure machine (deep) learning with fully homomorphic encrypted data, arising from large computational overhead, is addressed via applying fuzzy attributes. Fuzzy attributes are induced by globally convergent and robust variational membership-mappings based local deep models. Fuzzy attributes combine the local deep models in a robust and flexible manner such that the global model can be evaluated homomorphically in an efficient manner using a boolean circuit composed of bootstrapped binary gates. The proposed method, while preserving privacy in a distributed learning scenario, remains accurate, practical, and scalable. The method is evaluated through numerous experiments including demonstrations through MNIST dataset and Freiburg Groceries Dataset. Further, a biomedical application related to mental stress detection on individuals is considered.

Information Theoretic Evaluation of Privacy-Leakage, Interpretability, and Transferability for a Novel Trustworthy AI Framework

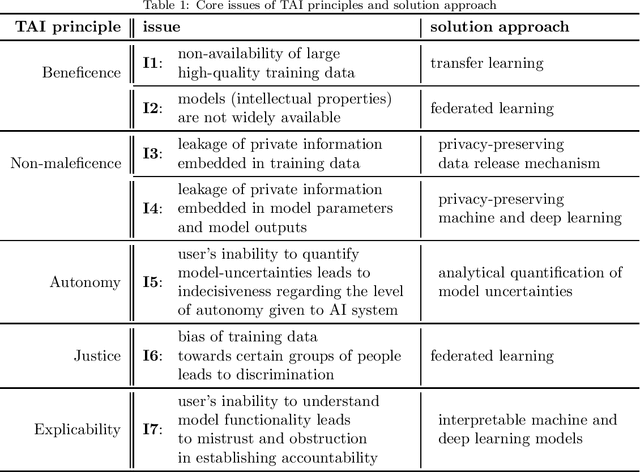
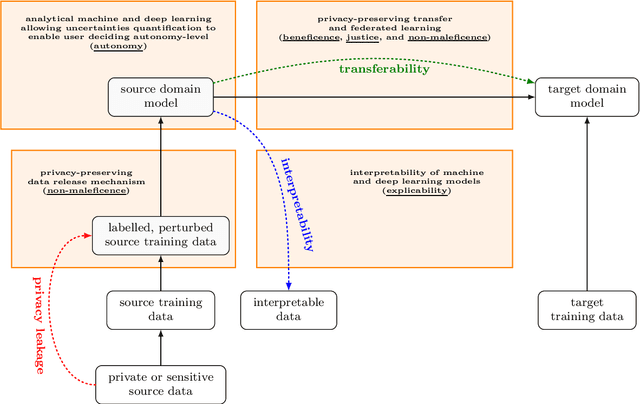
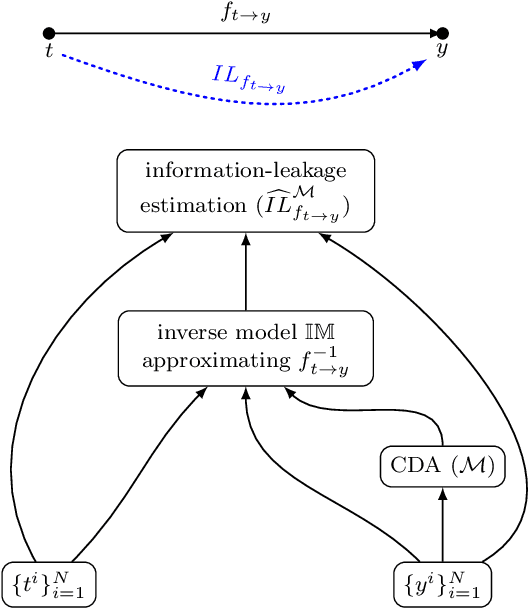
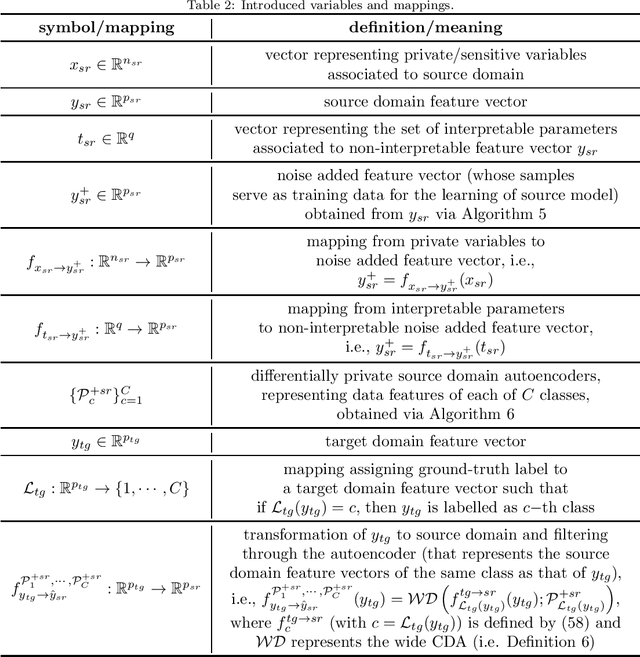
Guidelines and principles of trustworthy AI should be adhered to in practice during the development of AI systems. This work suggests a novel information theoretic trustworthy AI framework based on the hypothesis that information theory enables taking into account the ethical AI principles during the development of machine learning and deep learning models via providing a way to study and optimize the inherent tradeoffs between trustworthy AI principles. A unified approach to "privacy-preserving interpretable and transferable learning" is presented via introducing the information theoretic measures for privacy-leakage, interpretability, and transferability. A technique based on variational optimization, employing conditionally deep autoencoders, is developed for practically calculating the defined information theoretic measures for privacy-leakage, interpretability, and transferability.

Membership-Mappings for Data Representation Learning

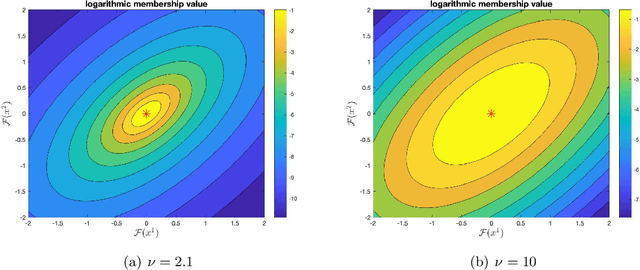
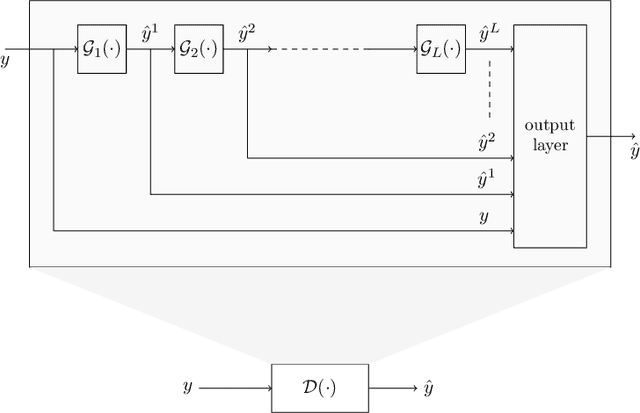
This study introduces using measure theoretic basis the notion of membership-mapping for representing data points through attribute values (motivated by fuzzy theory). A property of the membership-mapping, that can be exploited for data representation learning, is of providing an interpolation on the given data points in the data space. The study outlines an analytical approach to the variational learning of a membership-mappings based data representation model. An alternative idea of deep autoencoder, referred to as Bregman Divergence Based Conditionally Deep Autoencoder (that consists of layers such that each layer learns data representation at certain abstraction level through a membership-mappings based autoencoder), is presented. Experiments are provided to demonstrate the competitive performance of the proposed framework in classifying high-dimensional feature vectors and in rendering robustness to the classification.