Breaking Symmetries in Graph Search with Canonizing Sets

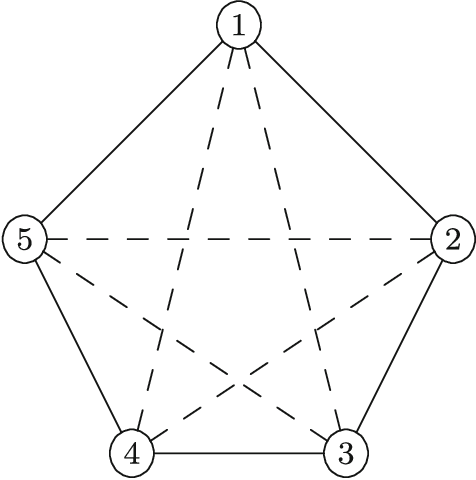
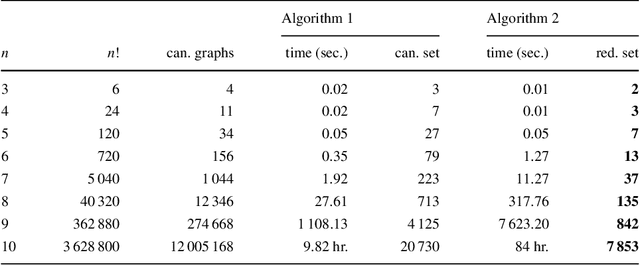
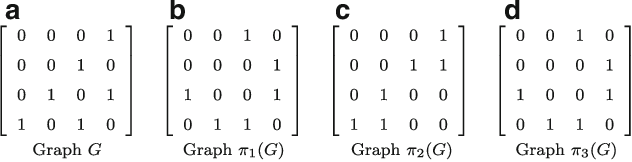
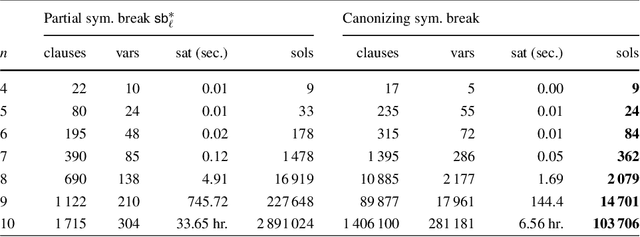
There are many complex combinatorial problems which involve searching for an undirected graph satisfying given constraints. Such problems are often highly challenging because of the large number of isomorphic representations of their solutions. This paper introduces effective and compact, complete symmetry breaking constraints for small graph search. Enumerating with these symmetry breaks generates all and only non-isomorphic solutions. For small search problems, with up to $10$ vertices, we compute instance independent symmetry breaking constraints. For small search problems with a larger number of vertices we demonstrate the computation of instance dependent constraints which are complete. We illustrate the application of complete symmetry breaking constraints to extend two known sequences from the OEIS related to graph enumeration. We also demonstrate the application of a generalization of our approach to fully-interchangeable matrix search problems.

Computing the Ramsey Number R(4,3,3) using Abstraction and Symmetry breaking

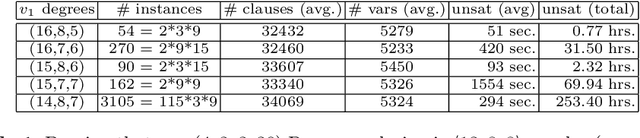
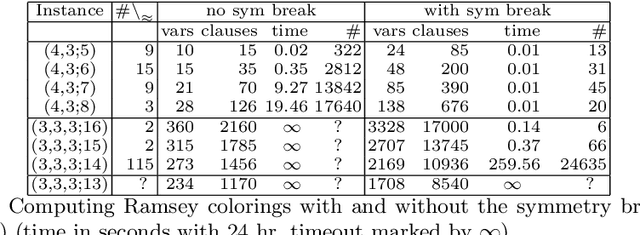
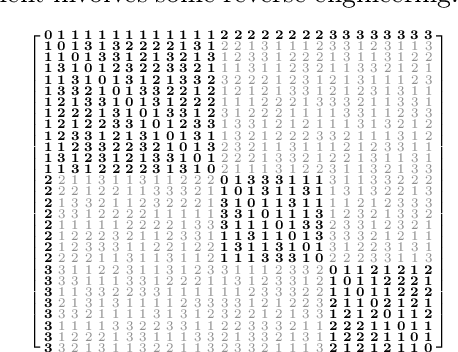
The number $R(4,3,3)$ is often presented as the unknown Ramsey number with the best chances of being found "soon". Yet, its precise value has remained unknown for almost 50 years. This paper presents a methodology based on \emph{abstraction} and \emph{symmetry breaking} that applies to solve hard graph edge-coloring problems. The utility of this methodology is demonstrated by using it to compute the value $R(4,3,3)=30$. Along the way it is required to first compute the previously unknown set ${\cal R}(3,3,3;13)$ consisting of 78{,}892 Ramsey colorings.

Solving Graph Coloring Problems with Abstraction and Symmetry

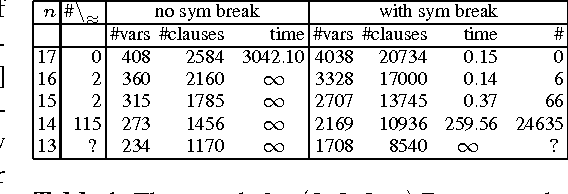
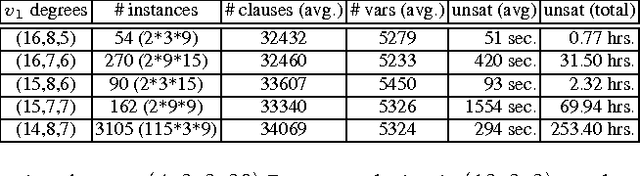
This paper introduces a general methodology, based on abstraction and symmetry, that applies to solve hard graph edge-coloring problems and demonstrates its use to provide further evidence that the Ramsey number $R(4,3,3)=30$. The number $R(4,3,3)$ is often presented as the unknown Ramsey number with the best chances of being found "soon". Yet, its precise value has remained unknown for more than 50 years. We illustrate our approach by showing that: (1) there are precisely 78{,}892 $(3,3,3;13)$ Ramsey colorings; and (2) if there exists a $(4,3,3;30)$ Ramsey coloring then it is (13,8,8) regular. Specifically each node has 13 edges in the first color, 8 in the second, and 8 in the third. We conjecture that these two results will help provide a proof that no $(4,3,3;30)$ Ramsey coloring exists implying that $R(4,3,3)=30$.