Data-driven Enhancement of the Time-domain First-order Regular Perturbation Model

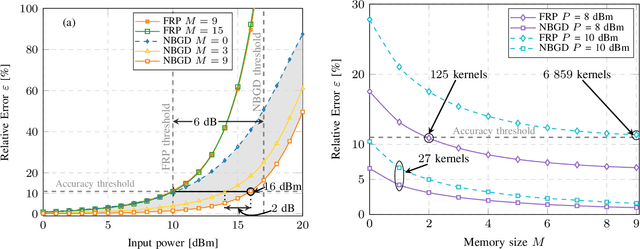
A normalized batch gradient descent optimizer is proposed to improve the first-order regular perturbation coefficients of the Manakov equation, often referred to as kernels. The optimization is based on the linear parameterization offered by the first-order regular perturbation and targets enhanced low-complexity models for the fiber channel. We demonstrate that the optimized model outperforms the analytical counterpart where the kernels are numerically evaluated via their integral form. The enhanced model provides the same accuracy with a reduced number of kernels while operating over an extended power range covering both the nonlinear and highly nonlinear regimes. A $6-7$~dB gain, depending on the metric used, is obtained with respect to the conventional first-order regular perturbation.

Discrete-Time Accuracy Analysis of the Time-Domain Regular Perturbation Model for Unamplified Links

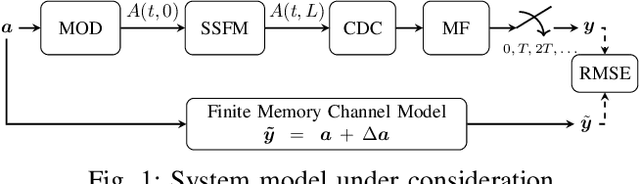
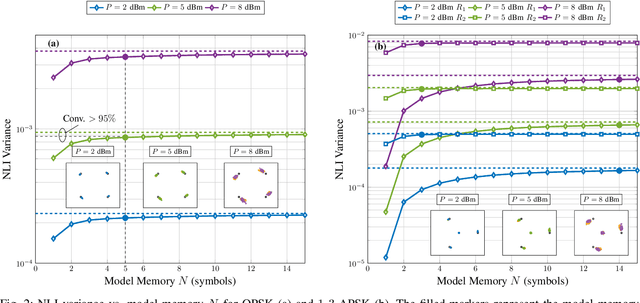
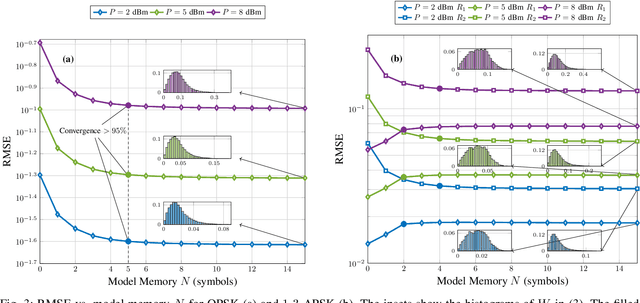
The accuracy of a discrete-time channel model based on regular perturbation is numerically studied for unamplified links. We analyse the distance between discrete nonlinear interference points and show that such distance can be used to estimate the effective channel memory.

Modeling of Nonlinear Interference Power for Dual-Polarization 4D Formats

We assess the accuracy of a recently introduced nonlinear interference model for general dual-polarization 4D formats.~ Unlike previous models for polarization-multiplexed 2D formats, an average gap from split-step Fourier simulations within 0.1 dB is demonstrated.