Causal networks and freedom of choice in Bell's theorem

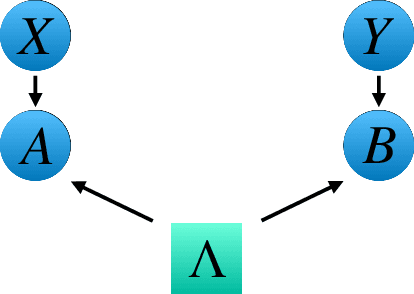
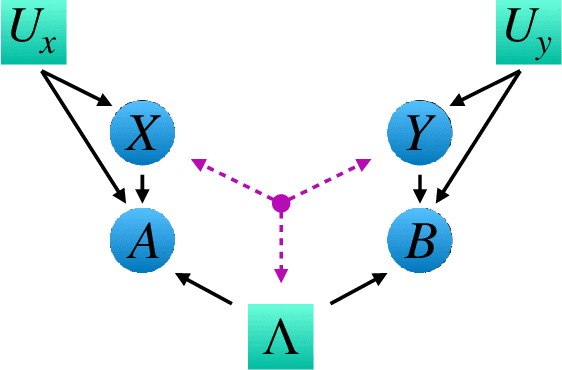
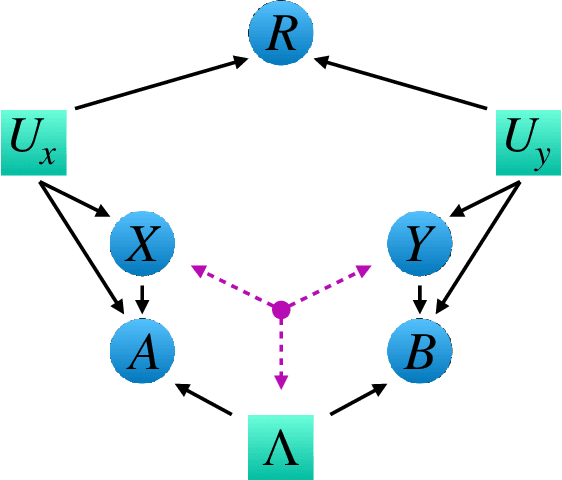
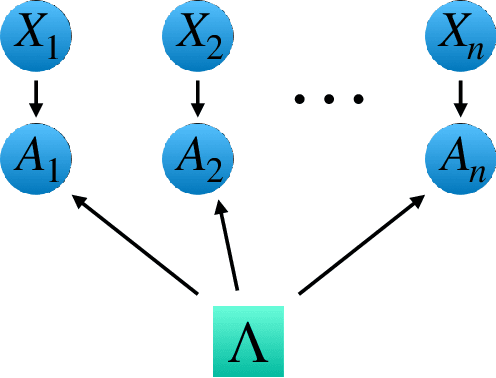
Bell's theorem is typically understood as the proof that quantum theory is incompatible with local hidden variable models. More generally, we can see the violation of a Bell inequality as witnessing the impossibility of explaining quantum correlations with classical causal models. The violation of a Bell inequality, however, does not exclude classical models where some level of measurement dependence is allowed, that is, the choice made by observers can be correlated with the source generating the systems to be measured. Here we show that the level of measurement dependence can be quantitatively upper bounded if we arrange the Bell test within a network. Furthermore, we also prove that these results can be adapted in order to derive non-linear Bell inequalities for a large class of causal networks and to identify quantumly realizable correlations which violate them.

Unveiling phase transitions with machine learning

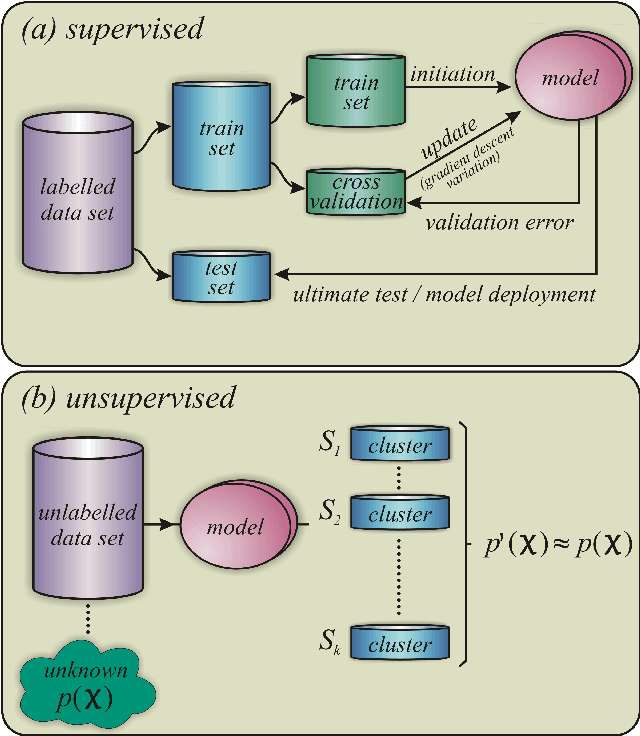
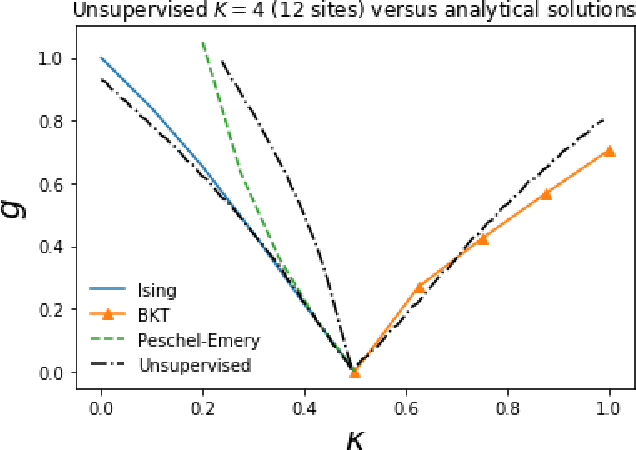
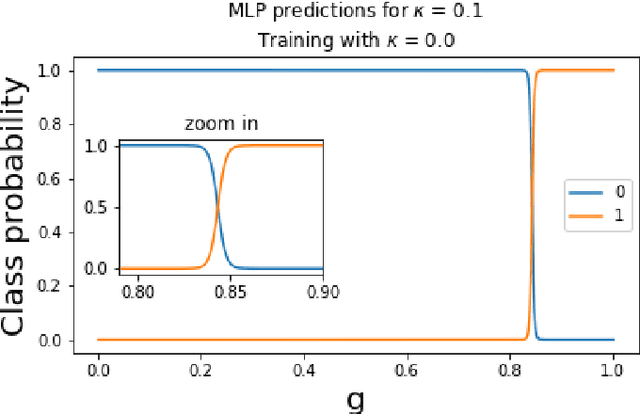
The classification of phase transitions is a central and challenging task in condensed matter physics. Typically, it relies on the identification of order parameters and the analysis of singularities in the free energy and its derivatives. Here, we propose an alternative framework to identify quantum phase transitions, employing both unsupervised and supervised machine learning techniques. Using the axial next-nearest neighbor Ising (ANNNI) model as a benchmark, we show how unsupervised learning can detect three phases (ferromagnetic, paramagnetic, and a cluster of the antiphase with the floating phase) as well as two distinct regions within the paramagnetic phase. Employing supervised learning we show that transfer learning becomes possible: a machine trained only with nearest-neighbour interactions can learn to identify a new type of phase occurring when next-nearest-neighbour interactions are introduced. All our results rely on few and low dimensional input data (up to twelve lattice sites), thus providing a computational friendly and general framework for the study of phase transitions in many-body systems.

Machine learning non-local correlations

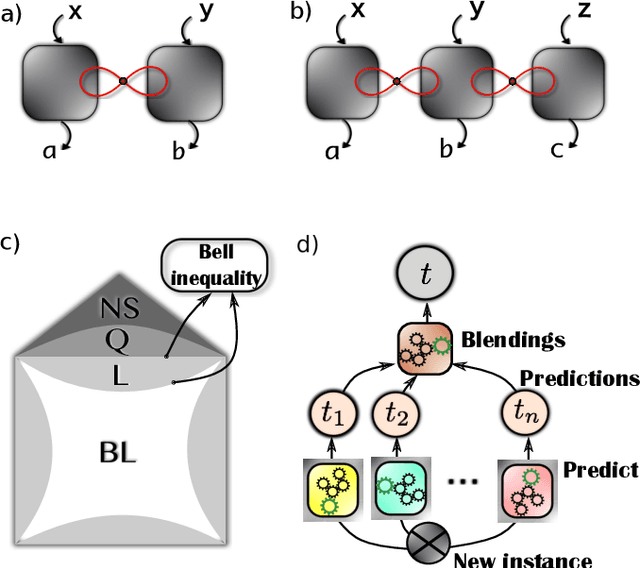
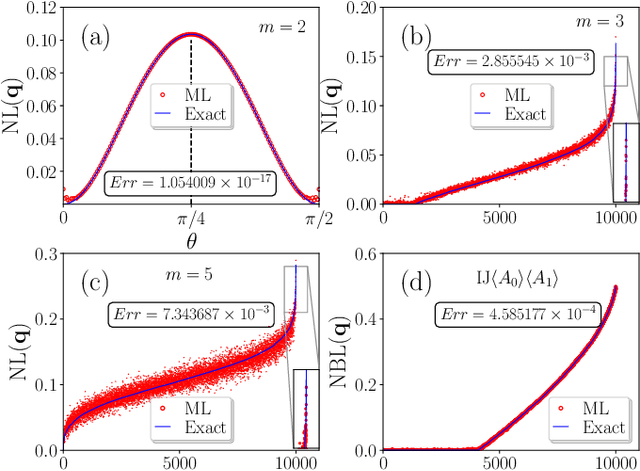
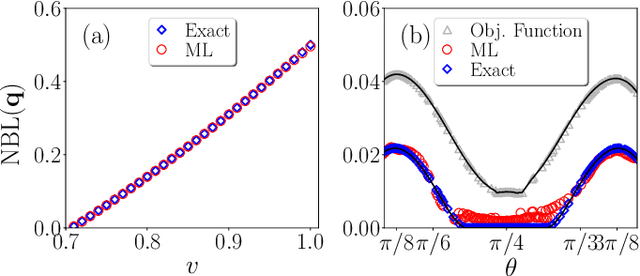
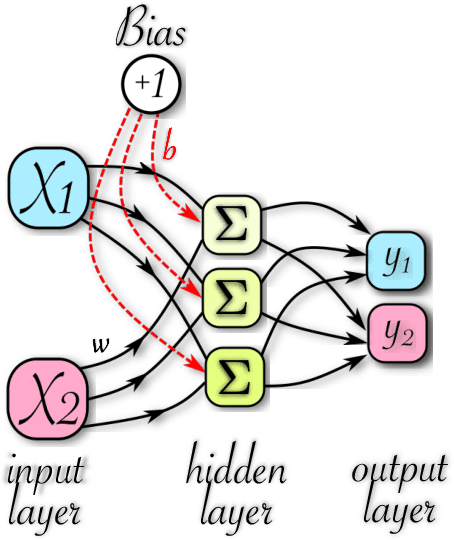
The ability to witness non-local correlations lies at the core of foundational aspects of quantum mechanics and its application in the processing of information. Commonly, this is achieved via the violation of Bell inequalities. Unfortunately, however, their systematic derivation quickly becomes unfeasible as the scenario of interest grows in complexity. To cope with that, we propose here a machine learning approach for the detection and quantification of non-locality. It consists of an ensemble of multilayer perceptrons blended with genetic algorithms achieving a high performance in a number of relevant Bell scenarios. Our results offer a novel method and a proof-of-principle for the relevance of machine learning for understanding non-locality.