Arup Mondal
BEAS: Blockchain Enabled Asynchronous & Secure Federated Machine Learning
Feb 06, 2022


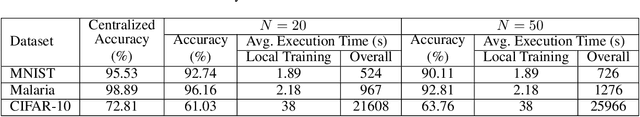
Abstract:Federated Learning (FL) enables multiple parties to distributively train a ML model without revealing their private datasets. However, it assumes trust in the centralized aggregator which stores and aggregates model updates. This makes it prone to gradient tampering and privacy leakage by a malicious aggregator. Malicious parties can also introduce backdoors into the joint model by poisoning the training data or model gradients. To address these issues, we present BEAS, the first blockchain-based framework for N-party FL that provides strict privacy guarantees of training data using gradient pruning (showing improved differential privacy compared to existing noise and clipping based techniques). Anomaly detection protocols are used to minimize the risk of data-poisoning attacks, along with gradient pruning that is further used to limit the efficacy of model-poisoning attacks. We also define a novel protocol to prevent premature convergence in heterogeneous learning environments. We perform extensive experiments on multiple datasets with promising results: BEAS successfully prevents privacy leakage from dataset reconstruction attacks, and minimizes the efficacy of poisoning attacks. Moreover, it achieves an accuracy similar to centralized frameworks, and its communication and computation overheads scale linearly with the number of participants.
Scotch: An Efficient Secure Computation Framework for Secure Aggregation
Jan 19, 2022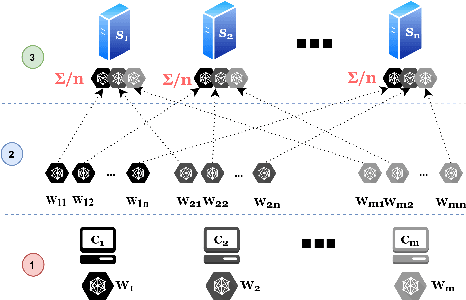


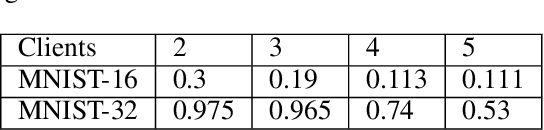
Abstract:Federated learning enables multiple data owners to jointly train a machine learning model without revealing their private datasets. However, a malicious aggregation server might use the model parameters to derive sensitive information about the training dataset used. To address such leakage, differential privacy and cryptographic techniques have been investigated in prior work, but these often result in large communication overheads or impact model performance. To mitigate this centralization of power, we propose \textsc{Scotch}, a decentralized \textit{m-party} secure-computation framework for federated aggregation that deploys MPC primitives, such as \textit{secret sharing}. Our protocol is simple, efficient, and provides strict privacy guarantees against curious aggregators or colluding data-owners with minimal communication overheads compared to other existing \textit{state-of-the-art} privacy-preserving federated learning frameworks. We evaluate our framework by performing extensive experiments on multiple datasets with promising results. \textsc{Scotch} can train the standard MLP NN with the training dataset split amongst 3 participating users and 3 aggregating servers with 96.57\% accuracy on MNIST, and 98.40\% accuracy on the Extended MNIST (digits) dataset, while providing various optimizations.
S++: A Fast and Deployable Secure-Computation Framework for Privacy-Preserving Neural Network Training
Jan 28, 2021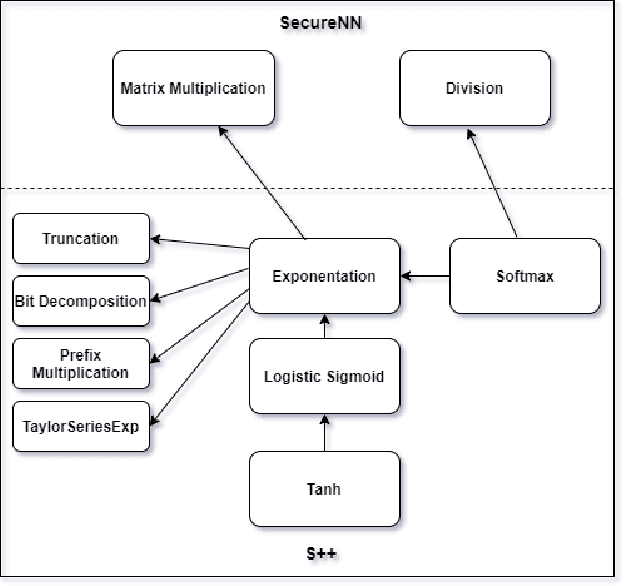
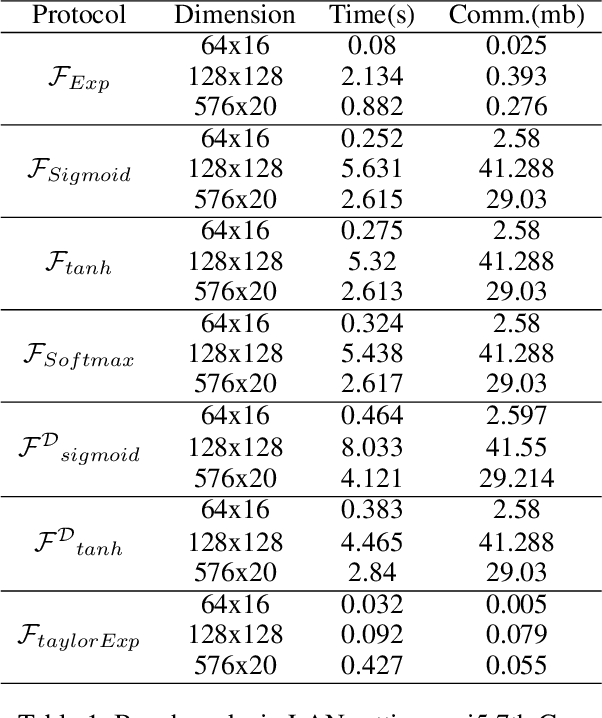
Abstract:We introduce S++, a simple, robust, and deployable framework for training a neural network (NN) using private data from multiple sources, using secret-shared secure function evaluation. In short, consider a virtual third party to whom every data-holder sends their inputs, and which computes the neural network: in our case, this virtual third party is actually a set of servers which individually learn nothing, even with a malicious (but non-colluding) adversary. Previous work in this area has been limited to just one specific activation function: ReLU, rendering the approach impractical for many use-cases. For the first time, we provide fast and verifiable protocols for all common activation functions and optimize them for running in a secret-shared manner. The ability to quickly, verifiably, and robustly compute exponentiation, softmax, sigmoid, etc., allows us to use previously written NNs without modification, vastly reducing developer effort and complexity of code. In recent times, ReLU has been found to converge much faster and be more computationally efficient as compared to non-linear functions like sigmoid or tanh. However, we argue that it would be remiss not to extend the mechanism to non-linear functions such as the logistic sigmoid, tanh, and softmax that are fundamental due to their ability to express outputs as probabilities and their universal approximation property. Their contribution in RNNs and a few recent advancements also makes them more relevant.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge