Applying Multi-Fidelity Bayesian Optimization in Chemistry: Open Challenges and Major Considerations

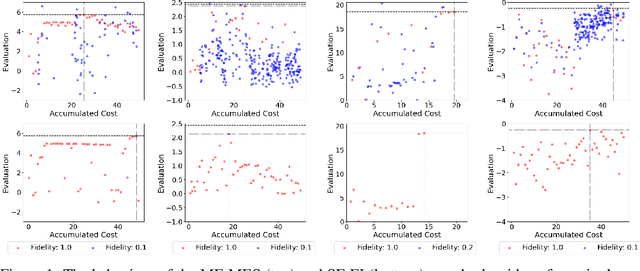
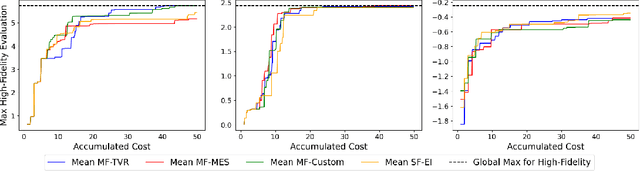
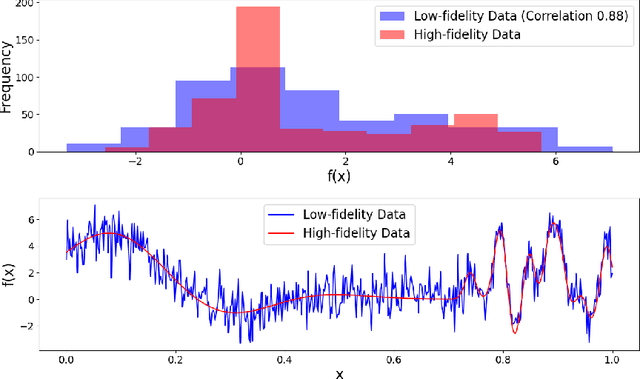
Multi fidelity Bayesian optimization (MFBO) leverages experimental and or computational data of varying quality and resource cost to optimize towards desired maxima cost effectively. This approach is particularly attractive for chemical discovery due to MFBO's ability to integrate diverse data sources. Here, we investigate the application of MFBO to accelerate the identification of promising molecules or materials. We specifically analyze the conditions under which lower fidelity data can enhance performance compared to single-fidelity problem formulations. We address two key challenges, selecting the optimal acquisition function, understanding the impact of cost, and data fidelity correlation. We then discuss how to assess the effectiveness of MFBO for chemical discovery.

Distributional constrained reinforcement learning for supply chain optimization

This work studies reinforcement learning (RL) in the context of multi-period supply chains subject to constraints, e.g., on production and inventory. We introduce Distributional Constrained Policy Optimization (DCPO), a novel approach for reliable constraint satisfaction in RL. Our approach is based on Constrained Policy Optimization (CPO), which is subject to approximation errors that in practice lead it to converge to infeasible policies. We address this issue by incorporating aspects of distributional RL into DCPO. Specifically, we represent the return and cost value functions using neural networks that output discrete distributions, and we reshape costs based on the associated confidence. Using a supply chain case study, we show that DCPO improves the rate at which the RL policy converges and ensures reliable constraint satisfaction by the end of training. The proposed method also improves predictability, greatly reducing the variance of returns between runs, respectively; this result is significant in the context of policy gradient methods, which intrinsically introduce significant variance during training.