A Differentiable Loss Function for Learning Heuristics in A*

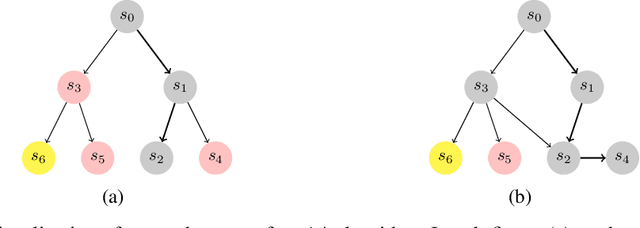
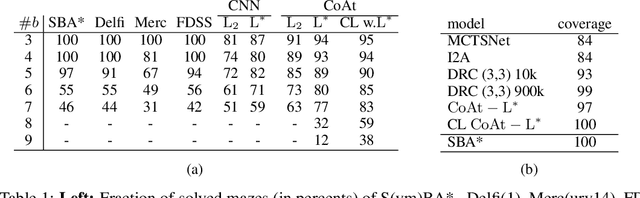
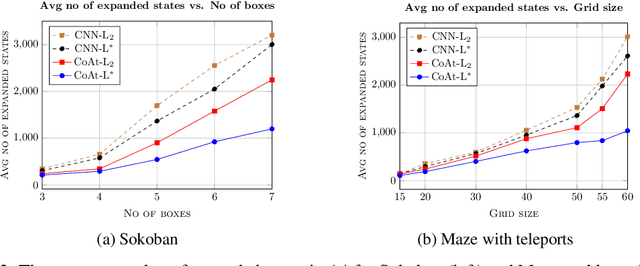
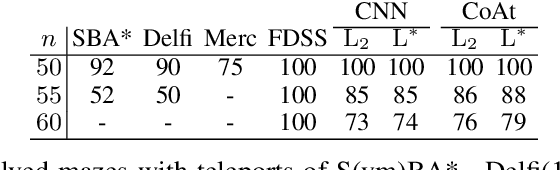
Optimization of heuristic functions for the A* algorithm, realized by deep neural networks, is usually done by minimizing square root loss of estimate of the cost to goal values. This paper argues that this does not necessarily lead to a faster search of A* algorithm since its execution relies on relative values instead of absolute ones. As a mitigation, we propose a L* loss, which upper-bounds the number of excessively expanded states inside the A* search. The L* loss, when used in the optimization of state-of-the-art deep neural networks for automated planning in maze domains like Sokoban and maze with teleports, significantly improves the fraction of solved problems, the quality of founded plans, and reduces the number of expanded states to approximately 50%

Heuristic Search Planning with Deep Neural Networks using Imitation, Attention and Curriculum Learning

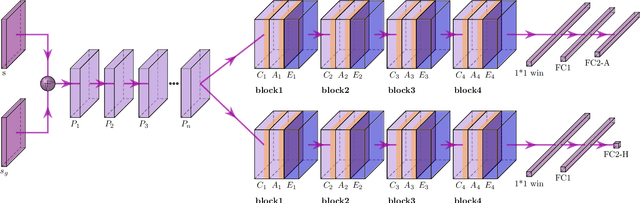
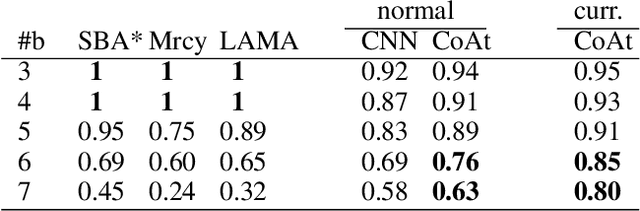
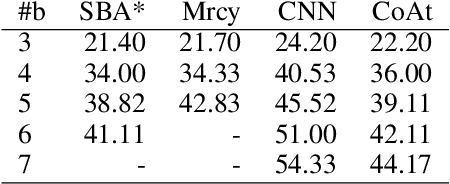
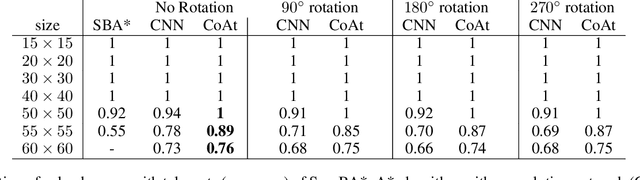
Learning a well-informed heuristic function for hard task planning domains is an elusive problem. Although there are known neural network architectures to represent such heuristic knowledge, it is not obvious what concrete information is learned and whether techniques aimed at understanding the structure help in improving the quality of the heuristics. This paper presents a network model to learn a heuristic capable of relating distant parts of the state space via optimal plan imitation using the attention mechanism, which drastically improves the learning of a good heuristic function. To counter the limitation of the method in the creation of problems of increasing difficulty, we demonstrate the use of curriculum learning, where newly solved problem instances are added to the training set, which, in turn, helps to solve problems of higher complexities and far exceeds the performances of all existing baselines including classical planning heuristics. We demonstrate its effectiveness for grid-type PDDL domains.