An MILP Approach for Real-time Optimal Controller Synthesis with Metric Temporal Logic Specifications

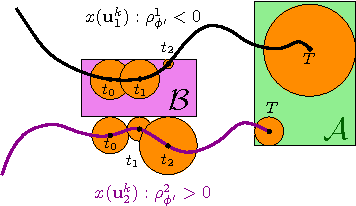
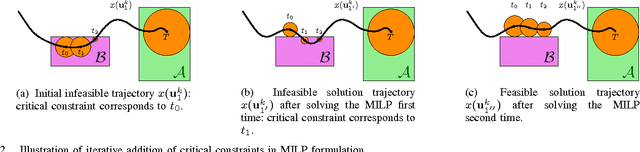
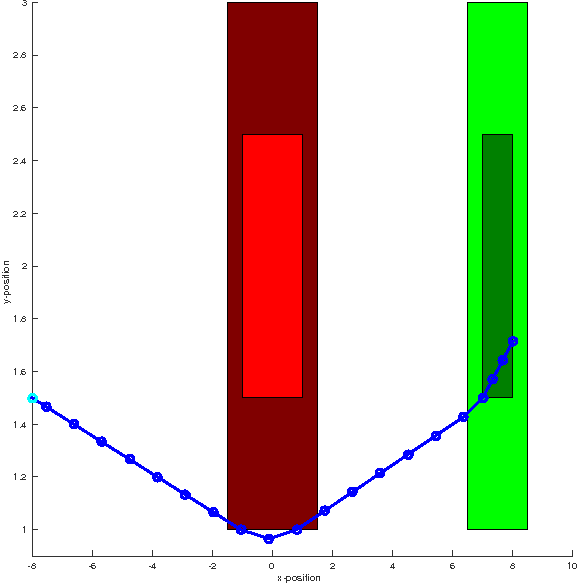
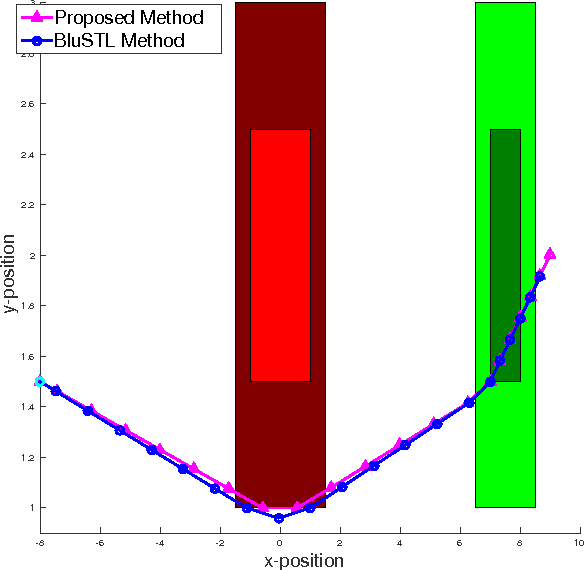
The fundamental idea of this work is to synthesize reactive controllers such that closed-loop execution trajectories of the system satisfy desired specifications that ensure correct system behaviors, while optimizing a desired performance criteria. In our approach, the correctness of a system's behavior can be defined according to the system's relation to the environment, for example, the output trajectories of the system terminate in a goal set without entering an unsafe set. Using Metric Temporal Logic (MTL) specifications we can further capture complex system behaviors and timing requirements, such as the output trajectories must pass through a number of way-points within a certain time frame before terminating in the goal set. Given a Mixed Logical Dynamical (MLD) system and system specifications in terms of MTL formula or simpler reach-avoid specifications, our goal is to find a closed-loop trajectory that satisfies the specifications, in non-deterministic environments. Using an MILP framework we search over the space of input signals to obtain such valid trajectories of the system, by adding constraints to satisfy the MTL formula only when necessary, to avoid the exponential complexity of solving MILP problems. We also present experimental results for planning a path for a mobile robot through a dynamically changing environment with a desired task specification.

Tracking Tetrahymena Pyriformis Cells using Decision Trees

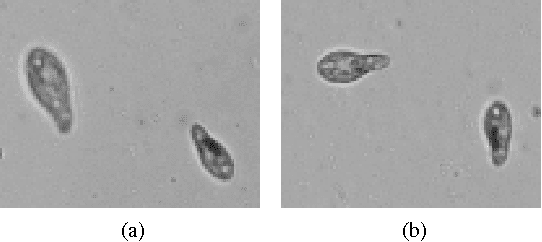
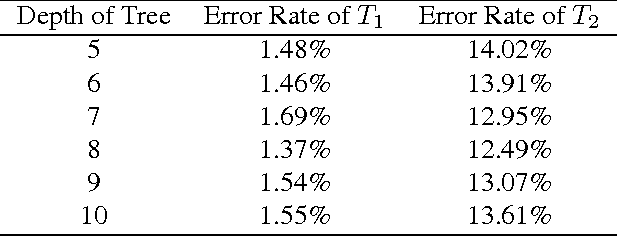
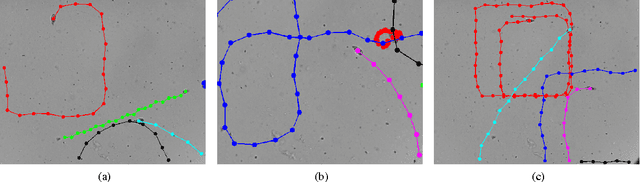
Matching cells over time has long been the most difficult step in cell tracking. In this paper, we approach this problem by recasting it as a classification problem. We construct a feature set for each cell, and compute a feature difference vector between a cell in the current frame and a cell in a previous frame. Then we determine whether the two cells represent the same cell over time by training decision trees as our binary classifiers. With the output of decision trees, we are able to formulate an assignment problem for our cell association task and solve it using a modified version of the Hungarian algorithm.