Relative Pose Estimation for Multi-Camera Systems from Affine Correspondences
Paper and Code
Jul 21, 2020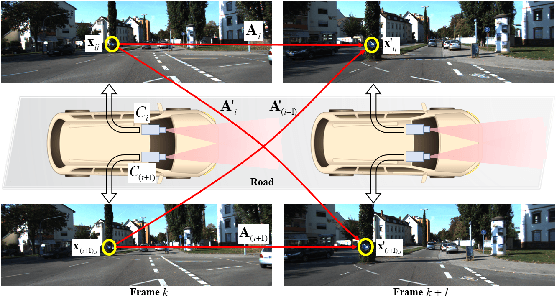
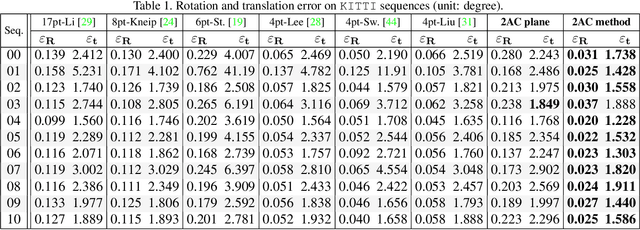


We propose four novel solvers for estimating the relative pose of a multi-camera system from affine correspondences (ACs). A new constraint is derived interpreting the relationship of ACs and the generalized camera model. Using the constraint, it is shown that a minimum of two ACs are enough for recovering the 6DOF relative pose, i.e., 3D rotation and translation, of the system. Considering planar camera motion, we propose a minimal solution using a single AC and a solver with two ACs to overcome the degenerate case. Also, we propose a minimal solution using two ACs with known gravity vector, e.g., from an IMU. Since the proposed methods require significantly fewer correspondences than state-of-the-art algorithms, they can be efficiently used within RANSAC for outlier removal and initial motion estimation. The solvers are tested both on synthetic data and on real-world scenes from the KITTI benchmark. It is shown that the accuracy of the estimated poses is superior to the state-of-the-art techniques.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge