Quantized Fisher Discriminant Analysis
Paper and Code
Sep 06, 2019
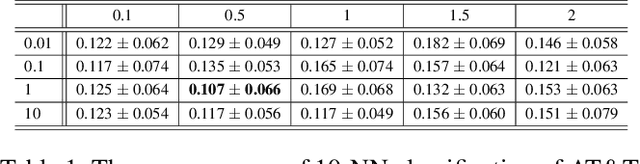
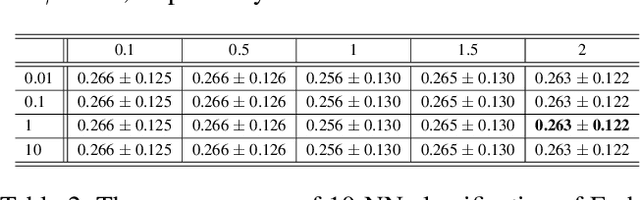
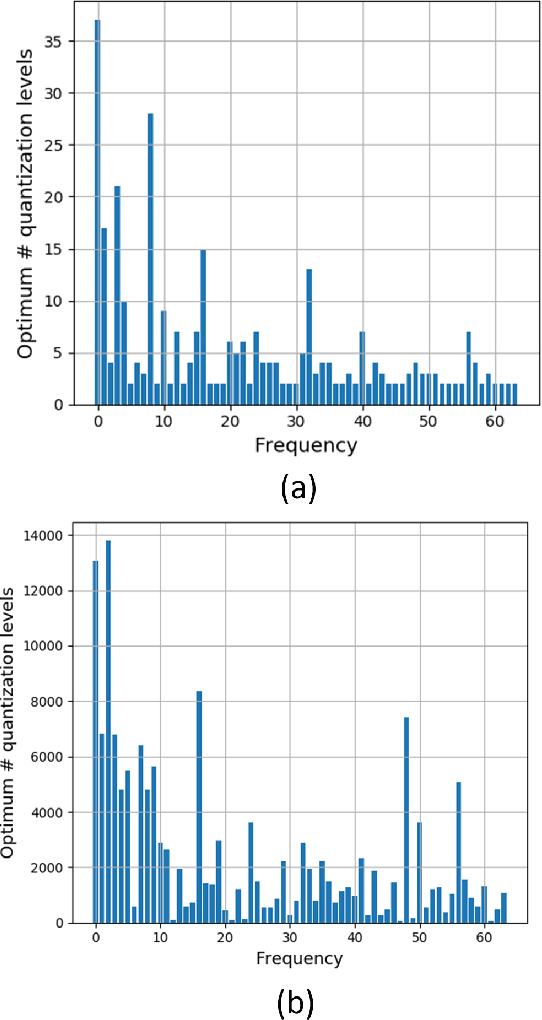
This paper proposes a new subspace learning method, named Quantized Fisher Discriminant Analysis (QFDA), which makes use of both machine learning and information theory. There is a lack of literature for combination of machine learning and information theory and this paper tries to tackle this gap. QFDA finds a subspace which discriminates the uniformly quantized images in the Discrete Cosine Transform (DCT) domain at least as well as discrimination of non-quantized images by Fisher Discriminant Analysis (FDA) while the images have been compressed. This helps the user to throw away the original images and keep the compressed images instead without noticeable loss of classification accuracy. We propose a cost function whose minimization can be interpreted as rate-distortion optimization in information theory. We also propose quantized Fisherfaces for facial analysis in QFDA. Our experiments on AT&T face dataset and Fashion MNIST dataset show the effectiveness of this subspace learning method.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge