Predicting with Confidence on Unseen Distributions
Paper and Code
Jul 07, 2021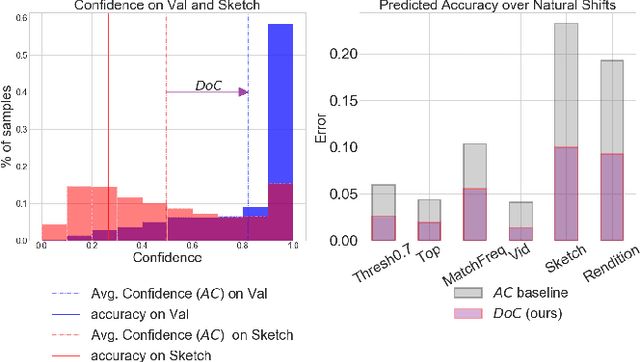
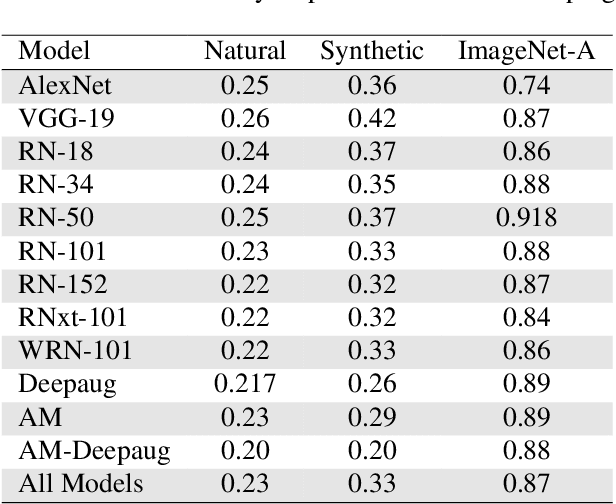
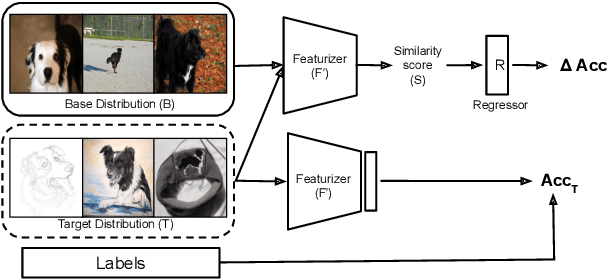
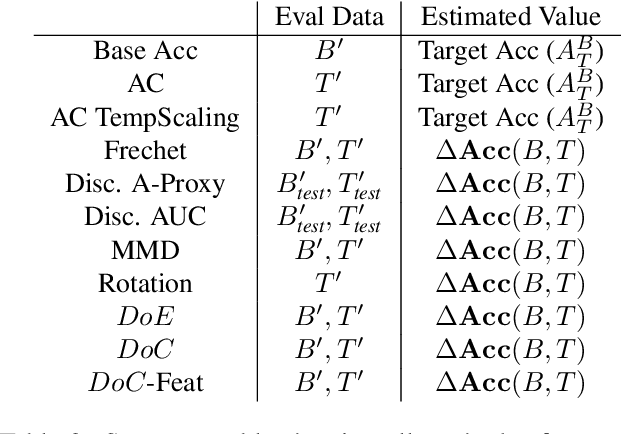
Recent work has shown that the performance of machine learning models can vary substantially when models are evaluated on data drawn from a distribution that is close to but different from the training distribution. As a result, predicting model performance on unseen distributions is an important challenge. Our work connects techniques from domain adaptation and predictive uncertainty literature, and allows us to predict model accuracy on challenging unseen distributions without access to labeled data. In the context of distribution shift, distributional distances are often used to adapt models and improve their performance on new domains, however accuracy estimation, or other forms of predictive uncertainty, are often neglected in these investigations. Through investigating a wide range of established distributional distances, such as Frechet distance or Maximum Mean Discrepancy, we determine that they fail to induce reliable estimates of performance under distribution shift. On the other hand, we find that the difference of confidences (DoC) of a classifier's predictions successfully estimates the classifier's performance change over a variety of shifts. We specifically investigate the distinction between synthetic and natural distribution shifts and observe that despite its simplicity DoC consistently outperforms other quantifications of distributional difference. $DoC$ reduces predictive error by almost half ($46\%$) on several realistic and challenging distribution shifts, e.g., on the ImageNet-Vid-Robust and ImageNet-Rendition datasets.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge