PAC Model Checking of Black-Box Continuous-Time Dynamical Systems

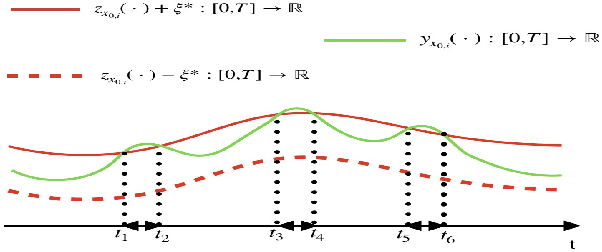
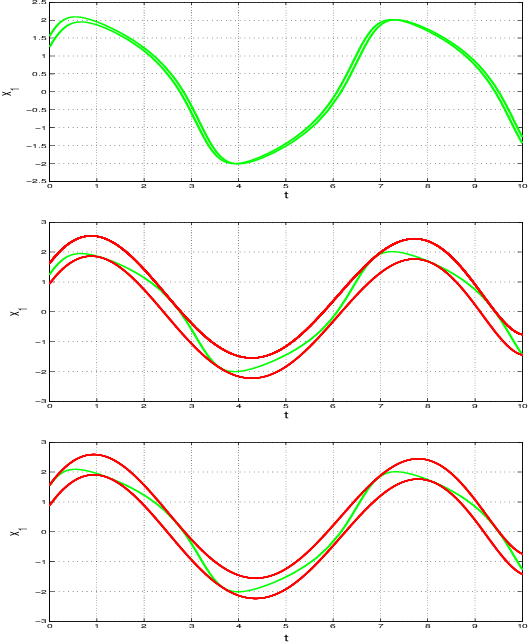
In this paper we present a novel model checking approach to finite-time safety verification of black-box continuous-time dynamical systems within the framework of probably approximately correct (PAC) learning. The black-box dynamical systems are the ones, for which no model is given but whose states changing continuously through time within a finite time interval can be observed at some discrete time instants for a given input. The new model checking approach is termed as PAC model checking due to incorporation of learned models with correctness guarantees expressed using the terms error probability and confidence. Based on the error probability and confidence level, our approach provides statistically formal guarantees that the time-evolving trajectories of the black-box dynamical system over finite time horizons fall within the range of the learned model plus a bounded interval, contributing to insights on the reachability of the black-box system and thus on the satisfiability of its safety requirements. The learned model together with the bounded interval is obtained by scenario optimization, which boils down to a linear programming problem. Three examples demonstrate the performance of our approach.