Nonlinear SVD with Asymmetric Kernels: feature learning and asymmetric Nyström method

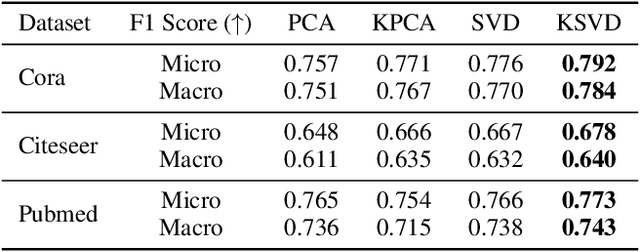
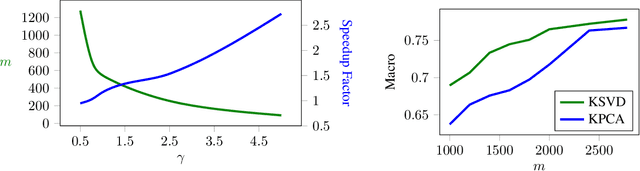
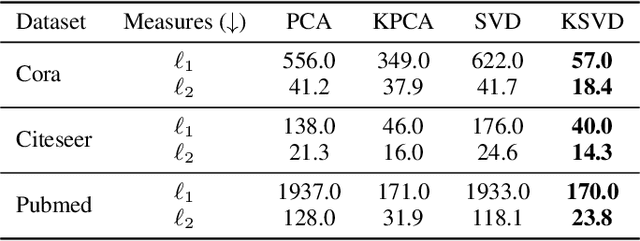
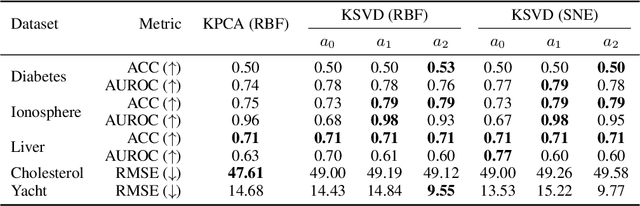
Asymmetric data naturally exist in real life, such as directed graphs. Different from the common kernel methods requiring Mercer kernels, this paper tackles the asymmetric kernel-based learning problem. We describe a nonlinear extension of the matrix Singular Value Decomposition through asymmetric kernels, namely KSVD. First, we construct two nonlinear feature mappings w.r.t. rows and columns of the given data matrix. The proposed optimization problem maximizes the variance of each mapping projected onto the subspace spanned by the other, subject to a mutual orthogonality constraint. Through Lagrangian duality, we show that it can be solved by the left and right singular vectors in the feature space induced by the asymmetric kernel. Moreover, we start from the integral equations with a pair of adjoint eigenfunctions corresponding to the singular vectors on an asymmetrical kernel, and extend the Nystr\"om method to asymmetric cases through the finite sample approximation, which can be applied to speedup the training in KSVD. Experiments show that asymmetric KSVD learns features outperforming Mercer-kernel based methods that resort to symmetrization, and also verify the effectiveness of the asymmetric Nystr\"om method.