New explicit thresholding/shrinkage formulas for one class of regularization problems with overlapping group sparsity and their applications
Paper and Code
May 09, 2014


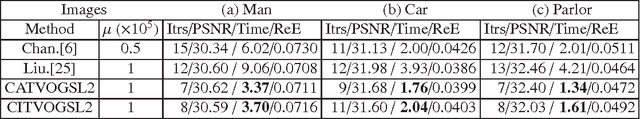
The least-square regression problems or inverse problems have been widely studied in many fields such as compressive sensing, signal processing, and image processing. To solve this kind of ill-posed problems, a regularization term (i.e., regularizer) should be introduced, under the assumption that the solutions have some specific properties, such as sparsity and group sparsity. Widely used regularizers include the $\ell_1$ norm, total variation (TV) semi-norm, and so on. Recently, a new regularization term with overlapping group sparsity has been considered. Majorization minimization iteration method or variable duplication methods are often applied to solve them. However, there have been no direct methods for solve the relevant problems because of the difficulty of overlapping. In this paper, we proposed new explicit shrinkage formulas for one class of these relevant problems, whose regularization terms have translation invariant overlapping groups. Moreover, we apply our results in TV deblurring and denoising with overlapping group sparsity. We use alternating direction method of multipliers to iterate solve it. Numerical results also verify the validity and effectiveness of our new explicit shrinkage formulas.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge