HYPER^2: Hyperbolic Poincare Embedding for Hyper-Relational Link Prediction
Paper and Code
Apr 20, 2021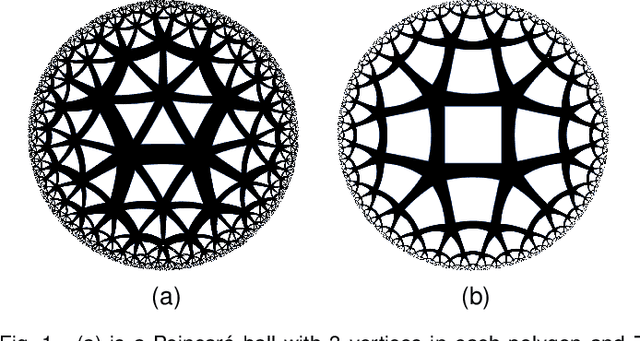

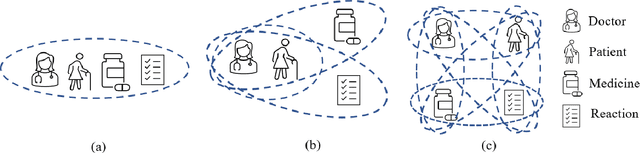
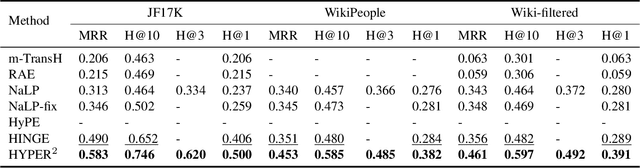
Link Prediction, addressing the issue of completing KGs with missing facts, has been broadly studied. However, less light is shed on the ubiquitous hyper-relational KGs. Most existing hyper-relational KG embedding models still tear an n-ary fact into smaller tuples, neglecting the indecomposability of some n-ary facts. While other frameworks work for certain arity facts only or ignore the significance of primary triple. In this paper, we represent an n-ary fact as a whole, simultaneously keeping the integrity of n-ary fact and maintaining the vital role that the primary triple plays. In addition, we generalize hyperbolic Poincar\'e embedding from binary to arbitrary arity data, which has not been studied yet. To tackle the weak expressiveness and high complexity issue, we propose HYPER^2 which is qualified for capturing the interaction between entities within and beyond triple through information aggregation on the tangent space. Extensive experiments demonstrate HYPER^2 achieves superior performance to its translational and deep analogues, improving SOTA by up to 34.5\% with relatively few dimensions. Moreover, we study the side effect of literals and we theoretically and experimentally compare the computational complexity of HYPER^2 against several best performing baselines, HYPER^2 is 49-61 times quicker than its counterparts.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge