Grokking Modular Polynomials
Paper and Code
Jun 05, 2024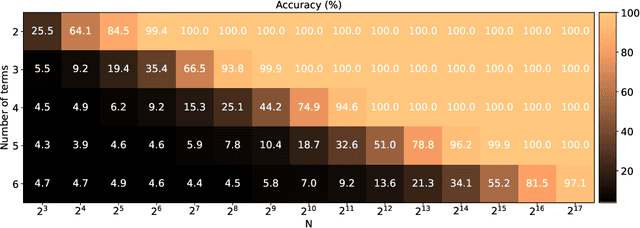
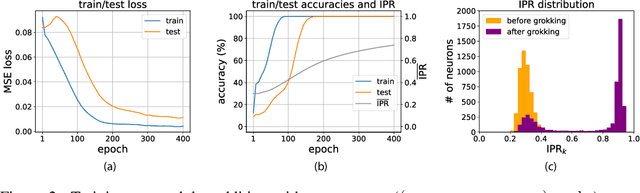

Neural networks readily learn a subset of the modular arithmetic tasks, while failing to generalize on the rest. This limitation remains unmoved by the choice of architecture and training strategies. On the other hand, an analytical solution for the weights of Multi-layer Perceptron (MLP) networks that generalize on the modular addition task is known in the literature. In this work, we (i) extend the class of analytical solutions to include modular multiplication as well as modular addition with many terms. Additionally, we show that real networks trained on these datasets learn similar solutions upon generalization (grokking). (ii) We combine these "expert" solutions to construct networks that generalize on arbitrary modular polynomials. (iii) We hypothesize a classification of modular polynomials into learnable and non-learnable via neural networks training; and provide experimental evidence supporting our claims.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge