Efficient debiasing with contrastive weight pruning

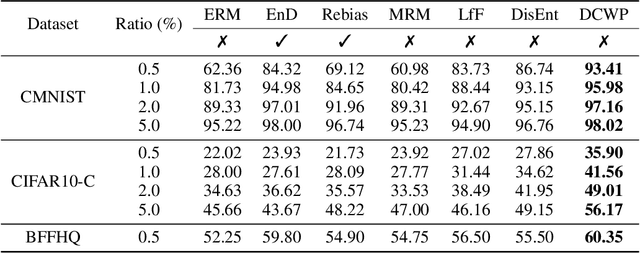
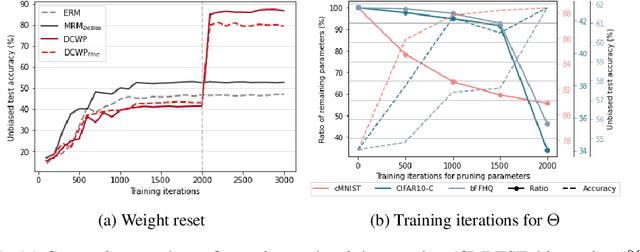
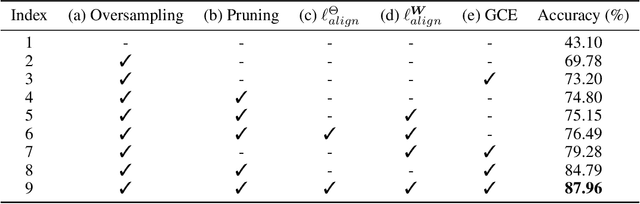
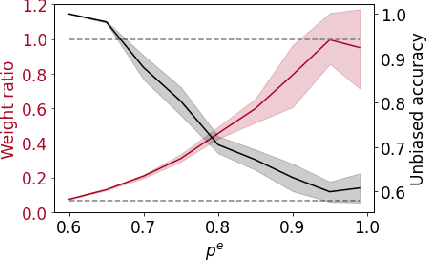
Neural networks are often biased to spuriously correlated features that provide misleading statistical evidence that does not generalize. This raises a fundamental question: "Does an optimal unbiased functional subnetwork exist in a severely biased network? If so, how to extract such subnetwork?" While few studies have revealed the existence of such optimal subnetworks with the guidance of ground-truth unbiased samples, the way to discover the optimal subnetworks with biased training dataset is still unexplored in practice. To address this, here we first present our theoretical insight that alerts potential limitations of existing algorithms in exploring unbiased subnetworks in the presence of strong spurious correlations. We then further elucidate the importance of bias-conflicting samples on structure learning. Motivated by these observations, we propose a Debiased Contrastive Weight Pruning (DCWP) algorithm, which probes unbiased subnetworks without expensive group annotations. Experimental results demonstrate that our approach significantly outperforms state-of-the-art debiasing methods despite its considerable reduction in the number of parameters.