Disentangling Dynamics and Content for Control and Planning
Paper and Code
Nov 24, 2017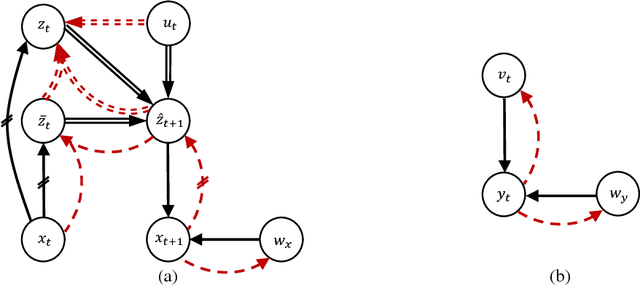
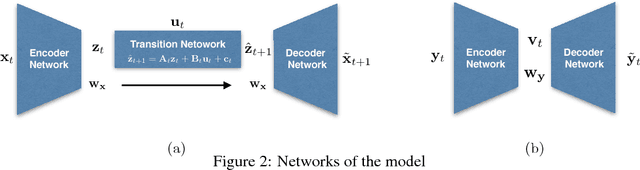
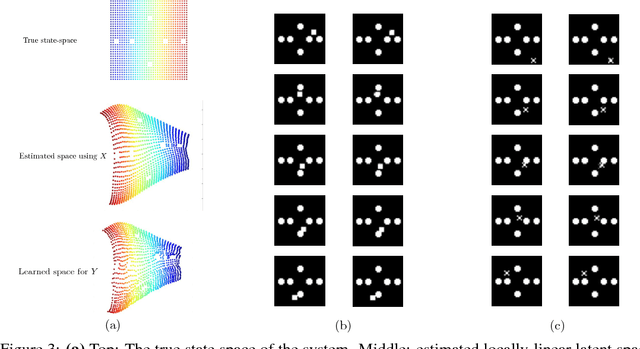
In this paper, We study the problem of learning a controllable representation for high-dimensional observations of dynamical systems. Specifically, we consider a situation where there are multiple sets of observations of dynamical systems with identical underlying dynamics. Only one of these sets has information about the effect of actions on the observation and the rest are just some random observations of the system. Our goal is to utilize the information in that one set and find a representation for the other sets that can be used for planning and ling-term prediction.
* 5
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge