CRISP: Curriculum based Sequential Neural Decoders for Polar Code Family
Paper and Code
Oct 01, 2022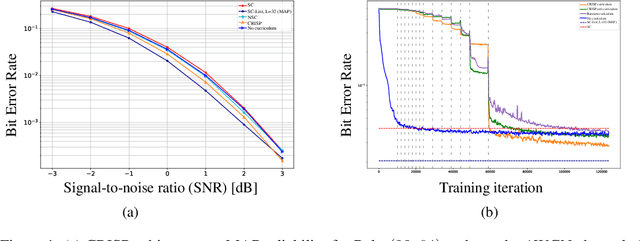
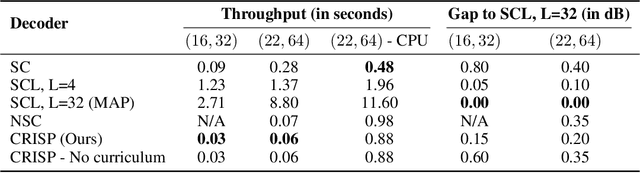
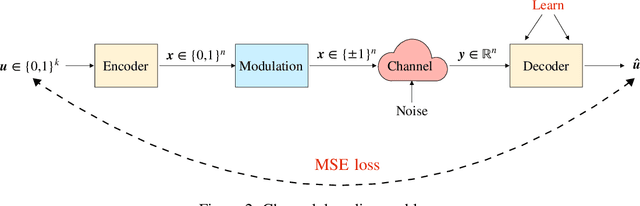
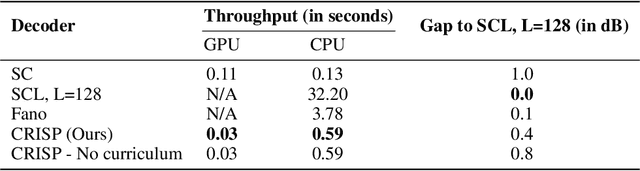
Polar codes are widely used state-of-the-art codes for reliable communication that have recently been included in the 5th generation wireless standards (5G). However, there remains room for the design of polar decoders that are both efficient and reliable in the short blocklength regime. Motivated by recent successes of data-driven channel decoders, we introduce a novel $\textbf{C}$ur$\textbf{RI}$culum based $\textbf{S}$equential neural decoder for $\textbf{P}$olar codes (CRISP). We design a principled curriculum, guided by information-theoretic insights, to train CRISP and show that it outperforms the successive-cancellation (SC) decoder and attains near-optimal reliability performance on the Polar(16,32) and Polar(22, 64) codes. The choice of the proposed curriculum is critical in achieving the accuracy gains of CRISP, as we show by comparing against other curricula. More notably, CRISP can be readily extended to Polarization-Adjusted-Convolutional (PAC) codes, where existing SC decoders are significantly less reliable. To the best of our knowledge, CRISP constructs the first data-driven decoder for PAC codes and attains near-optimal performance on the PAC(16, 32) code.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge