Consistency constraints for overlapping data clustering
Paper and Code
Aug 15, 2016
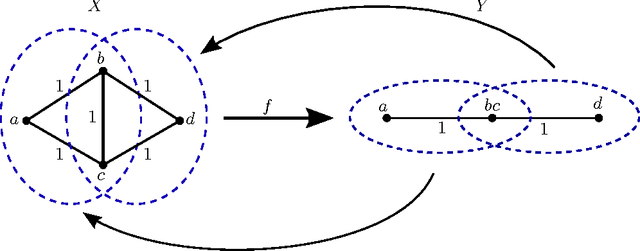
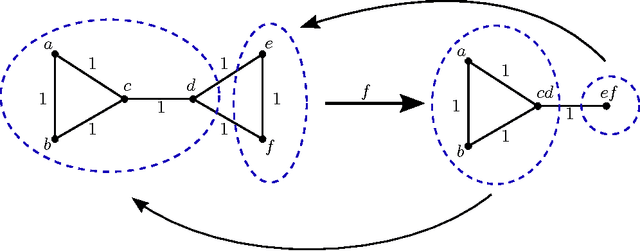
We examine overlapping clustering schemes with functorial constraints, in the spirit of Carlsson--Memoli. This avoids issues arising from the chaining required by partition-based methods. Our principal result shows that any clustering functor is naturally constrained to refine single-linkage clusters and be refined by maximal-linkage clusters. We work in the context of metric spaces with non-expansive maps, which is appropriate for modeling data processing which does not increase information content.
* 12 pages
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge