Configuring Intelligent Reflecting Surface with Performance Guarantees: Optimal Beamforming
Paper and Code
Dec 04, 2021

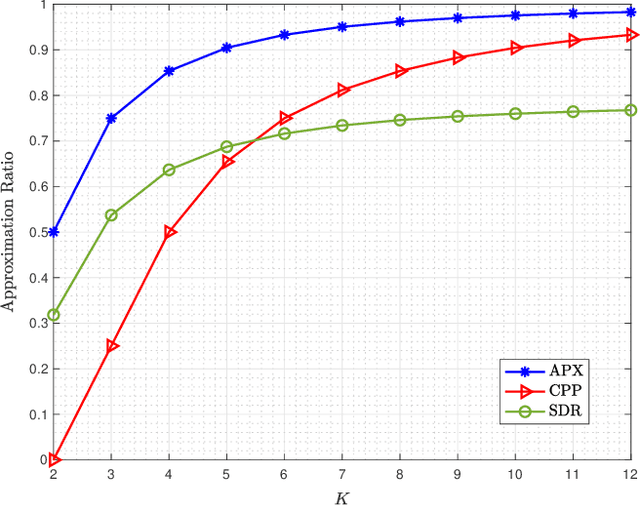
This work proposes linear time strategies to optimally configure the phase shifts for the reflective elements of an intelligent reflecting surface (IRS). Specifically, we show that the binary phase beamforming can be optimally solved in linear time to maximize the received signal-to-noise ratio (SNR). For the general K-ary phase beamforming, we develop a linear time approximation algorithm that guarantees performance within a constant fraction (1+\cos(\pi/K))/2 of the global optimum, e.g., it can attain over 85% of the optimal performance for the quadrature beamforming with K=4. According to the numerical results, the proposed approximation algorithm for discrete IRS beamforming outperforms the existing algorithms significantly in boosting the received SNR.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge