Compressed sensing radar detectors under the row-orthogonal design model: a statistical mechanics perspective
Paper and Code
Oct 03, 2022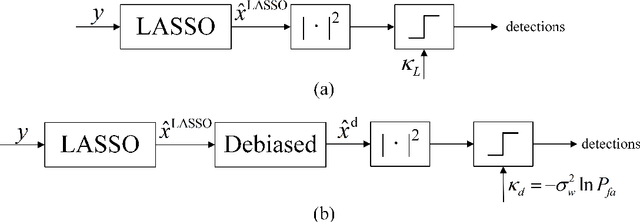

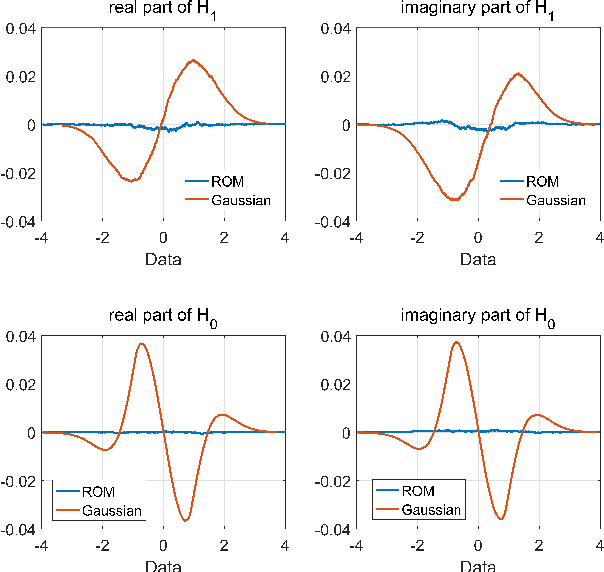
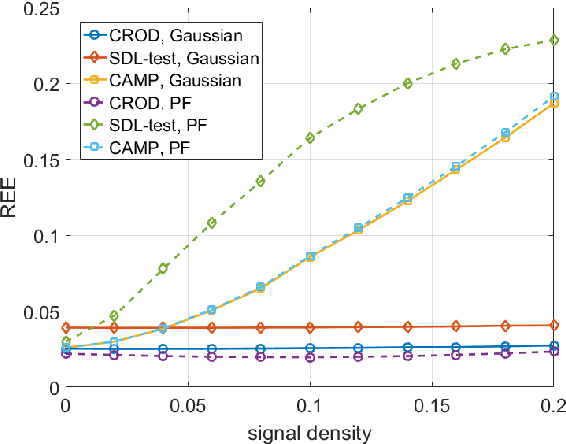
Compressed sensing (CS) model of complex-valued data can represent the signal recovery process of a large amount types of radar systems, especially when the measurement matrix is row-orthogonal. Based on debiased least absolute shrinkage and selection operator (LASSO), detection problem under Gaussian random design model, i.e. the elements of measurement matrix are drawn from Gaussian distribution, is studied by literature. However, we find that these approaches are not suitable for row-orthogonal measurement matrices, which are of more practical relevance. In view of statistical mechanics approaches, we provide derivations of more accurate test statistics and thresholds (or p-values) under the row-orthogonal design model, and theoretically analyze the detection performance of the present detector. Such detector can analytically provide the threshold according to given false alarm rate, which is not possible with the conventional CS detector, and the detection performance is proved to be better than that of the traditional LASSO detector. Comparing with other debiased LASSO based detectors, simulation results indicate that the proposed approach can achieve more accurate probability of false alarm when the measurement matrix is row-orthogonal, leading to better detection performance under Neyman-Pearson principle.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge