Discovering highly efficient low-weight quantum error-correcting codes with reinforcement learning

The realization of scalable fault-tolerant quantum computing is expected to hinge on quantum error-correcting codes. In the quest for more efficient quantum fault tolerance, a critical code parameter is the weight of measurements that extract information about errors to enable error correction: as higher measurement weights require higher implementation costs and introduce more errors, it is important in code design to optimize measurement weight. This underlies the surging interest in quantum low-density parity-check (qLDPC) codes, the study of which has primarily focused on the asymptotic (large-code-limit) properties. In this work, we introduce a versatile and computationally efficient approach to stabilizer code weight reduction based on reinforcement learning (RL), which produces new low-weight codes that substantially outperform the state of the art in practically relevant parameter regimes, extending significantly beyond previously accessible small distances. For example, our approach demonstrates savings in physical qubit overhead compared to existing results by 1 to 2 orders of magnitude for weight 6 codes and brings the overhead into a feasible range for near-future experiments. We also investigate the interplay between code parameters using our RL framework, offering new insights into the potential efficiency and power of practically viable coding strategies. Overall, our results demonstrate how RL can effectively advance the crucial yet challenging problem of quantum code discovery and thereby facilitate a faster path to the practical implementation of fault-tolerant quantum technologies.

Quantum Earth Mover's Distance: A New Approach to Learning Quantum Data

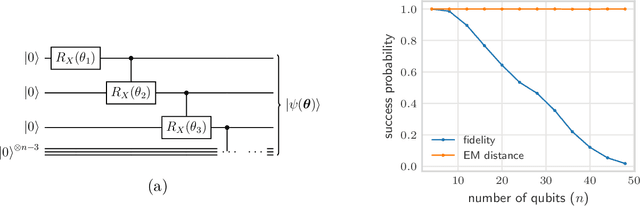
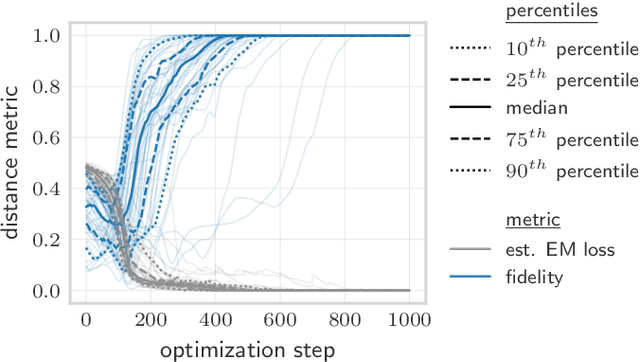
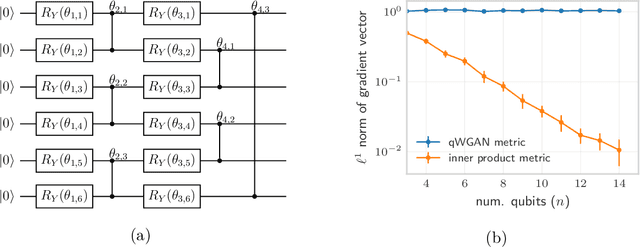
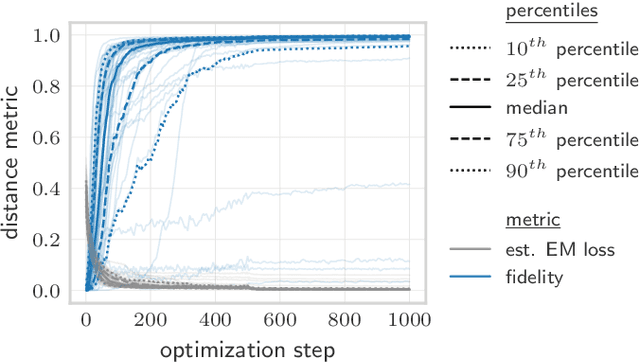
Quantifying how far the output of a learning algorithm is from its target is an essential task in machine learning. However, in quantum settings, the loss landscapes of commonly used distance metrics often produce undesirable outcomes such as poor local minima and exponentially decaying gradients. As a new approach, we consider here the quantum earth mover's (EM) or Wasserstein-1 distance, recently proposed in [De Palma et al., arXiv:2009.04469] as a quantum analog to the classical EM distance. We show that the quantum EM distance possesses unique properties, not found in other commonly used quantum distance metrics, that make quantum learning more stable and efficient. We propose a quantum Wasserstein generative adversarial network (qWGAN) which takes advantage of the quantum EM distance and provides an efficient means of performing learning on quantum data. Our qWGAN requires resources polynomial in the number of qubits, and our numerical experiments demonstrate that it is capable of learning a diverse set of quantum data.