Physics-Informed Statistical Modeling for Wildfire Aerosols Process Using Multi-Source Geostationary Satellite Remote-Sensing Data Streams

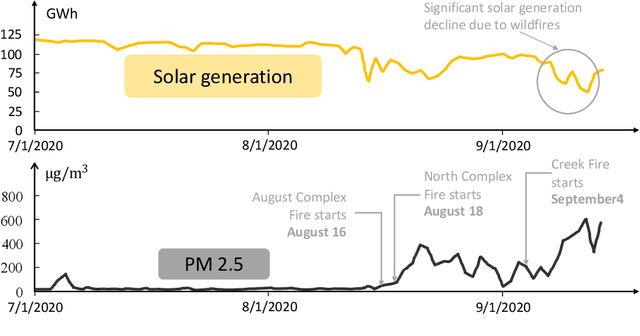
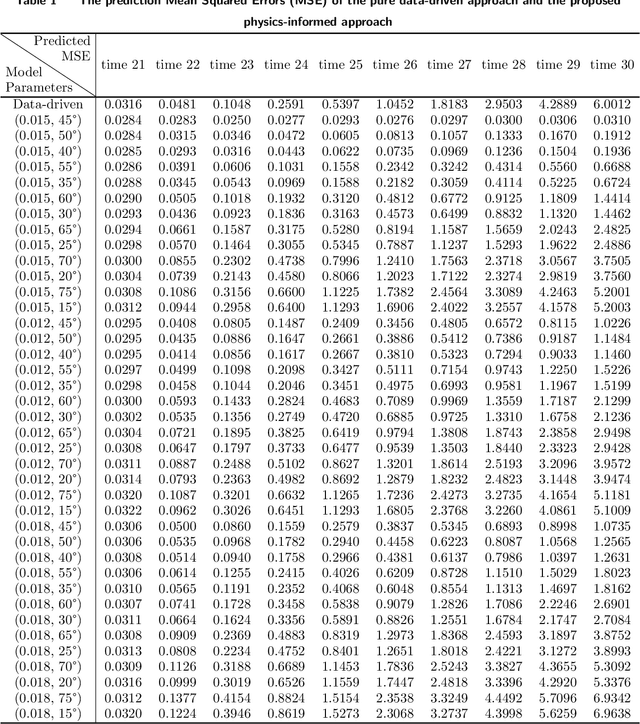
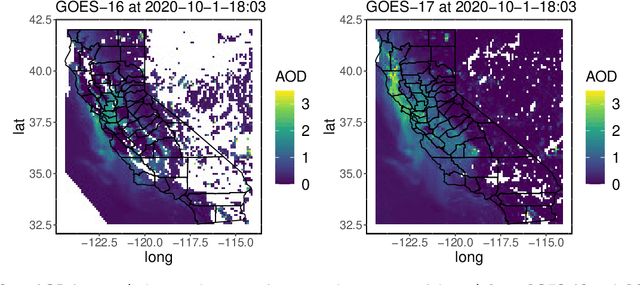
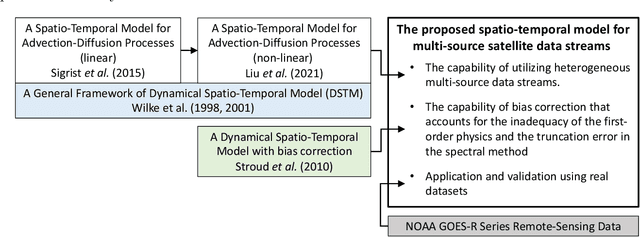
Increasingly frequent wildfires significantly affect solar energy production as the atmospheric aerosols generated by wildfires diminish the incoming solar radiation to the earth. Atmospheric aerosols are measured by Aerosol Optical Depth (AOD), and AOD data streams can be retrieved and monitored by geostationary satellites. However, multi-source remote-sensing data streams often present heterogeneous characteristics, including different data missing rates, measurement errors, systematic biases, and so on. To accurately estimate and predict the underlying AOD propagation process, there exist practical needs and theoretical interests to propose a physics-informed statistical approach for modeling wildfire AOD propagation by simultaneously utilizing, or fusing, multi-source heterogeneous satellite remote-sensing data streams. Leveraging a spectral approach, the proposed approach integrates multi-source satellite data streams with a fundamental advection-diffusion equation that governs the AOD propagation process. A bias correction process is included in the statistical model to account for the bias of the physics model and the truncation error of the Fourier series. The proposed approach is applied to California wildfires AOD data streams obtained from the National Oceanic and Atmospheric Administration. Comprehensive numerical examples are provided to demonstrate the predictive capabilities and model interpretability of the proposed approach. Computer code has been made available on GitHub.

Robust Matrix Completion State Estimation in Distribution Systems

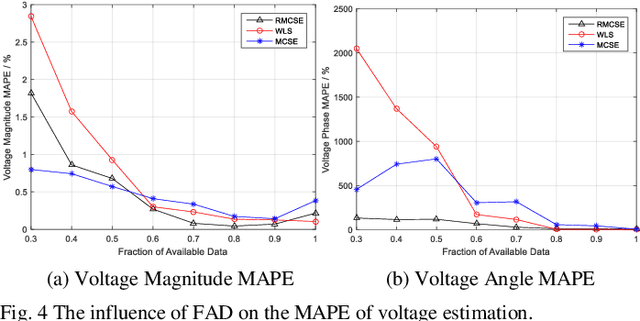
Due to the insufficient measurements in the distribution system state estimation (DSSE), full observability and redundant measurements are difficult to achieve without using the pseudo measurements. The matrix completion state estimation (MCSE) combines the matrix completion and power system model to estimate voltage by exploring the low-rank characteristics of the matrix. This paper proposes a robust matrix completion state estimation (RMCSE) to estimate the voltage in a distribution system under a low-observability condition. Tradition state estimation weighted least squares (WLS) method requires full observability to calculate the states and needs redundant measurements to proceed a bad data detection. The proposed method improves the robustness of the MCSE to bad data by minimizing the rank of the matrix and measurements residual with different weights. It can estimate the system state in a low-observability system and has robust estimates without the bad data detection process in the face of multiple bad data. The method is numerically evaluated on the IEEE 33-node radial distribution system. The estimation performance and robustness of RMCSE are compared with the WLS with the largest normalized residual bad data identification (WLS-LNR), and the MCSE.