Joint Beamforming and Compression Design for Per-Antenna Power Constrained Cooperative Cellular Networks

In the cooperative cellular network, relay-like base stations are connected to the central processor (CP) via rate-limited fronthaul links and the joint processing is performed at the CP, which thus can effectively mitigate the multiuser interference. In this paper, we consider the joint beamforming and compression problem with per-antenna power constraints in the cooperative cellular network. We first establish the equivalence between the considered problem and its semidefinite relaxation (SDR). Then we further derive the partial Lagrangian dual of the SDR problem and show that the objective function of the obtained dual problem is differentiable. Based on the differentiability, we propose two efficient projected gradient ascent algorithms for solving the dual problem, which are projected exact gradient ascent (PEGA) and projected inexact gradient ascent (PIGA). While PEGA is guaranteed to find the global solution of the dual problem (and hence the global solution of the original problem), PIGA is more computationally efficient due to the lower complexity in inexactly computing the gradient. Global optimality and high efficiency of the proposed algorithms are demonstrated via numerical experiments.

QoS-based Beamforming and Compression Design for Cooperative Cellular Networks via Lagrangian Duality

This paper considers the quality-of-service (QoS)-based joint beamforming and compression design problem in the downlink cooperative cellular network, where multiple relay-like base stations (BSs), connected to the central processor via rate-limited fronthaul links, cooperatively transmit messages to the users. The problem of interest is formulated as the minimization of the total transmit power of the BSs, subject to all users' signal-to-interference-plus-noise ratio (SINR) constraints and all BSs' fronthaul rate constraints. In this paper, we first show that there is no duality gap between the considered joint optimization problem and its Lagrangian dual by showing the tightness of its semidefinite relaxation (SDR). Then, we propose an efficient algorithm based on the above duality result for solving the considered problem. The proposed algorithm judiciously exploits the special structure of an enhanced Karush-Kuhn-Tucker (KKT) conditions of the considered problem and finds the solution that satisfies the enhanced KKT conditions via two fixed point iterations. Two key features of the proposed algorithm are: (1) it is able to detect whether the considered problem is feasible or not and find its globally optimal solution when it is feasible; (2) it is highly efficient because both of the fixed point iterations in the proposed algorithm are linearly convergent and evaluating the functions in the fixed point iterations are computationally cheap. Numerical results show the global optimality and efficiency of the proposed algorithm.

Efficiently and Globally Solving Joint Beamforming and Compression Problem in the Cooperative Cellular Network via Lagrangian Duality

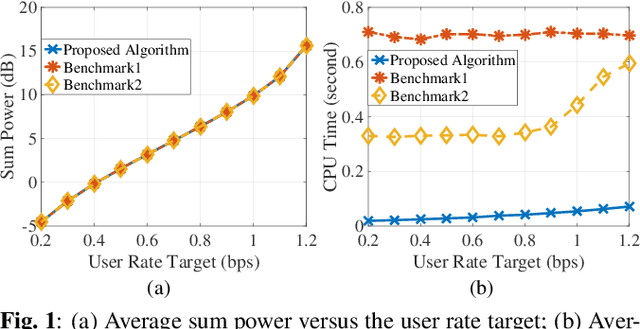
Consider the joint beamforming and quantization problem in the cooperative cellular network, where multiple relay-like base stations (BSs) connected to the central processor (CP) via rate-limited fronthaul links cooperatively serve the users. This problem can be formulated as the minimization of the total transmit power, subject to all users' signal-to-interference-plus-noise-ratio (SINR) constraints and all relay-like BSs' fronthaul rate constraints. In this paper, we first show that there is no duality gap between the considered problem and its Lagrangian dual by showing the tightness of the semidefinite relaxation (SDR) of the considered problem. Then we propose an efficient algorithm based on Lagrangian duality for solving the considered problem. The proposed algorithm judiciously exploits the special structure of the Karush-Kuhn-Tucker (KKT) conditions of the considered problem and finds the solution that satisfies the KKT conditions via two fixed-point iterations. The proposed algorithm is highly efficient (as evaluating the functions in both fixed-point iterations are computationally cheap) and is guaranteed to find the global solution of the problem. Simulation results show the efficiency and the correctness of the proposed algorithm.