Vladimir Vutov
Integrating Transformations in Probabilistic Circuits
Oct 06, 2023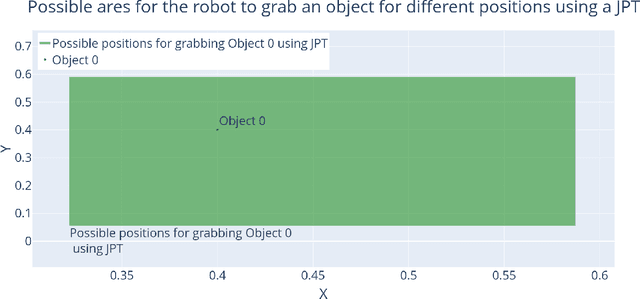
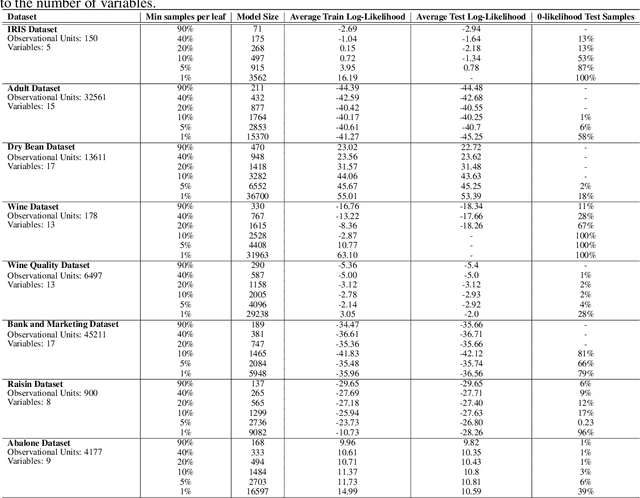
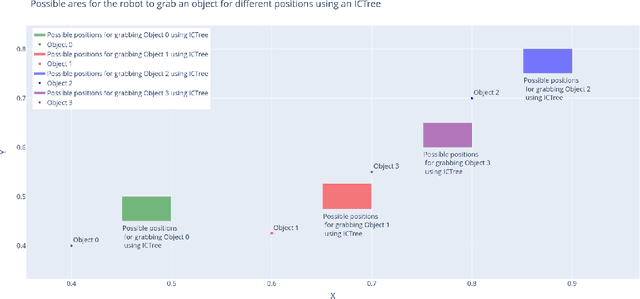
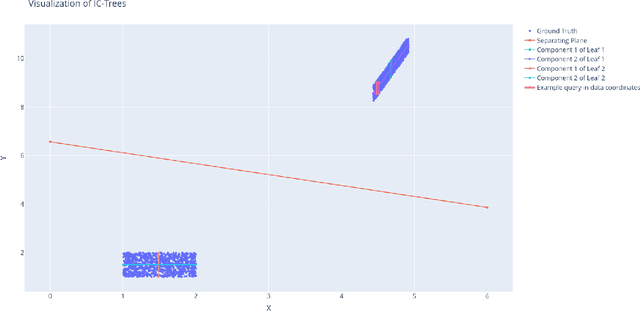
Abstract:This study addresses the predictive limitation of probabilistic circuits and introduces transformations as a remedy to overcome it. We demonstrate this limitation in robotic scenarios. We motivate that independent component analysis is a sound tool to preserve the independence properties of probabilistic circuits. Our approach is an extension of joint probability trees, which are model-free deterministic circuits. By doing so, it is demonstrated that the proposed approach is able to achieve higher likelihoods while using fewer parameters compared to the joint probability trees on seven benchmark data sets as well as on real robot data. Furthermore, we discuss how to integrate transformations into tree-based learning routines. Finally, we argue that exact inference with transformed quantile parameterized distributions is not tractable. However, our approach allows for efficient sampling and approximate inference.
Supervised topological data analysis for MALDI imaging applications
Feb 27, 2023Abstract:We propose a new algebraic topological framework, which obtains intrinsic information from the MALDI data and transforms it to reflect topological persistence in the data. Our framework has two main advantages. First, the topological persistence helps us to distinguish the signal from noise. Second, it compresses the MALDI data, which results in saving storage space, and also optimizes the computational time for further classification tasks. We introduce an algorithm that performs our topological framework and depends on a single tuning parameter. Furthermore, we show that it is computationally efficient. Following the persistence extraction, logistic regression and random forest classifiers are executed based on the resulting persistence transformation diagrams to classify the observational units into binary class labels, describing the lung cancer subtypes. Further, we utilized the proposed framework in a real-world MALDI data set, and the competitiveness of the methods is illustrated via cross-validation.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge