Guided Nonlocal Patch Regularization and Efficient Filtering-Based Inversion for Multiband Fusion

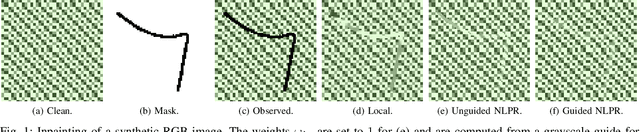
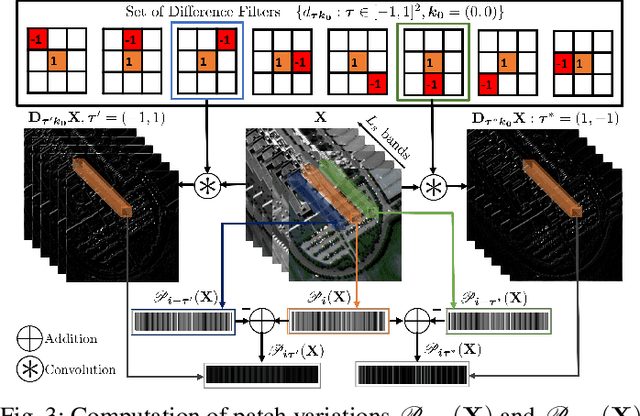
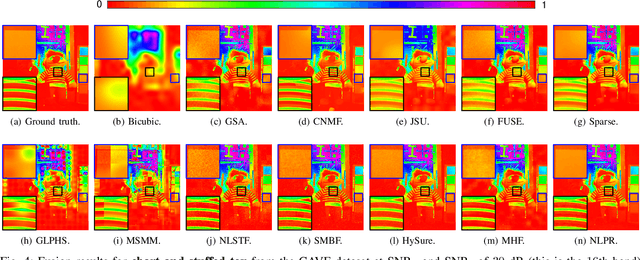
In multiband fusion, an image with a high spatial and low spectral resolution is combined with an image with a low spatial but high spectral resolution to produce a single multiband image having high spatial and spectral resolutions. This comes up in remote sensing applications such as pansharpening~(MS+PAN), hyperspectral sharpening~(HS+PAN), and HS-MS fusion~(HS+MS). Remote sensing images are textured and have repetitive structures. Motivated by nonlocal patch-based methods for image restoration, we propose a convex regularizer that (i) takes into account long-distance correlations, (ii) penalizes patch variation, which is more effective than pixel variation for capturing texture information, and (iii) uses the higher spatial resolution image as a guide image for weight computation. We come up with an efficient ADMM algorithm for optimizing the regularizer along with a standard least-squares loss function derived from the imaging model. The novelty of our algorithm is that by expressing patch variation as filtering operations and by judiciously splitting the original variables and introducing latent variables, we are able to solve the ADMM subproblems efficiently using FFT-based convolution and soft-thresholding. As far as the reconstruction quality is concerned, our method is shown to outperform state-of-the-art variational and deep learning techniques.

Linearized ADMM and Fast Nonlocal Denoising for Efficient Plug-and-Play Restoration

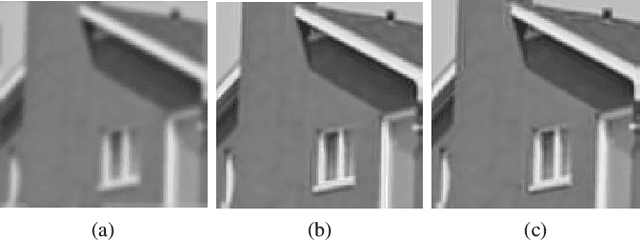
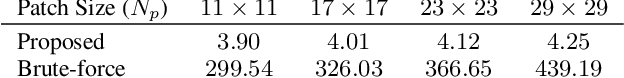
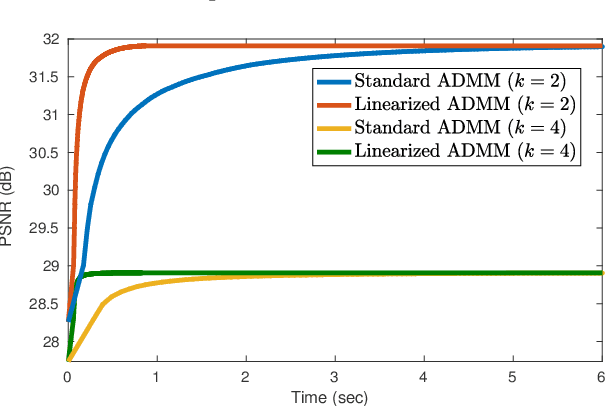
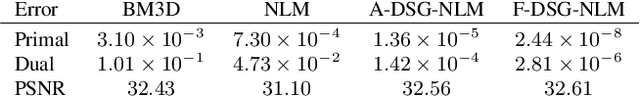
In plug-and-play image restoration, the regularization is performed using powerful denoisers such as nonlocal means (NLM) or BM3D. This is done within the framework of alternating direction method of multipliers (ADMM), where the regularization step is formally replaced by an off-the-shelf denoiser. Each plug-and-play iteration involves the inversion of the forward model followed by a denoising step. In this paper, we present a couple of ideas for improving the efficiency of the inversion and denoising steps. First, we propose to use linearized ADMM, which generally allows us to perform the inversion at a lower cost than standard ADMM. Moreover, we can easily incorporate hard constraints into the optimization framework as a result. Second, we develop a fast algorithm for doubly stochastic NLM, originally proposed by Sreehari et al. (IEEE TCI, 2016), which is about 80x faster than brute-force computation. This particular denoiser can be expressed as the proximal map of a convex regularizer and, as a consequence, we can guarantee convergence for linearized plug-and-play ADMM. We demonstrate the effectiveness of our proposals for super-resolution and single-photon imaging.