Optimizing $αμ$

$\alpha\mu$ is a search algorithm which repairs two defaults of Perfect Information Monte Carlo search: strategy fusion and non locality. In this paper we optimize $\alpha\mu$ for the game of Bridge, avoiding useless computations. The proposed optimizations are general and apply to other imperfect information turn-based games. We define multiple optimizations involving Pareto fronts, and show that these optimizations speed up the search. Some of these optimizations are cuts that stop the search at a node, while others keep track of which possible worlds have become redundant, avoiding unnecessary, costly evaluations. We also measure the benefits of parallelizing the double dummy searches at the leaves of the $\alpha\mu$ search tree.

Construction and Elicitation of a Black Box Model in the Game of Bridge

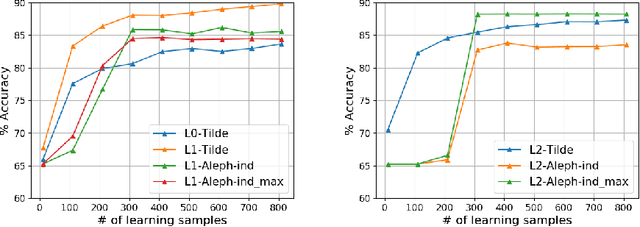
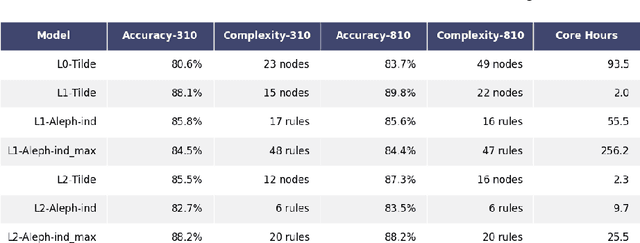
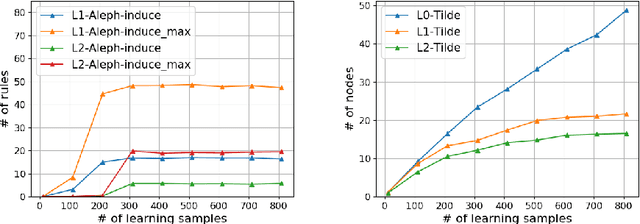
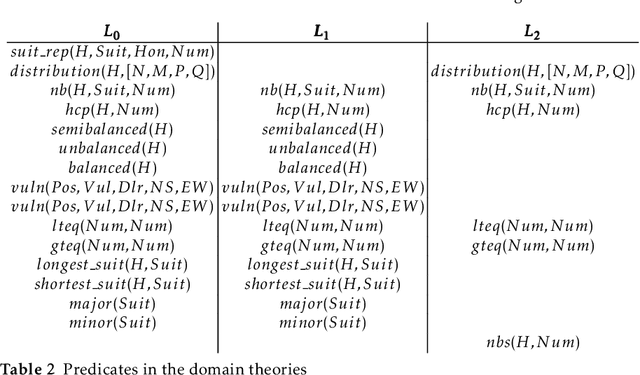
We address the problem of building a decision model for a specific bidding situation in the game of Bridge. We propose the following multi-step methodology i) Build a set of examples for the decision problem and use simulations to associate a decision to each example ii) Use supervised relational learning to build an accurate and readable model iii) Perform a joint analysis between domain experts and data scientists to improve the learning language, including the production by experts of a handmade model iv) Build a better, more readable and accurate model.