APFEx: Adaptive Pareto Front Explorer for Intersectional Fairness

Ensuring fairness in machine learning models is critical, especially when biases compound across intersecting protected attributes like race, gender, and age. While existing methods address fairness for single attributes, they fail to capture the nuanced, multiplicative biases faced by intersectional subgroups. We introduce Adaptive Pareto Front Explorer (APFEx), the first framework to explicitly model intersectional fairness as a joint optimization problem over the Cartesian product of sensitive attributes. APFEx combines three key innovations- (1) an adaptive multi-objective optimizer that dynamically switches between Pareto cone projection, gradient weighting, and exploration strategies to navigate fairness-accuracy trade-offs, (2) differentiable intersectional fairness metrics enabling gradient-based optimization of non-smooth subgroup disparities, and (3) theoretical guarantees of convergence to Pareto-optimal solutions. Experiments on four real-world datasets demonstrate APFEx's superiority, reducing fairness violations while maintaining competitive accuracy. Our work bridges a critical gap in fair ML, providing a scalable, model-agnostic solution for intersectional fairness.

Learning from Heterophilic Graphs: A Spectral Theory Perspective on the Impact of Self-Loops and Parallel Edges

Graph heterophily poses a formidable challenge to the performance of Message-passing Graph Neural Networks (MP-GNNs). The familiar low-pass filters like Graph Convolutional Networks (GCNs) face performance degradation, which can be attributed to the blending of the messages from dissimilar neighboring nodes. The performance of the low-pass filters on heterophilic graphs still requires an in-depth analysis. In this context, we update the heterophilic graphs by adding a number of self-loops and parallel edges. We observe that eigenvalues of the graph Laplacian decrease and increase respectively by increasing the number of self-loops and parallel edges. We conduct several studies regarding the performance of GCN on various benchmark heterophilic networks by adding either self-loops or parallel edges. The studies reveal that the GCN exhibited either increasing or decreasing performance trends on adding self-loops and parallel edges. In light of the studies, we established connections between the graph spectra and the performance trends of the low-pass filters on the heterophilic graphs. The graph spectra characterize the essential intrinsic properties of the input graph like the presence of connected components, sparsity, average degree, cluster structures, etc. Our work is adept at seamlessly evaluating graph spectrum and properties by observing the performance trends of the low-pass filters without pursuing the costly eigenvalue decomposition. The theoretical foundations are also discussed to validate the impact of adding self-loops and parallel edges on the graph spectrum.

Grounding the Ungrounded: A Spectral-Graph Framework for Quantifying Hallucinations in multimodal LLMs

Hallucinations in large language models (LLMs) remain a fundamental obstacle to trustworthy AI, particularly in high-stakes multimodal domains such as medicine, law, and finance. Existing evaluation techniques are largely heuristic -- anchored in qualitative benchmarking or ad-hoc empirical mitigation -- providing neither principled quantification nor actionable theoretical guarantees. This gap leaves a critical blind spot in understanding how hallucinations arise, propagate, and interact across modalities. We introduce the first (to our knowledge) rigorous information geometric framework in diffusion dynamics for quantifying hallucinations in multimodal LLMs (MLLMs), advancing the field from qualitative detection to mathematically grounded measurement. Our approach represents MLLM outputs as the spectral embeddings over multimodal graph Laplacians and characterizes the manifold gaps of truth vs inconsistencies as the semantic distortion, enabling the tight Rayleigh--Ritz bounds on the multimodal hallucination energy as a functional of time-dependent temperature profiles. By leveraging eigenmode decompositions in Reproducing Kernel Hilbert Space (RKHS) embeddings, our framework delivers modality-aware, theoretically interpretable metrics that capture the evolution of hallucinations across time and input prompts through temperature annealing. This work establishes a principled foundation for quantifying and bounding hallucinations, transforming them from a qualitative risk to a tractable, analyzable phenomenon.

Relation-Aware Slicing in Cross-Domain Alignment

The Sliced Gromov-Wasserstein (SGW) distance, aiming to relieve the computational cost of solving a non-convex quadratic program that is the Gromov-Wasserstein distance, utilizes projecting directions sampled uniformly from unit hyperspheres. This slicing mechanism incurs unnecessary computational costs due to uninformative directions, which also affects the representative power of the distance. However, finding a more appropriate distribution over the projecting directions (slicing distribution) is often an optimization problem in itself that comes with its own computational cost. In addition, with more intricate distributions, the sampling itself may be expensive. As a remedy, we propose an optimization-free slicing distribution that provides fast sampling for the Monte Carlo approximation. We do so by introducing the Relation-Aware Projecting Direction (RAPD), effectively capturing the pairwise association of each of two pairs of random vectors, each following their ambient law. This enables us to derive the Relation-Aware Slicing Distribution (RASD), a location-scale law corresponding to sampled RAPDs. Finally, we introduce the RASGW distance and its variants, e.g., IWRASGW (Importance Weighted RASGW), which overcome the shortcomings experienced by SGW. We theoretically analyze its properties and substantiate its empirical prowess using extensive experiments on various alignment tasks.

Assessing the Limits of In-Context Learning beyond Functions using Partially Ordered Relation

Generating rational and generally accurate responses to tasks, often accompanied by example demonstrations, highlights Large Language Model's (LLM's) remarkable In-Context Learning (ICL) capabilities without requiring updates to the model's parameter space. Despite having an ongoing exploration focused on the inference from a document-level concept, its behavior in learning well-defined functions or relations in context needs a careful investigation. In this article, we present the performance of ICL on partially ordered relation by introducing the notion of inductively increasing complexity in prompts. In most cases, the saturated performance of the chosen metric indicates that while ICL offers some benefits, its effectiveness remains constrained as we increase the complexity in the prompts even in presence of sufficient demonstrative examples. The behavior is evident from our empirical findings and has further been theoretically justified in term of its implicit optimization process. The code is available \href{https://anonymous.4open.science/r/ICLonPartiallyOrderSet}{here}.

Transformers Are Universally Consistent

Despite their central role in the success of foundational models and large-scale language modeling, the theoretical foundations governing the operation of Transformers remain only partially understood. Contemporary research has largely focused on their representational capacity for language comprehension and their prowess in in-context learning, frequently under idealized assumptions such as linearized attention mechanisms. Initially conceived to model sequence-to-sequence transformations, a fundamental and unresolved question is whether Transformers can robustly perform functional regression over sequences of input tokens. This question assumes heightened importance given the inherently non-Euclidean geometry underlying real-world data distributions. In this work, we establish that Transformers equipped with softmax-based nonlinear attention are uniformly consistent when tasked with executing Ordinary Least Squares (OLS) regression, provided both the inputs and outputs are embedded in hyperbolic space. We derive deterministic upper bounds on the empirical error which, in the asymptotic regime, decay at a provable rate of $\mathcal{O}(t^{-1/2d})$, where $t$ denotes the number of input tokens and $d$ the embedding dimensionality. Notably, our analysis subsumes the Euclidean setting as a special case, recovering analogous convergence guarantees parameterized by the intrinsic dimensionality of the data manifold. These theoretical insights are corroborated through empirical evaluations on real-world datasets involving both continuous and categorical response variables.

Topology-Driven Clustering: Enhancing Performance with Betti Number Filtration

Clustering aims to form groups of similar data points in an unsupervised regime. Yet, clustering complex datasets containing critically intertwined shapes poses significant challenges. The prevailing clustering algorithms widely depend on evaluating similarity measures based on Euclidean metrics. Exploring topological characteristics to perform clustering of complex datasets inevitably presents a better scope. The topological clustering algorithms predominantly perceive the point set through the lens of Simplicial complexes and Persistent homology. Despite these approaches, the existing topological clustering algorithms cannot somehow fully exploit topological structures and show inconsistent performances on some highly complicated datasets. This work aims to mitigate the limitations by identifying topologically similar neighbors through the Vietoris-Rips complex and Betti number filtration. In addition, we introduce the concept of the Betti sequences to capture flexibly essential features from the topological structures. Our proposed algorithm is adept at clustering complex, intertwined shapes contained in the datasets. We carried out experiments on several synthetic and real-world datasets. Our algorithm demonstrated commendable performances across the datasets compared to some of the well-known topology-based clustering algorithms.

Hyperbolic Fuzzy $C$-Means with Adaptive Weight-based Filtering for Clustering in Non-Euclidean Spaces

Clustering algorithms play a pivotal role in unsupervised learning by identifying and grouping similar objects based on shared characteristics. While traditional clustering techniques, such as hard and fuzzy center-based clustering, have been widely used, they struggle with complex, high-dimensional, and non-Euclidean datasets. In particular, the Fuzzy $C$-Means (FCM) algorithm, despite its efficiency and popularity, exhibits notable limitations in non-Euclidean spaces. Euclidean spaces assume linear separability and uniform distance scaling, limiting their effectiveness in capturing complex, hierarchical, or non-Euclidean structures in fuzzy clustering. To overcome these challenges, we introduce Filtration-based Hyperbolic Fuzzy $C$-Means (HypeFCM), a novel clustering algorithm tailored for better representation of data relationships in non-Euclidean spaces. HypeFCM integrates the principles of fuzzy clustering with hyperbolic geometry and employs a weight-based filtering mechanism to improve performance. The algorithm initializes weights using a Dirichlet distribution and iteratively refines cluster centroids and membership assignments based on a hyperbolic metric in the Poincar\'e Disc model. Extensive experimental evaluations demonstrate that HypeFCM significantly outperforms conventional fuzzy clustering methods in non-Euclidean settings, underscoring its robustness and effectiveness.

Generative Adversarial Network based Voice Conversion: Techniques, Challenges, and Recent Advancements

Voice conversion (VC) stands as a crucial research area in speech synthesis, enabling the transformation of a speaker's vocal characteristics to resemble another while preserving the linguistic content. This technology has broad applications, including automated movie dubbing, speech-to-singing conversion, and assistive devices for pathological speech rehabilitation. With the increasing demand for high-quality and natural-sounding synthetic voices, researchers have developed a wide range of VC techniques. Among these, generative adversarial network (GAN)-based approaches have drawn considerable attention for their powerful feature-mapping capabilities and potential to produce highly realistic speech. Despite notable advancements, challenges such as ensuring training stability, maintaining linguistic consistency, and achieving perceptual naturalness continue to hinder progress in GAN-based VC systems. This systematic review presents a comprehensive analysis of the voice conversion landscape, highlighting key techniques, key challenges, and the transformative impact of GANs in the field. The survey categorizes existing methods, examines technical obstacles, and critically evaluates recent developments in GAN-based VC. By consolidating and synthesizing research findings scattered across the literature, this review provides a structured understanding of the strengths and limitations of different approaches. The significance of this survey lies in its ability to guide future research by identifying existing gaps, proposing potential directions, and offering insights for building more robust and efficient VC systems. Overall, this work serves as an essential resource for researchers, developers, and practitioners aiming to advance the state-of-the-art (SOTA) in voice conversion technology.

Collective Learning Mechanism based Optimal Transport Generative Adversarial Network for Non-parallel Voice Conversion

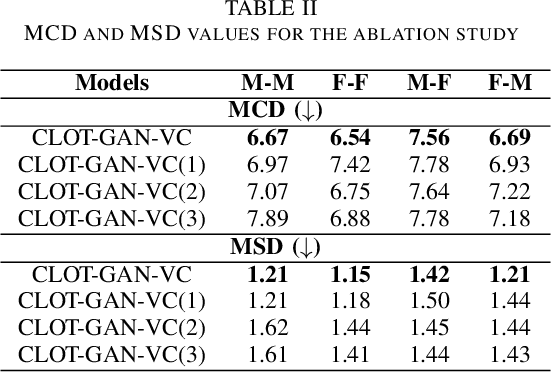
After demonstrating significant success in image synthesis, Generative Adversarial Network (GAN) models have likewise made significant progress in the field of speech synthesis, leveraging their capacity to adapt the precise distribution of target data through adversarial learning processes. Notably, in the realm of State-Of-The-Art (SOTA) GAN-based Voice Conversion (VC) models, there exists a substantial disparity in naturalness between real and GAN-generated speech samples. Furthermore, while many GAN models currently operate on a single generator discriminator learning approach, optimizing target data distribution is more effectively achievable through a single generator multi-discriminator learning scheme. Hence, this study introduces a novel GAN model named Collective Learning Mechanism-based Optimal Transport GAN (CLOT-GAN) model, incorporating multiple discriminators, including the Deep Convolutional Neural Network (DCNN) model, Vision Transformer (ViT), and conformer. The objective of integrating various discriminators lies in their ability to comprehend the formant distribution of mel-spectrograms, facilitated by a collective learning mechanism. Simultaneously, the inclusion of Optimal Transport (OT) loss aims to precisely bridge the gap between the source and target data distribution, employing the principles of OT theory. The experimental validation on VCC 2018, VCTK, and CMU-Arctic datasets confirms that the CLOT-GAN-VC model outperforms existing VC models in objective and subjective assessments.