Steve Manns
Robust Modeling of Unknown Dynamical Systems via Ensemble Averaged Learning
Mar 07, 2022
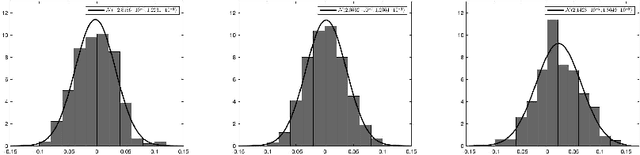

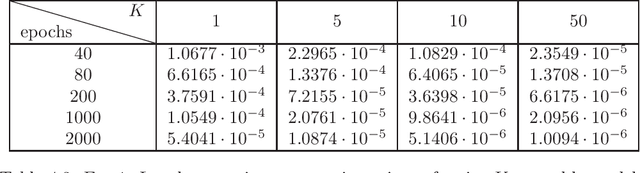
Abstract:Recent work has focused on data-driven learning of the evolution of unknown systems via deep neural networks (DNNs), with the goal of conducting long time prediction of the evolution of the unknown system. Training a DNN with low generalization error is a particularly important task in this case as error is accumulated over time. Because of the inherent randomness in DNN training, chiefly in stochastic optimization, there is uncertainty in the resulting prediction, and therefore in the generalization error. Hence, the generalization error can be viewed as a random variable with some probability distribution. Well-trained DNNs, particularly those with many hyperparameters, typically result in probability distributions for generalization error with low bias but high variance. High variance causes variability and unpredictably in the results of a trained DNN. This paper presents a computational technique which decreases the variance of the generalization error, thereby improving the reliability of the DNN model to generalize consistently. In the proposed ensemble averaging method, multiple models are independently trained and model predictions are averaged at each time step. A mathematical foundation for the method is presented, including results regarding the distribution of the local truncation error. In addition, three time-dependent differential equation problems are considered as numerical examples, demonstrating the effectiveness of the method to decrease variance of DNN predictions generally.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge