A theoretical framework for reservoir computing on networks of organic electrochemical transistors

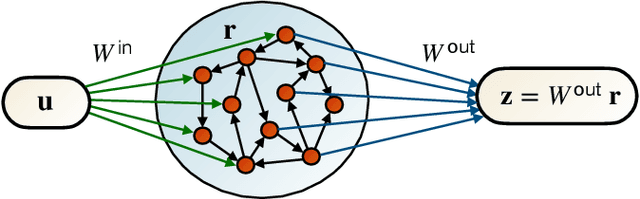
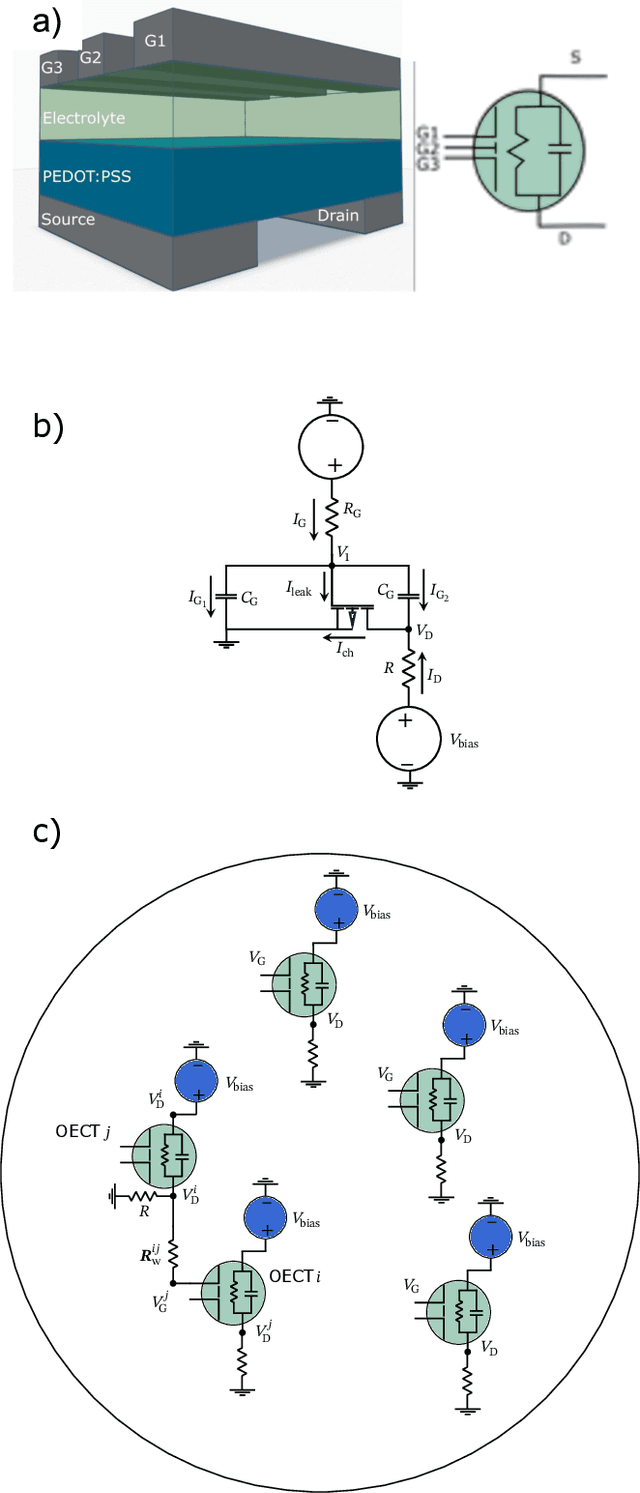
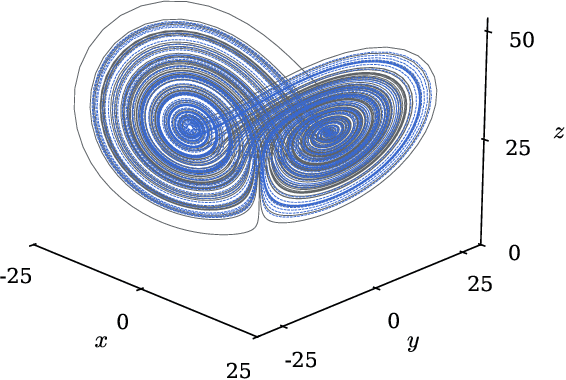
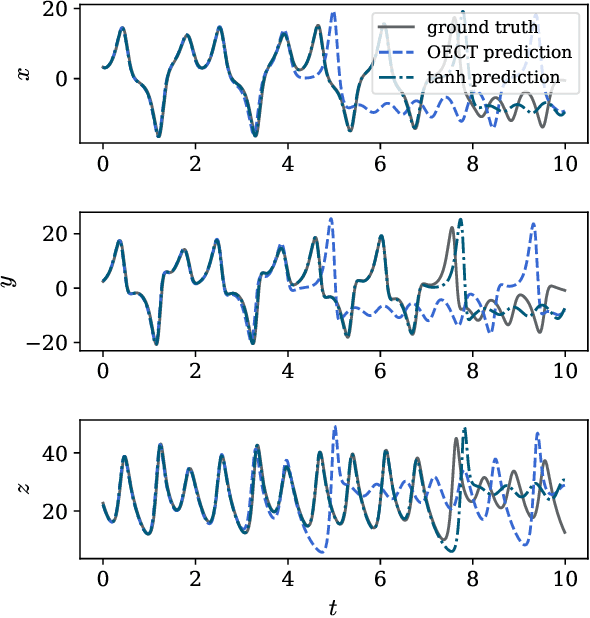
Efficient and accurate prediction of physical systems is important even when the rules of those systems cannot be easily learned. Reservoir computing, a type of recurrent neural network with fixed nonlinear units, is one such prediction method and is valued for its ease of training. Organic electrochemical transistors (OECTs) are physical devices with nonlinear transient properties that can be used as the nonlinear units of a reservoir computer. We present a theoretical framework for simulating reservoir computers using OECTs as the non-linear units as a test bed for designing physical reservoir computers. We present a proof of concept demonstrating that such an implementation can accurately predict the Lorenz attractor with comparable performance to standard reservoir computer implementations. We explore the effect of operating parameters and find that the prediction performance strongly depends on the pinch-off voltage of the OECTs.

Tuning the activation function to optimize the forecast horizon of a reservoir computer

Reservoir computing is a machine learning framework where the readouts from a nonlinear system (the reservoir) are trained so that the output from the reservoir, when forced with an input signal, reproduces a desired output signal. A common implementation of reservoir computers is to use a recurrent neural network as the reservoir. The design of this network can have significant effects on the performance of the reservoir computer. In this paper we study the effect of the node activation function on the ability of reservoir computers to learn and predict chaotic time series. We find that the Forecast Horizon (FH), the time during which the reservoir's predictions remain accurate, can vary by an order of magnitude across a set of 16 activation functions used in machine learning. By using different functions from this set, and by modifying their parameters, we explore whether the entropy of node activation levels or the curvature of the activation functions determine the predictive ability of the reservoirs. We find that the FH is low when the activation function is used in a region where it has low curvature, and a positive correlation between curvature and FH. For the activation functions studied we find that the largest FH generally occurs at intermediate levels of the entropy of node activation levels. Our results show that the performance of reservoir computers is very sensitive to the activation function shape. Therefore, modifying this shape in hyperparameter optimization algorithms can lead to improvements in reservoir computer performance.