Legal Summarisation through LLMs: The PRODIGIT Project

We present some initial results of a large-scale Italian project called PRODIGIT which aims to support tax judges and lawyers through digital technology, focusing on AI. We have focused on generation of summaries of judicial decisions and on the extraction of related information, such as the identification of legal issues and decision-making criteria, and the specification of keywords. To this end, we have deployed and evaluated different tools and approaches to extractive and abstractive summarisation. We have applied LLMs, and particularly on GPT4, which has enabled us to obtain results that proved satisfactory, according to an evaluation by expert tax judges and lawyers. On this basis, a prototype application is being built which will be made publicly available.

Anomaly Detection based on Compressed Data: an Information Theoretic Characterization

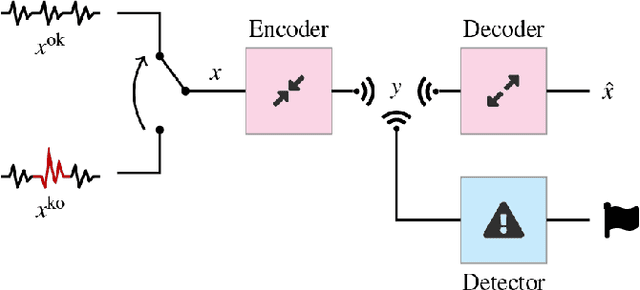
We analyze the effect of lossy compression in the processing of sensor signals that must be used to detect anomalous events in the system under observation. The intuitive relationship between the quality loss at higher compression and the possibility of telling anomalous behaviours from normal ones is formalized in terms of information-theoretic quantities. Some analytic derivations are made within the Gaussian framework and possibly in the asymptotic regime for what concerns the stretch of signals considered. Analytical conclusions are matched with the performance of practical detectors in a toy case allowing the assessment of different compression/detector configurations.

Rakeness in the design of Analog-to-Information Conversion of Sparse and Localized Signals

Design of Random Modulation Pre-Integration systems based on the restricted-isometry property may be suboptimal when the energy of the signals to be acquired is not evenly distributed, i.e. when they are both sparse and localized. To counter this, we introduce an additional design criterion, that we call rakeness, accounting for the amount of energy that the measurements capture from the signal to be acquired. Hence, for localized signals a proper system tuning increases the rakeness as well as the average SNR of the samples used in its reconstruction. Yet, maximizing average SNR may go against the need of capturing all the components that are potentially non-zero in a sparse signal, i.e., against the restricted isometry requirement ensuring reconstructability. What we propose is to administer the trade-off between rakeness and restricted isometry in a statistical way by laying down an optimization problem. The solution of such an optimization problem is the statistic of the process generating the random waveforms onto which the signal is projected to obtain the measurements. The formal definition of such a problems is given as well as its solution for signals that are either localized in frequency or in more generic domain. Sample applications, to ECG signals and small images of printed letters and numbers, show that rakeness-based design leads to non-negligible improvements in both cases.