Optimal Learning

This paper studies the problem of learning an unknown function $f$ from given data about $f$. The learning problem is to give an approximation $\hat f$ to $f$ that predicts the values of $f$ away from the data. There are numerous settings for this learning problem depending on (i) what additional information we have about $f$ (known as a model class assumption), (ii) how we measure the accuracy of how well $\hat f$ predicts $f$, (iii) what is known about the data and data sites, (iv) whether the data observations are polluted by noise. A mathematical description of the optimal performance possible (the smallest possible error of recovery) is known in the presence of a model class assumption. Under standard model class assumptions, it is shown in this paper that a near optimal $\hat f$ can be found by solving a certain discrete over-parameterized optimization problem with a penalty term. Here, near optimal means that the error is bounded by a fixed constant times the optimal error. This explains the advantage of over-parameterization which is commonly used in modern machine learning. The main results of this paper prove that over-parameterized learning with an appropriate loss function gives a near optimal approximation $\hat f$ of the function $f$ from which the data is collected. Quantitative bounds are given for how much over-parameterization needs to be employed and how the penalization needs to be scaled in order to guarantee a near optimal recovery of $f$. An extension of these results to the case where the data is polluted by additive deterministic noise is also given.

Optimized imaging using non-rigid registration

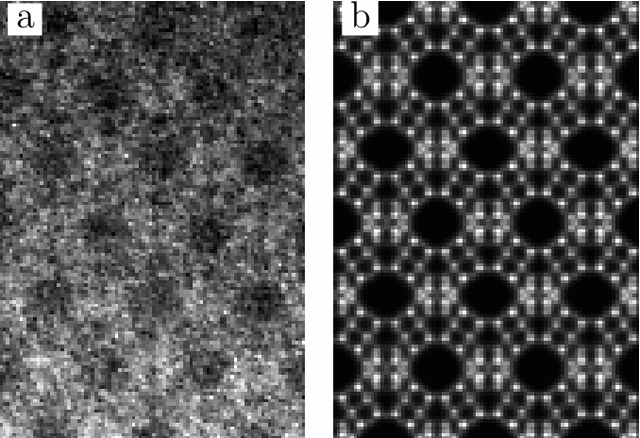
The extraordinary improvements of modern imaging devices offer access to data with unprecedented information content. However, widely used image processing methodologies fall far short of exploiting the full breadth of information offered by numerous types of scanning probe, optical, and electron microscopies. In many applications, it is necessary to keep measurement intensities below a desired threshold. We propose a methodology for extracting an increased level of information by processing a series of data sets suffering, in particular, from high degree of spatial uncertainty caused by complex multiscale motion during the acquisition process. An important role is played by a nonrigid pixel-wise registration method that can cope with low signal-to-noise ratios. This is accompanied by formulating objective quality measures which replace human intervention and visual inspection in the processing chain. Scanning transmission electron microscopy of siliceous zeolite material exhibits the above-mentioned obstructions and therefore serves as orientation and a test of our procedures.