Decimated Prony's Method for Stable Super-resolution

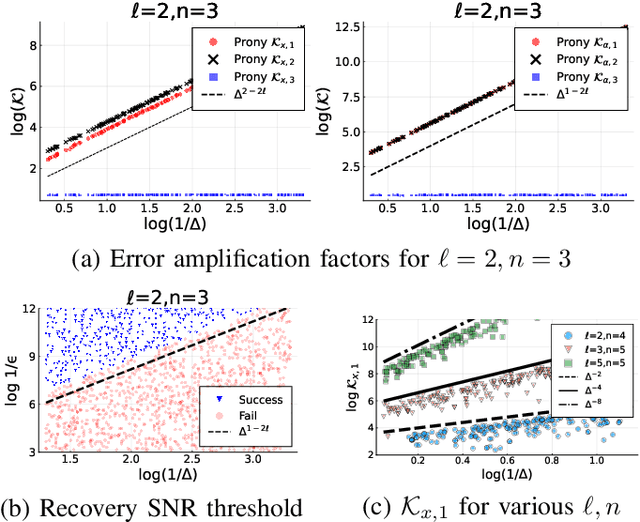
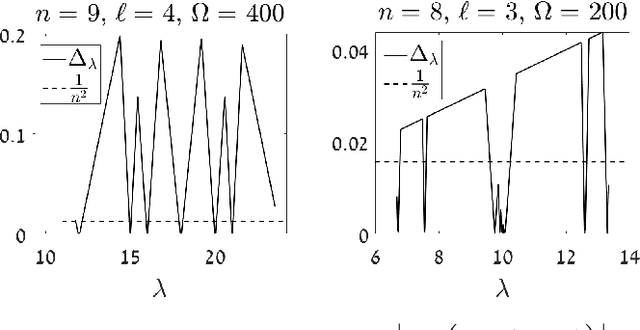
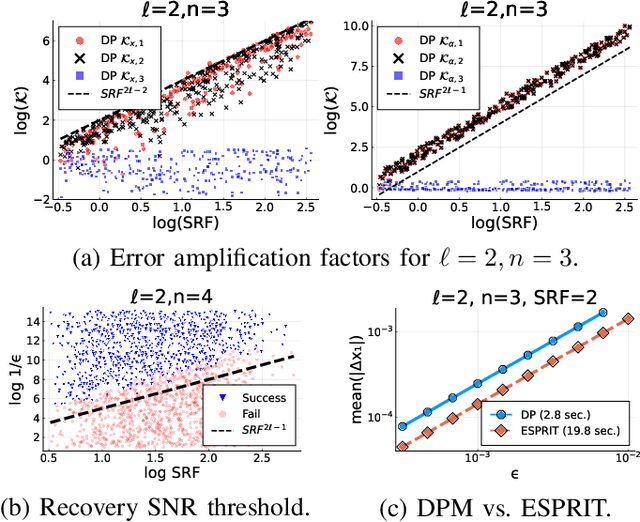
We study recovery of amplitudes and nodes of a finite impulse train from a limited number of equispaced noisy frequency samples. This problem is known as super-resolution (SR) under sparsity constraints and has numerous applications, including direction of arrival and finite rate of innovation sampling. Prony's method is an algebraic technique which fully recovers the signal parameters in the absence of measurement noise. In the presence of noise, Prony's method may experience significant loss of accuracy, especially when the separation between Dirac pulses is smaller than the Nyquist-Shannon-Rayleigh (NSR) limit. In this work we combine Prony's method with a recently established decimation technique for analyzing the SR problem in the regime where the distance between two or more pulses is much smaller than the NSR limit. We show that our approach attains optimal asymptotic stability in the presence of noise. Our result challenges the conventional belief that Prony-type methods tend to be highly numerically unstable.

Non-Adaptive Randomized Algorithm for Group Testing

We study the problem of group testing with a non-adaptive randomized algorithm in the random incidence design (RID) model where each entry in the test is chosen randomly independently from $\{0,1\}$ with a fixed probability $p$. The property that is sufficient and necessary for a unique decoding is the separability of the tests, but unfortunately no linear time algorithm is known for such tests. In order to achieve linear-time decodable tests, the algorithms in the literature use the disjunction property that gives almost optimal number of tests. We define a new property for the tests which we call semi-disjunction property. We show that there is a linear time decoding for such test and for $d\to \infty$ the number of tests converges to the number of tests with the separability property and is therefore optimal (in the RID model). Our analysis shows that, in the RID model, the number of tests in our algorithm is better than the one with the disjunction property even for small $d$.