Accelerating Recursive Partition-Based Causal Structure Learning

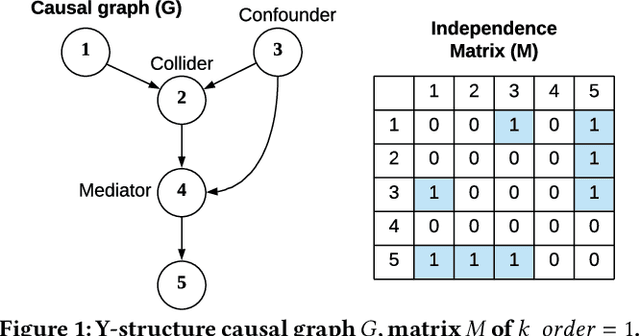
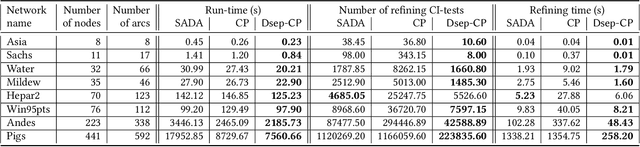
Causal structure discovery from observational data is fundamental to the causal understanding of autonomous systems such as medical decision support systems, advertising campaigns and self-driving cars. This is essential to solve well-known causal decision making and prediction problems associated with those real-world applications. Recently, recursive causal discovery algorithms have gained particular attention among the research community due to their ability to provide good results by using Conditional Independent (CI) tests in smaller sub-problems. However, each of such algorithms needs a refinement function to remove undesired causal relations of the discovered graphs. Notably, with the increase of the problem size, the computation cost (i.e., the number of CI-tests) of the refinement function makes an algorithm expensive to deploy in practice. This paper proposes a generic causal structure refinement strategy that can locate the undesired relations with a small number of CI-tests, thus speeding up the algorithm for large and complex problems. We theoretically prove the correctness of our algorithm. We then empirically evaluate its performance against the state-of-the-art algorithms in terms of solution quality and completion time in synthetic and real datasets.

Improving Solution Quality of Bounded Max-Sum Algorithm to Solve DCOPs involving Hard and Soft Constraints

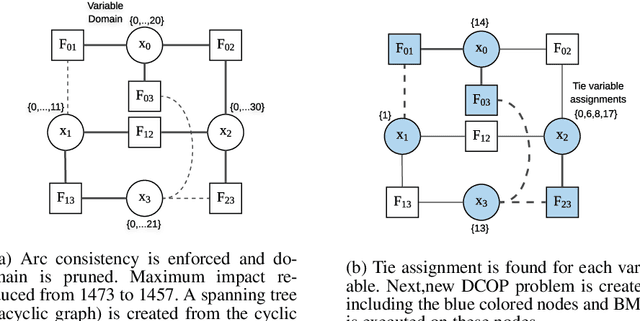
Bounded Max-Sum (BMS) is a message-passing algorithm that provides approximation solution to a specific form of de-centralized coordination problems, namely Distributed Constrained Optimization Problems (DCOPs). In particular, BMS algorithm is able to solve problems of this type having large search space at the expense of low computational cost. Notably, the traditional DCOP formulation does not consider those constraints that must be satisfied(also known as hard constraints), rather it concentrates only on soft constraints. Hence, although the presence of both types of constraints are observed in a number of real-world applications, the BMS algorithm does not actively capitalize on the hard constraints. To address this issue, we tailor BMS in such a way that can deal with DCOPs having both type constraints. In so doing, our approach improves the solution quality of the algorithm. The empirical results exhibit a marked improvement in the quality of the solutions of large DCOPs.

Speeding Up Distributed Pseudo-tree Optimization Procedure with Cross Edge Consistency to Solve DCOPs

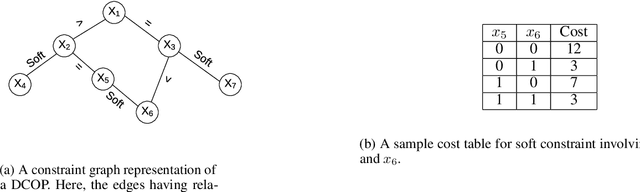
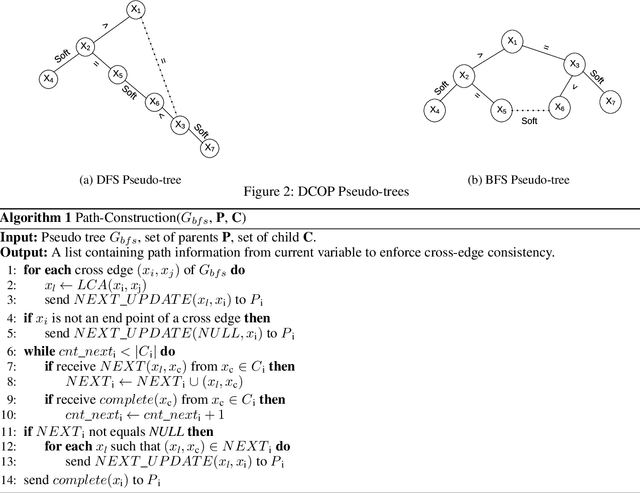
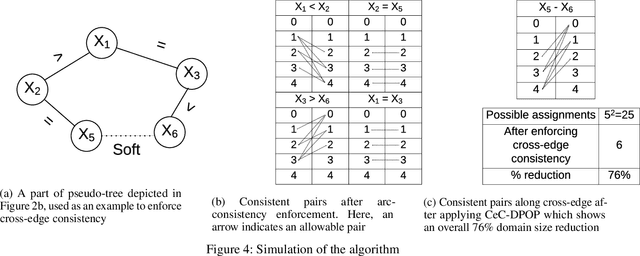
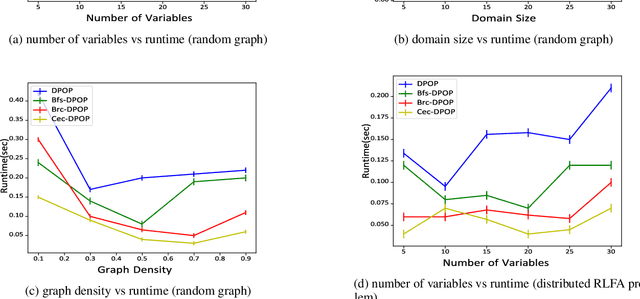
Distributed Pseudo-tree Optimization Procedure (DPOP) is a well-known message passing algorithm that has been used to provide optimal solutions of Distributed Constraint Optimization Problems (DCOPs) -- a framework that is designed to optimize constraints in cooperative multi-agent systems. The traditional DCOP formulation does not consider those constraints that must be satisfied (also known as hard constraints), rather it concentrates only on soft constraints. However, the presence of both types of constraints are observed in a number of applications, such as Distributed Radio Link Frequency Assignment and Distributed Event Scheduling, etc. Although the combination of these types of constraints is recently incorporated in DPOP to solve DCOPs, scalability remains an issue for them as finding an optimal solution is NP-hard. Additionally, in DPOP, the agents are arranged as a DFS pseudo-tree. Recently it has been observed that the constructed pseudo-trees in this way often come to be chain-like and greatly impair the algorithm's performance. To address these issues, we develop an algorithm that speeds up the DPOP algorithm by reducing the size of the messages exchanged and increasing parallelism in the pseudo tree. Our empirical evidence suggests that our approach outperforms the state-of-the-art algorithms by a significant margin.