Bi-Lipschitz Ansatz for Anti-Symmetric Functions

Motivated by applications for simulating quantum many body functions, we propose a new universal ansatz for approximating anti-symmetric functions. The main advantage of this ansatz over previous alternatives is that it is bi-Lipschitz with respect to a naturally defined metric. As a result, we are able to obtain quantitative approximation results for approximation of Lipschitz continuous antisymmetric functions. Moreover, we provide preliminary experimental evidence to the improved performance of this ansatz for learning antisymmetric functions.

On Bi-gram Graph Attributes

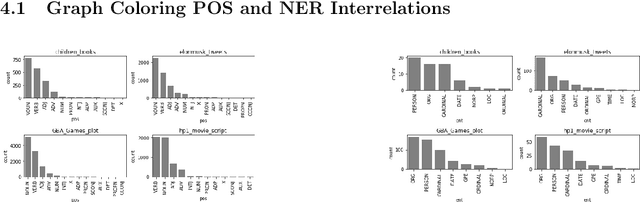
We propose a new approach to text semantic analysis and general corpus analysis using, as termed in this article, a "bi-gram graph" representation of a corpus. The different attributes derived from graph theory are measured and analyzed as unique insights or against other corpus graphs. We observe a vast domain of tools and algorithms that can be developed on top of the graph representation; creating such a graph proves to be computationally cheap, and much of the heavy lifting is achieved via basic graph calculations. Furthermore, we showcase the different use-cases for the bi-gram graphs and how scalable it proves to be when dealing with large datasets.