Marcin Bienkowski
Contract Scheduling with Distributional and Multiple Advice
Apr 18, 2024Abstract:Contract scheduling is a widely studied framework for designing real-time systems with interruptible capabilities. Previous work has showed that a prediction on the interruption time can help improve the performance of contract-based systems, however it has relied on a single prediction that is provided by a deterministic oracle. In this work, we introduce and study more general and realistic learning-augmented settings in which the prediction is in the form of a probability distribution, or it is given as a set of multiple possible interruption times. For both prediction settings, we design and analyze schedules which perform optimally if the prediction is accurate, while simultaneously guaranteeing the best worst-case performance if the prediction is adversarial. We also provide evidence that the resulting system is robust to prediction errors in the distributional setting. Last, we present an experimental evaluation that confirms the theoretical findings, and illustrates the performance improvements that can be attained in practice.
An Improved Algorithm For Online Reranking
Sep 11, 2022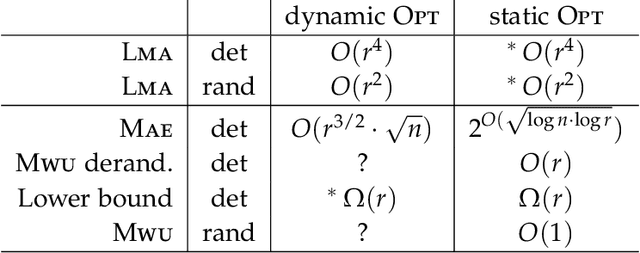
Abstract:We study a fundamental model of online preference aggregation, where an algorithm maintains an ordered list of $n$ elements. An input is a stream of preferred sets $R_1, R_2, \dots, R_t, \dots$. Upon seeing $R_t$ and without knowledge of any future sets, an algorithm has to rerank elements (change the list ordering), so that at least one element of $R_t$ is found near the list front. The incurred cost is a sum of the list update costs (the number of swaps of neighboring list elements) and access costs (position of the first element of $R_t$ on the list). This scenario occurs naturally in applications such as ordering items in an online shop using aggregated preferences of shop customers. The theoretical underpinning of this problem is known as Min-Sum Set Cover. Unlike previous work (Fotakis et al., ICALP 2020, NIPS 2020) that mostly studied the performance of an online algorithm ALG against the static optimal solution (a single optimal list ordering), in this paper, we study an arguably harder variant where the benchmark is the provably stronger optimal dynamic solution OPT (that may also modify the list ordering). In terms of an online shop, this means that the aggregated preferences of its user base evolve with time. We construct a computationally efficient randomized algorithm whose competitive ratio (ALG-to-OPT cost ratio) is $O(r^2)$ and prove the existence of a deterministic $O(r^4)$-competitive algorithm. Here, $r$ is the maximum cardinality of sets $R_t$. This is the first algorithm whose ratio does not depend on $n$: the previously best algorithm for this problem was $O(r^{3/2} \cdot \sqrt{n})$-competitive and $\Omega(r)$ is a lower bound on the performance of any deterministic online algorithm.
 Add to Chrome
Add to Chrome Add to Firefox
Add to Firefox Add to Edge
Add to Edge