Spatially Resolved Meteorological and Ancillary Data in Central Europe for Rainfall Streamflow Modeling

We present a dataset for rainfall streamflow modeling that is fully spatially resolved with the aim of taking neural network-driven hydrological modeling beyond lumped catchments. To this end, we compiled data covering five river basins in central Europe: upper Danube, Elbe, Oder, Rhine, and Weser. The dataset contains meteorological forcings, as well as ancillary information on soil, rock, land cover, and orography. The data is harmonized to a regular 9km times 9km grid and contains daily values that span from October 1981 to September 2011. We also provide code to further combine our dataset with publicly available river discharge data for end-to-end rainfall streamflow modeling.

On Lottery Tickets and Minimal Task Representations in Deep Reinforcement Learning

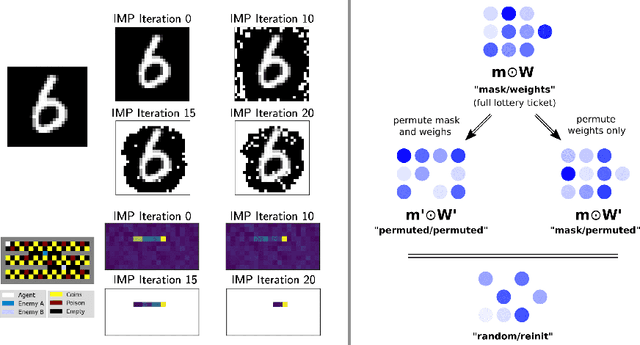
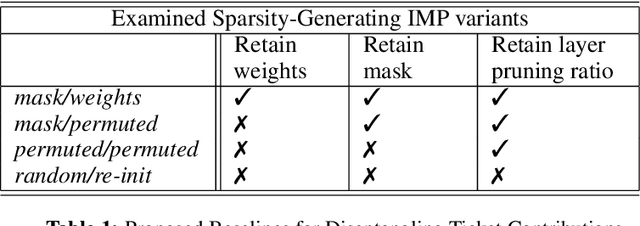
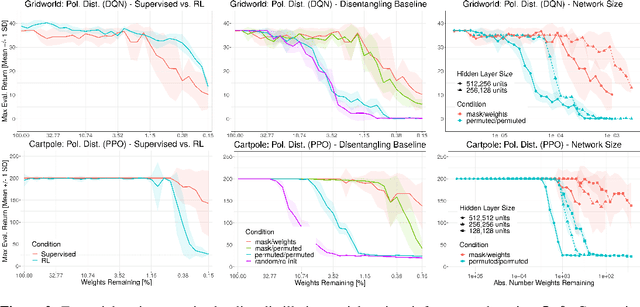
The lottery ticket hypothesis questions the role of overparameterization in supervised deep learning. But how is the performance of winning lottery tickets affected by the distributional shift inherent to reinforcement learning problems? In this work, we address this question by comparing sparse agents who have to address the non-stationarity of the exploration-exploitation problem with supervised agents trained to imitate an expert. We show that feed-forward networks trained via reinforcement learning and imitation learning can be pruned to the same level of sparsity, suggesting that the distributional shift has a limited impact on the size of winning tickets. Using a set of carefully designed baseline conditions, we find that the majority of the lottery ticket effect in both learning paradigms can be attributed to the identified mask rather than the weight initialization. The input layer mask selectively prunes entire input dimensions that turn out to be irrelevant for the task at hand. At a moderate level of sparsity the mask identified by iterative magnitude pruning yields minimal task-relevant representations, i.e., an interpretable inductive bias. Finally, we propose a simple initialization rescaling which promotes the robust identification of sparse task representations in low-dimensional control tasks.