A statistical physics approach to learning curves for the Inverse Ising problem

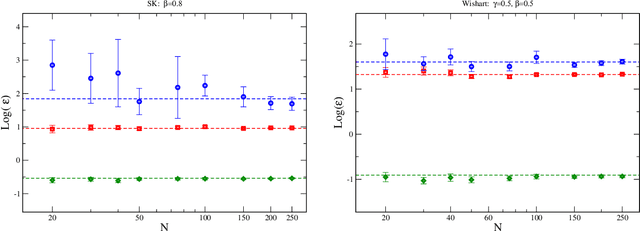
Using methods of statistical physics, we analyse the error of learning couplings in large Ising models from independent data (the inverse Ising problem). We concentrate on learning based on local cost functions, such as the pseudo-likelihood method for which the couplings are inferred independently for each spin. Assuming that the data are generated from a true Ising model, we compute the reconstruction error of the couplings using a combination of the replica method with the cavity approach for densely connected systems. We show that an explicit estimator based on a quadratic cost function achieves minimal reconstruction error, but requires the length of the true coupling vector as prior knowledge. A simple mean field estimator of the couplings which does not need such knowledge is asymptotically optimal, i.e. when the number of observations is much large than the number of spins. Comparison of the theory with numerical simulations shows excellent agreement for data generated from two models with random couplings in the high temperature region: a model with independent couplings (Sherrington-Kirkpatrick model), and a model where the matrix of couplings has a Wishart distribution.

Variational perturbation and extended Plefka approaches to dynamics on random networks: the case of the kinetic Ising model

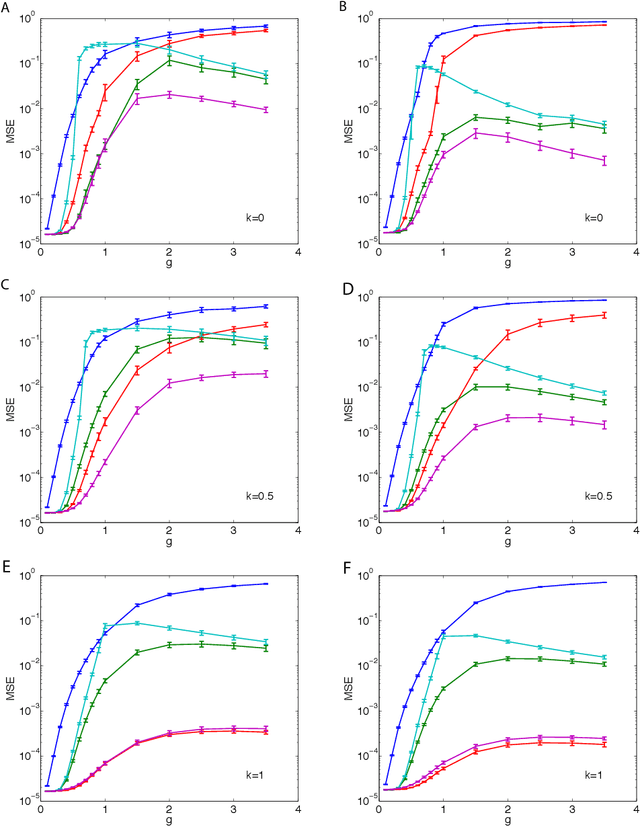
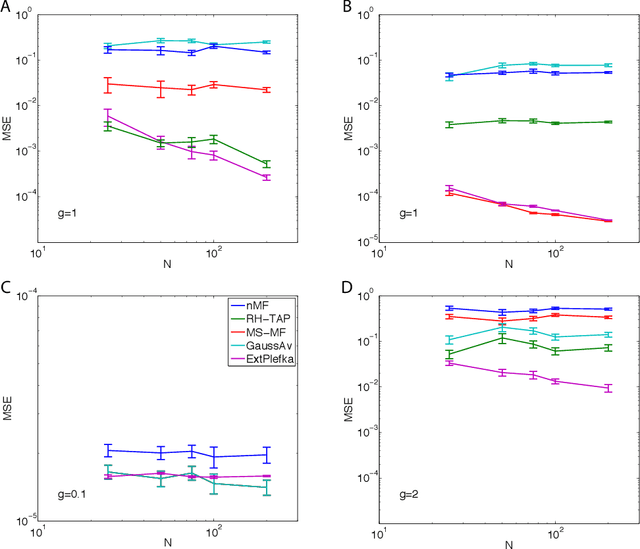
We describe and analyze some novel approaches for studying the dynamics of Ising spin glass models. We first briefly consider the variational approach based on minimizing the Kullback-Leibler divergence between independent trajectories and the real ones and note that this approach only coincides with the mean field equations from the saddle point approximation to the generating functional when the dynamics is defined through a logistic link function, which is the case for the kinetic Ising model with parallel update. We then spend the rest of the paper developing two ways of going beyond the saddle point approximation to the generating functional. In the first one, we develop a variational perturbative approximation to the generating functional by expanding the action around a quadratic function of the local fields and conjugate local fields whose parameters are optimized. We derive analytical expressions for the optimal parameters and show that when the optimization is suitably restricted, we recover the mean field equations that are exact for the fully asymmetric random couplings (M\'ezard and Sakellariou, 2011). However, without this restriction the results are different. We also describe an extended Plefka expansion in which in addition to the magnetization, we also fix the correlation and response functions. Finally, we numerically study the performance of these approximations for Sherrington-Kirkpatrick type couplings for various coupling strengths, degrees of coupling symmetry and external fields. We show that the dynamical equations derived from the extended Plefka expansion outperform the others in all regimes, although it is computationally more demanding. The unconstrained variational approach does not perform well in the small coupling regime, while it approaches dynamical TAP equations of (Roudi and Hertz, 2011) for strong couplings.