A new locally linear embedding scheme in light of Hessian eigenmap

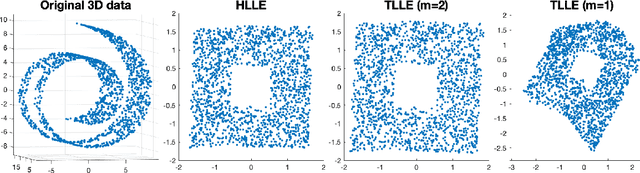
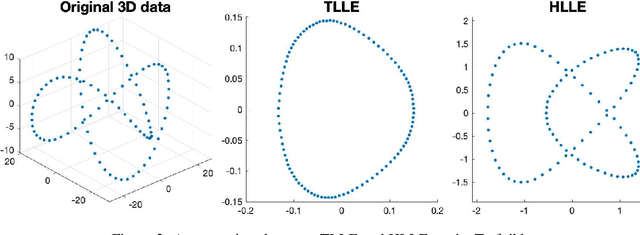
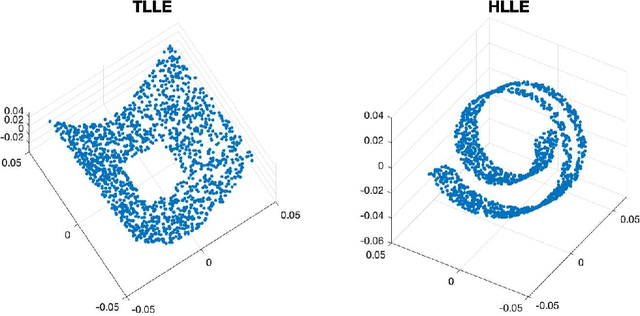
We provide a new interpretation of Hessian locally linear embedding (HLLE), revealing that it is essentially a variant way to implement the same idea of locally linear embedding (LLE). Based on the new interpretation, a substantial simplification can be made, in which the idea of "Hessian" is replaced by rather arbitrary weights. Moreover, we show by numerical examples that HLLE may produce projection-like results when the dimension of the target space is larger than that of the data manifold, and hence one further modification concerning the manifold dimension is suggested. Combining all the observations, we finally achieve a new LLE-type method, which is called tangential LLE (TLLE). It is simpler and more robust than HLLE.

Avoiding unwanted results in locally linear embedding: A new understanding of regularization

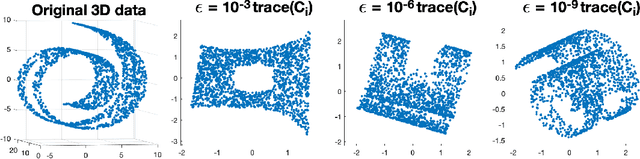
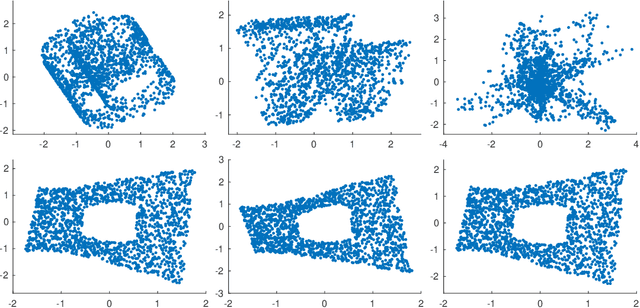
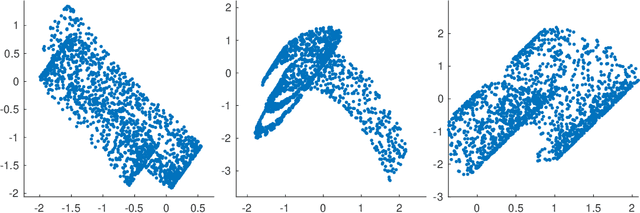
We demonstrate that locally linear embedding (LLE) inherently admits some unwanted results when no regularization is used, even for cases in which regularization is not supposed to be needed in the original algorithm. The existence of one special type of result, which we call ``projection pattern'', is mathematically proved in the situation that an exact local linear relation is achieved in each neighborhood of the data. These special patterns as well as some other bizarre results that may occur in more general situations are shown by numerical examples on the Swiss roll with a hole embedded in a high dimensional space. It is observed that all these bad results can be effectively prevented by using regularization.