Embedding Description Logic Programs into Default Logic

Description logic programs (dl-programs) under the answer set semantics formulated by Eiter {\em et al.} have been considered as a prominent formalism for integrating rules and ontology knowledge bases. A question of interest has been whether dl-programs can be captured in a general formalism of nonmonotonic logic. In this paper, we study the possibility of embedding dl-programs into default logic. We show that dl-programs under the strong and weak answer set semantics can be embedded in default logic by combining two translations, one of which eliminates the constraint operator from nonmonotonic dl-atoms and the other translates a dl-program into a default theory. For dl-programs without nonmonotonic dl-atoms but with the negation-as-failure operator, our embedding is polynomial, faithful, and modular. In addition, our default logic encoding can be extended in a simple way to capture recently proposed weakly well-supported answer set semantics, for arbitrary dl-programs. These results reinforce the argument that default logic can serve as a fruitful foundation for query-based approaches to integrating ontology and rules. With its simple syntax and intuitive semantics, plus available computational results, default logic can be considered an attractive approach to integration of ontology and rules.

Loop Formulas for Description Logic Programs

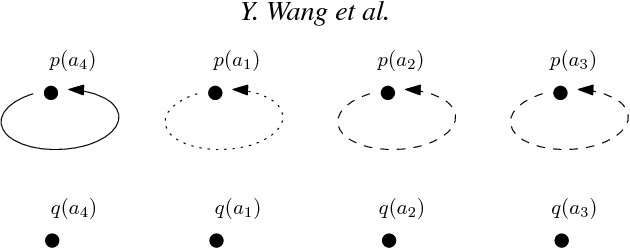
Description Logic Programs (dl-programs) proposed by Eiter et al. constitute an elegant yet powerful formalism for the integration of answer set programming with description logics, for the Semantic Web. In this paper, we generalize the notions of completion and loop formulas of logic programs to description logic programs and show that the answer sets of a dl-program can be precisely captured by the models of its completion and loop formulas. Furthermore, we propose a new, alternative semantics for dl-programs, called the {\em canonical answer set semantics}, which is defined by the models of completion that satisfy what are called canonical loop formulas. A desirable property of canonical answer sets is that they are free of circular justifications. Some properties of canonical answer sets are also explored.