GNN-based Auto-Encoder for Short Linear Block Codes: A DRL Approach

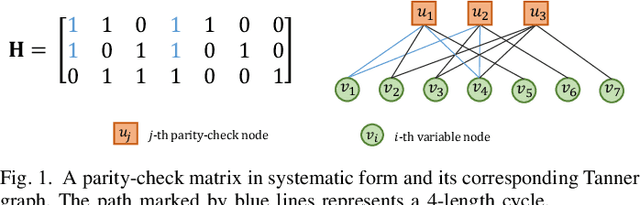
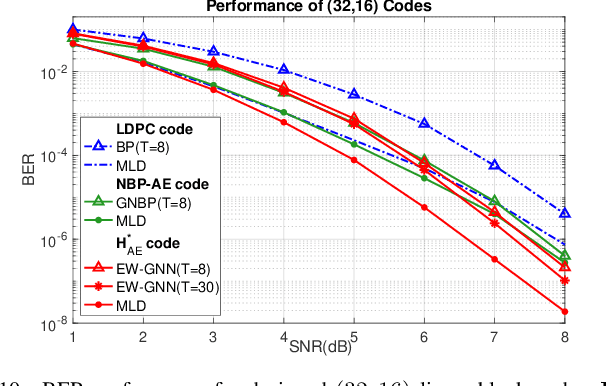
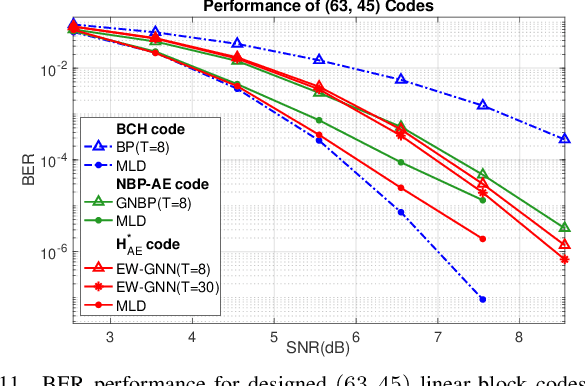
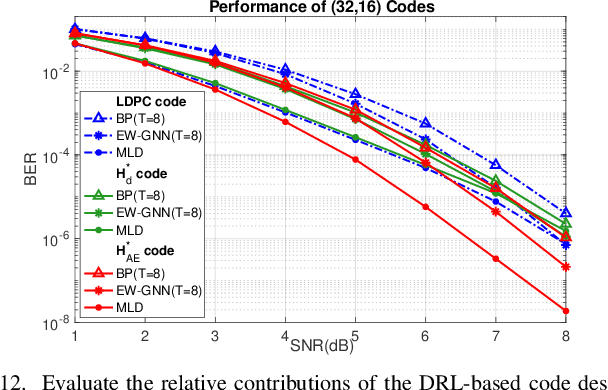
This paper presents a novel auto-encoder based end-to-end channel encoding and decoding. It integrates deep reinforcement learning (DRL) and graph neural networks (GNN) in code design by modeling the generation of code parity-check matrices as a Markov Decision Process (MDP), to optimize key coding performance metrics such as error-rates and code algebraic properties. An edge-weighted GNN (EW-GNN) decoder is proposed, which operates on the Tanner graph with an iterative message-passing structure. Once trained on a single linear block code, the EW-GNN decoder can be directly used to decode other linear block codes of different code lengths and code rates. An iterative joint training of the DRL-based code designer and the EW-GNN decoder is performed to optimize the end-end encoding and decoding process. Simulation results show the proposed auto-encoder significantly surpasses several traditional coding schemes at short block lengths, including low-density parity-check (LDPC) codes with the belief propagation (BP) decoding and the maximum-likelihood decoding (MLD), and BCH with BP decoding, offering superior error-correction capabilities while maintaining low decoding complexity.

A Scalable Graph Neural Network Decoder for Short Block Codes

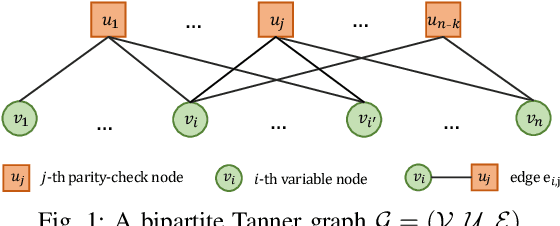
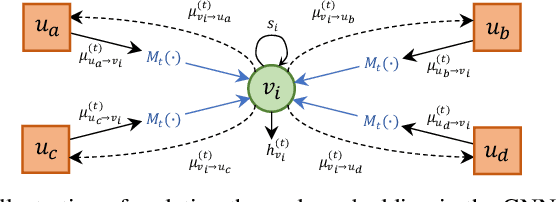
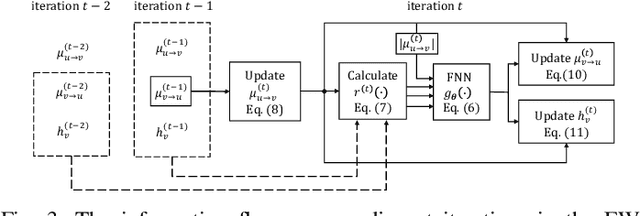
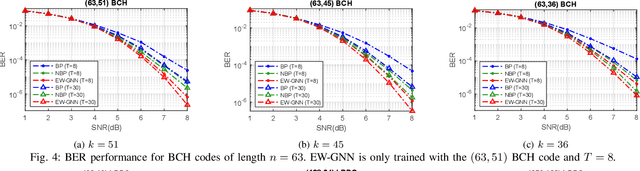
In this work, we propose a novel decoding algorithm for short block codes based on an edge-weighted graph neural network (EW-GNN). The EW-GNN decoder operates on the Tanner graph with an iterative message-passing structure, which algorithmically aligns with the conventional belief propagation (BP) decoding method. In each iteration, the "weight" on the message passed along each edge is obtained from a fully connected neural network that has the reliability information from nodes/edges as its input. Compared to existing deep-learning-based decoding schemes, the EW-GNN decoder is characterised by its scalability, meaning that 1) the number of trainable parameters is independent of the codeword length, and 2) an EW-GNN decoder trained with shorter/simple codes can be directly used for longer/sophisticated codes of different code rates. Furthermore, simulation results show that the EW-GNN decoder outperforms the BP and deep-learning-based BP methods from the literature in terms of the decoding error rate.