Gridless Parameter Estimation in Partly Calibrated Rectangular Arrays

Spatial frequency estimation from a mixture of noisy sinusoids finds applications in various fields. While subspace-based methods offer cost-effective super-resolution parameter estimation, they demand precise array calibration, posing challenges for large antennas. In contrast, sparsity-based approaches outperform subspace methods, especially in scenarios with limited snapshots or correlated sources. This study focuses on direction-of-arrival (DOA) estimation using a partly calibrated rectangular array with fully calibrated subarrays. A gridless sparse formulation leveraging shift invariances in the array is developed, yielding two competitive algorithms under the alternating direction method of multipliers (ADMM) and successive convex approximation frameworks, respectively. Numerical simulations show the superior error performance of our proposed method, particularly in highly correlated scenarios, compared to the conventional subspace-based methods. It is demonstrated that the proposed formulation can also be adopted in the fully calibrated case to improve the robustness of the subspace-based methods to the source correlation. Furthermore, we provide a generalization of the proposed method to a more challenging case where a part of the sensors is unobservable due to failures.

SALSA: A Sequential Alternating Least Squares Approximation Method For MIMO Channel Estimation

In this paper, we consider the channel estimation problem in sub-6 GHz uplink wideband MIMO-OFDM communication systems, where a user equipment with a fully-digital beamforming structure is communicating with a base station having a hybrid analog-digital beamforming structure. A novel channel estimation method called Sequential Alternating Least Squares Approximation (SALSA) is proposed by exploiting a hidden tensor structure in the uplink measurement matrix. Specifically, by showing that any MIMO channel matrix can be approximately decomposed into a summation of R factor matrices having a Kronecker structure, the uplink measurement matrix can be reshaped into a 3-way tensor admitting a Tucker decomposition. Exploiting the tensor structure, the MIMO channel matrix is estimated sequentially using an alternating least squares method. Detailed simulation results are provided showing the effectiveness of the proposed SALSA method as compared to the classical least squares method.

Compressed Sensing Constant Modulus Constrained Projection Matrix Design and High-Resolution DoA Estimation Methods

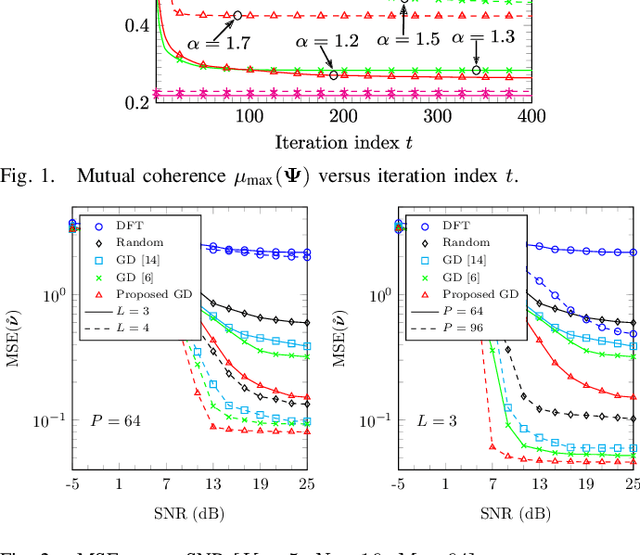
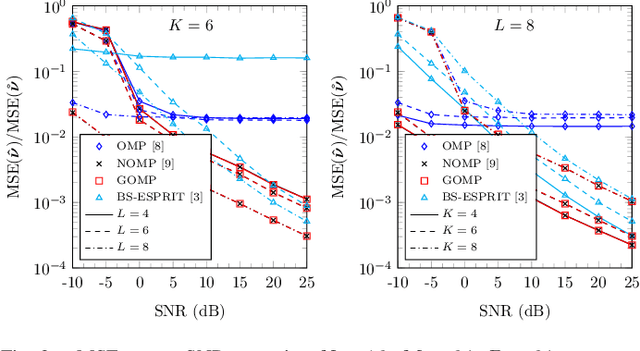
This paper proposes a compressed sensing-based high-resolution direction-of-arrival estimation method called gradient orthogonal matching pursuit (GOMP). It contains two main steps: a sparse coding approximation step using the well-known OMP method and a sequential iterative refinement step using a newly proposed gradient-descent method. To enhance the recoverability, we further propose an efficient projection matrix design method, which considers the constant modulus constraints imposed by the projection matrix hardware components. Simulation results show the effectiveness of the proposed methods as compared to benchmark algorithms.

Double-RIS Versus Single-RIS Aided Systems: Tensor-Based MIMO Channel Estimation and Design Perspectives

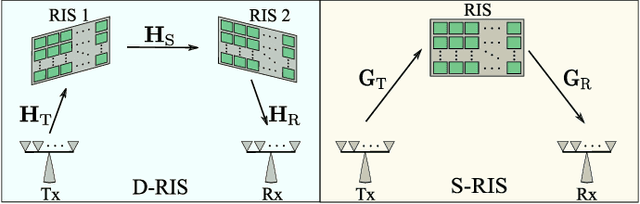
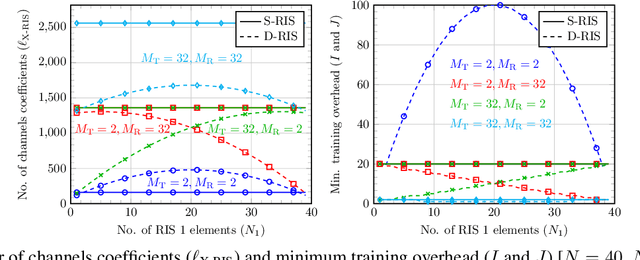
Reconfigurable intelligent surfaces (RISs) have been proposed recently as new technology to tune the wireless propagation channels in real-time. However, most of the current works assume single-RIS (S-RIS)-aided systems, which can be limited in some application scenarios where a transmitter might need a multi-RIS-aided channel to communicate with a receiver. In this paper, we consider a double-RIS (D-RIS)-aided MIMO system and propose an alternating least-squared-based channel estimation method by exploiting the Tucker2 tensor structure of the received signals. Using the proposed method, the cascaded MIMO channel parts can be estimated separately, up to trivial scaling factors. Compared with the S-RIS systems, we show that if the RIS elements of a S-RIS system are distributed carefully between the two RISs in a D-RIS system, the training overhead can be reduced and the estimation accuracy can also be increased. Therefore, D-RIS systems can be seen as an appealing approach to further increase the coverage, capacity, and efficiency of future wireless networks compared to S-RIS systems.

Tensor-Based Channel Estimation and Reflection Design for RIS-Aided Millimeter-Wave MIMO Communication Systems

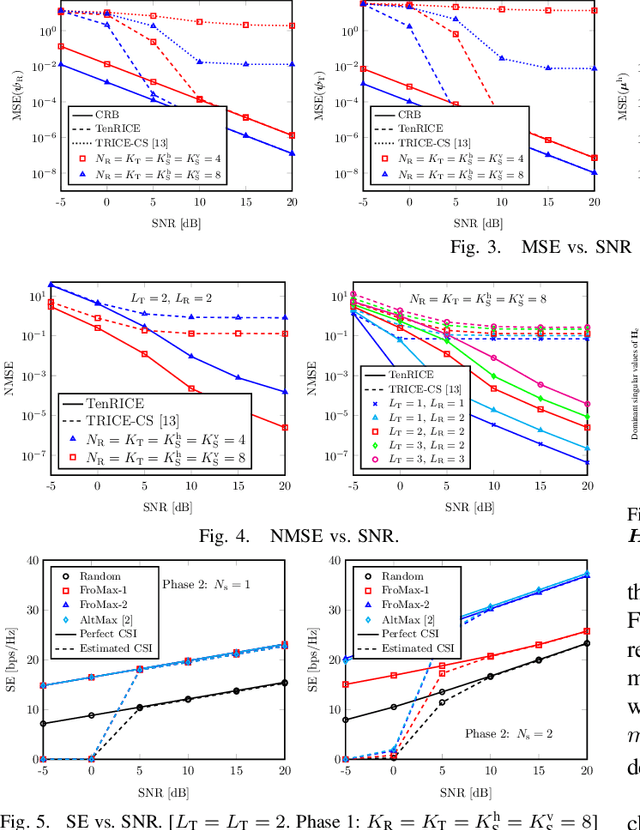
In this work, we consider both channel estimation and reflection design problems in point-to-point reconfigurable intelligent surface (RIS)-aided millimeter-wave (mmWave) MIMO communication systems. First, we show that by exploiting the low-rank nature of mmWave MIMO channels, the received training signals can be written as a low-rank multi-way tensor admitting a canonical polyadic CP decomposition. Utilizing such a structure, a tensor-based RIS channel estimation method (termed TenRICE) is proposed, wherein the tensor factor matrices are estimated using an alternating least squares method. Using TenRICE, the transmitter-to-RIS and the RIS-to-receiver channels are efficiently and separately estimated, up to a trivial scaling factor. After that, we formulate the beamforming and RIS reflection design as a spectral efficiency maximization problem. Due to its non-convexity, we propose a heuristic non-iterative two-step method, where the RIS reflection vector is obtained in a closed form using a Frobenius-norm maximization (FroMax) strategy. Our numerical results show that TenRICE has a superior performance, compared to benchmark methods, approaching the Cram\'er-Rao lower bound with a low training overhead. Moreover, we show that FroMax achieves a comparable performance to benchmark methods with a lower complexity.

Recovery under Side Constraints

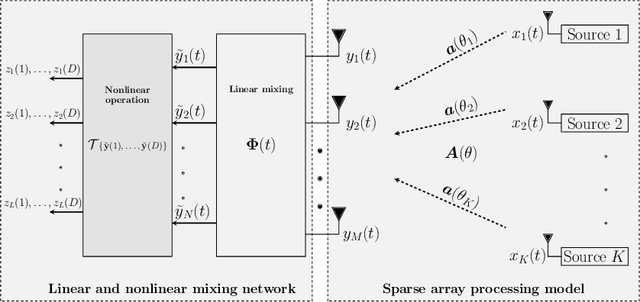
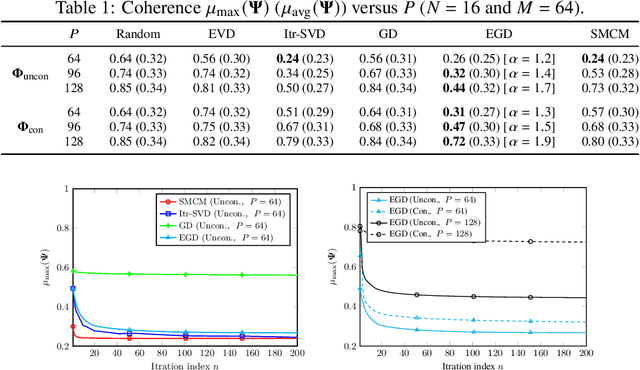
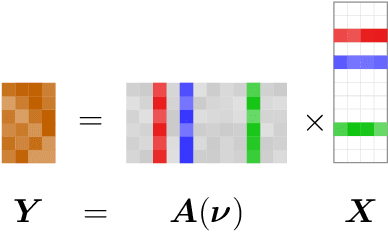
This paper addresses sparse signal reconstruction under various types of structural side constraints with applications in multi-antenna systems. Side constraints may result from prior information on the measurement system and the sparse signal structure. They may involve the structure of the sensing matrix, the structure of the non-zero support values, the temporal structure of the sparse representationvector, and the nonlinear measurement structure. First, we demonstrate how a priori information in form of structural side constraints influence recovery guarantees (null space properties) using L1-minimization. Furthermore, for constant modulus signals, signals with row-, block- and rank-sparsity, as well as non-circular signals, we illustrate how structural prior information can be used to devise efficient algorithms with improved recovery performance and reduced computational complexity. Finally, we address the measurement system design for linear and nonlinear measurements of sparse signals. Moreover, we discuss the linear mixing matrix design based on coherence minimization. Then we extend our focus to nonlinear measurement systems where we design parallel optimization algorithms to efficiently compute stationary points in the sparse phase retrieval problem with and without dictionary learning.

Fully Digital and Hybrid Beamforming Design For Millimeter-Wave MIMO-OFDM Two-Way Relaying Systems

In this work, we consider the design of hybrid analog-digital (HAD) multi-carrier MIMO-OFDM two-way relaying systems, where the relay station is equipped with a HAD amplify-and-forward architecture and every mobile station is equipped with a fully-digital beamforming architecture. We propose a sub-optimal solution by reformulating the original non-convex problem as a constrained Tucker2 decomposition with the objective of minimizing the sum Euclidean-norm between the HAD amplification matrices and their fully-digital counterparts. For the fully-digital amplification matrix design, we use a Frobenius-norm maximization of the effective channels on every subcarrier and propose an effective solution applicable for multi-stream communication scenarios. After that, we propose an alternating maximization (AltMax) HAD solution by exploiting the tensor structure of the reformulated problem. Simulation results are provided, where we show that the proposed fully-digital and AltMax-based HAD amplification matrix designs outperform some benchmark methods, especially for multi-stream communication scenarios.