Resolution and Relevance Trade-offs in Deep Learning

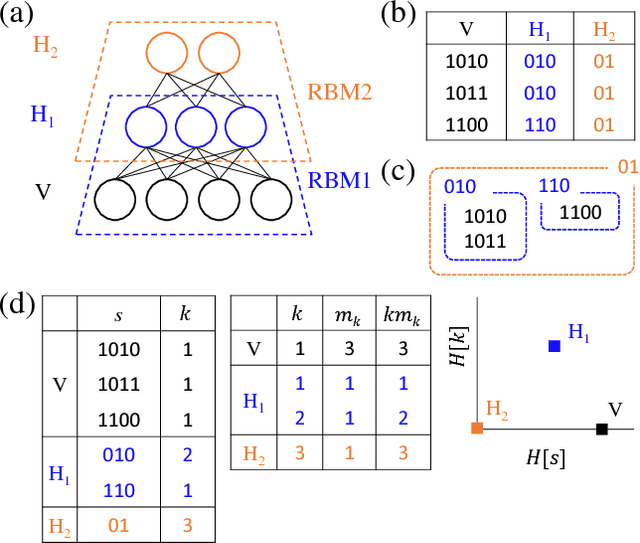
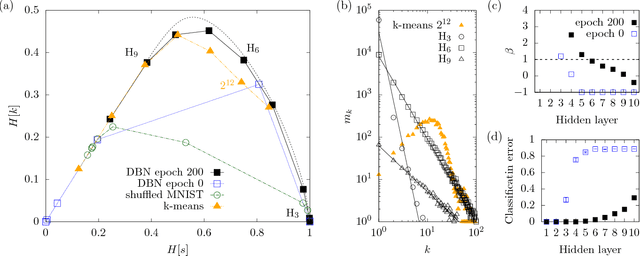
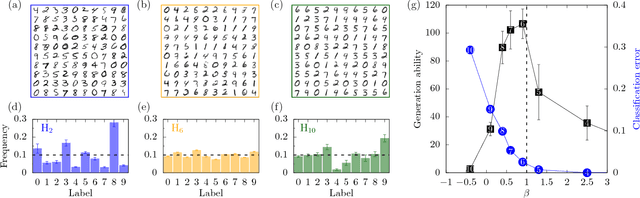
Deep learning has been successfully applied to various tasks, but its underlying mechanism remains unclear. Neural networks associate similar inputs in the visible layer to the same state of hidden variables in deep layers. The fraction of inputs that are associated to the same state is a natural measure of similarity and is simply related to the cost in bits required to represent these inputs. The degeneracy of states with the same information cost provides instead a natural measure of noise and is simply related the entropy of the frequency of states, that we call relevance. Representations with minimal noise, at a given level of similarity (resolution), are those that maximise the relevance. A signature of such efficient representations is that frequency distributions follow power laws. We show, in extensive numerical experiments, that deep neural networks extract a hierarchy of efficient representations from data, because they i) achieve low levels of noise (i.e. high relevance) and ii) exhibit power law distributions. We also find that the layer that is most efficient to reliably generate patterns of training data is the one for which relevance and resolution are traded at the same price, which implies that frequency distribution follows Zipf's law.

Minimal Perceptrons for Memorizing Complex Patterns

Feedforward neural networks have been investigated to understand learning and memory, as well as applied to numerous practical problems in pattern classification. It is a rule of thumb that more complex tasks require larger networks. However, the design of optimal network architectures for specific tasks is still an unsolved fundamental problem. In this study, we consider three-layered neural networks for memorizing binary patterns. We developed a new complexity measure of binary patterns, and estimated the minimal network size for memorizing them as a function of their complexity. We formulated the minimal network size for regular, random, and complex patterns. In particular, the minimal size for complex patterns, which are neither ordered nor disordered, was predicted by measuring their Hamming distances from known ordered patterns. Our predictions agreed with simulations based on the back-propagation algorithm.