Signal Recovery from Random Dot-Product Graphs Under Local Differential Privacy

We consider the problem of recovering latent information from graphs under $\varepsilon$-edge local differential privacy where the presence of relationships/edges between two users/vertices remains confidential, even from the data curator. For the class of generalized random dot-product graphs, we show that a standard local differential privacy mechanism induces a specific geometric distortion in the latent positions. Leveraging this insight, we show that consistent recovery of the latent positions is achievable by appropriately adjusting the statistical inference procedure for the privatized graph. Furthermore, we prove that our procedure is nearly minimax-optimal under local edge differential privacy constraints. Lastly, we show that this framework allows for consistent recovery of geometric and topological information underlying the latent positions, as encoded in their persistence diagrams. Our results extend previous work from the private community detection literature to a substantially richer class of models and inferential tasks.

Federated Analytics in Practice: Engineering for Privacy, Scalability and Practicality

Cross-device Federated Analytics (FA) is a distributed computation paradigm designed to answer analytics queries about and derive insights from data held locally on users' devices. On-device computations combined with other privacy and security measures ensure that only minimal data is transmitted off-device, achieving a high standard of data protection. Despite FA's broad relevance, the applicability of existing FA systems is limited by compromised accuracy; lack of flexibility for data analytics; and an inability to scale effectively. In this paper, we describe our approach to combine privacy, scalability, and practicality to build and deploy a system that overcomes these limitations. Our FA system leverages trusted execution environments (TEEs) and optimizes the use of on-device computing resources to facilitate federated data processing across large fleets of devices, while ensuring robust, defensible, and verifiable privacy safeguards. We focus on federated analytics (statistics and monitoring), in contrast to systems for federated learning (ML workloads), and we flag the key differences.

Perfect Spectral Clustering with Discrete Covariates

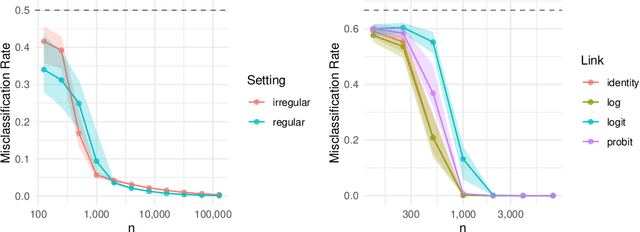
Among community detection methods, spectral clustering enjoys two desirable properties: computational efficiency and theoretical guarantees of consistency. Most studies of spectral clustering consider only the edges of a network as input to the algorithm. Here we consider the problem of performing community detection in the presence of discrete node covariates, where network structure is determined by a combination of a latent block model structure and homophily on the observed covariates. We propose a spectral algorithm that we prove achieves perfect clustering with high probability on a class of large, sparse networks with discrete covariates, effectively separating latent network structure from homophily on observed covariates. To our knowledge, our method is the first to offer a guarantee of consistent latent structure recovery using spectral clustering in the setting where edge formation is dependent on both latent and observed factors.