Minimax Data Sanitization with Distortion Constraint and Adversarial Inference

We study a privacy-preserving data-sharing setting where a privatizer transforms private data into a sanitized version observed by an authorized reconstructor and two unauthorized adversaries, each with access to side information correlated with the private data. The reconstructor is evaluated under a distortion function, while each adversary is evaluated using a separate loss function. The privatizer ensures the reconstructor distortion remains below a fixed threshold while maximizing the minimum loss across the two adversaries. This two-adversary setting models cases where individual users cannot reconstruct the data accurately, but their combined side information enables estimation within the distortion threshold. The privatizer maximizes individual loss while permitting accurate reconstruction only through collaboration. This echoes secret-sharing principles, but with lossy rather than perfect recovery. We frame this as a constrained data-driven minimax optimization problem and propose a data-driven training procedure that alternately updates the privatizer, reconstructor, and adversaries. We also analyze the Gaussian and binary cases as special scenarios where optimal solutions can be obtained. These theoretical optimal results are benchmarks for evaluating the proposed minimax training approach.

Context-Aware Search and Retrieval Over Erasure Channels

This paper introduces and analyzes a search and retrieval model that adopts key semantic communication principles from retrieval-augmented generation. We specifically present an information-theoretic analysis of a remote document retrieval system operating over a symbol erasure channel. The proposed model encodes the feature vector of a query, derived from term-frequency weights of a language corpus by using a repetition code with an adaptive rate dependent on the contextual importance of the terms. At the decoder, we select between two documents based on the contextual closeness of the recovered query. By leveraging a jointly Gaussian approximation for both the true and reconstructed similarity scores, we derive an explicit expression for the retrieval error probability, i.e., the probability under which the less similar document is selected. Numerical simulations on synthetic and real-world data (Google NQ) confirm the validity of the analysis. They further demonstrate that assigning greater redundancy to critical features effectively reduces the error rate, highlighting the effectiveness of semantic-aware feature encoding in error-prone communication settings.

Differentially-Private Collaborative Online Personalized Mean Estimation

We consider the problem of collaborative personalized mean estimation under a privacy constraint in an environment of several agents continuously receiving data according to arbitrary unknown agent-specific distributions. In particular, we provide a method based on hypothesis testing coupled with differential privacy and data variance estimation. Two privacy mechanisms and two data variance estimation schemes are proposed, and we provide a theoretical convergence analysis of the proposed algorithm for any bounded unknown distributions on the agents' data, showing that collaboration provides faster convergence than a fully local approach where agents do not share data. Moreover, we provide analytical performance curves for the case with an oracle class estimator, i.e., the class structure of the agents, where agents receiving data from distributions with the same mean are considered to be in the same class, is known. The theoretical faster-than-local convergence guarantee is backed up by extensive numerical results showing that for a considered scenario the proposed approach indeed converges much faster than a fully local approach, and performs comparably to ideal performance where all data is public. This illustrates the benefit of private collaboration in an online setting.

Straggler-Resilient Differentially-Private Decentralized Learning

We consider the straggler problem in decentralized learning over a logical ring while preserving user data privacy. Especially, we extend the recently proposed framework of differential privacy (DP) amplification by decentralization by Cyffers and Bellet to include overall training latency--comprising both computation and communication latency. Analytical results on both the convergence speed and the DP level are derived for both a skipping scheme (which ignores the stragglers after a timeout) and a baseline scheme that waits for each node to finish before the training continues. A trade-off between overall training latency, accuracy, and privacy, parameterized by the timeout of the skipping scheme, is identified and empirically validated for logistic regression on a real-world dataset.

Pliable Private Information Retrieval

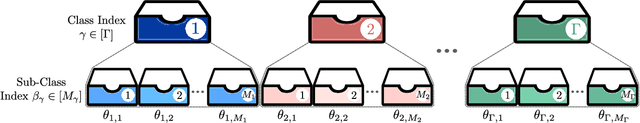
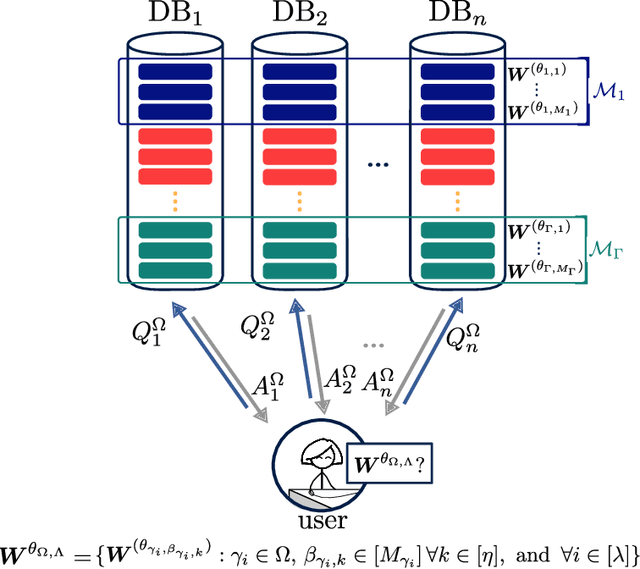
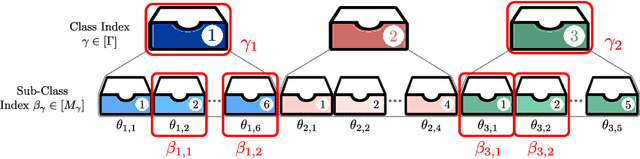
We formulate a new variant of the private information retrieval (PIR) problem where the user is pliable, i.e., interested in any message from a desired subset of the available dataset, denoted as pliable private information retrieval (PPIR). We consider a setup where a dataset consisting of $f$ messages is replicated in $n$ noncolluding databases and classified into $\Gamma$ classes. For this setup, the user wishes to retrieve any $\lambda\geq 1$ messages from multiple desired classes, i.e., $\eta\geq 1$, while revealing no information about the identity of the desired classes to the databases. We term this problem multi-message PPIR (M-PPIR) and introduce the single-message PPIR (PPIR) problem as an elementary special case of M-PPIR. We first derive converse bounds on the M-PPIR rate, which is defined as the ratio of the desired amount of information and the total amount of downloaded information, followed by the corresponding achievable schemes. As a result, we show that the PPIR capacity, i.e., the maximum achievable PPIR rate, for $n$ noncolluding databases matches the capacity of PIR with $n$ databases and $\Gamma$ messages. Thus, enabling flexibility, i.e., pliability, where privacy is only guaranteed for classes, but not for messages as in classical PIR, allows to trade-off privacy versus download rate. A similar insight is shown to hold for the general case of M-PPIR.