Supervised Learning for Multi-Block Incomplete Data

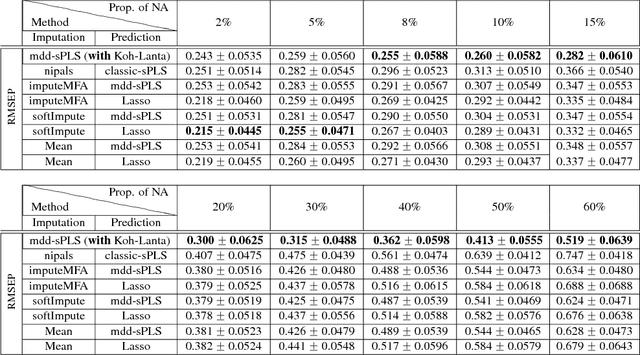
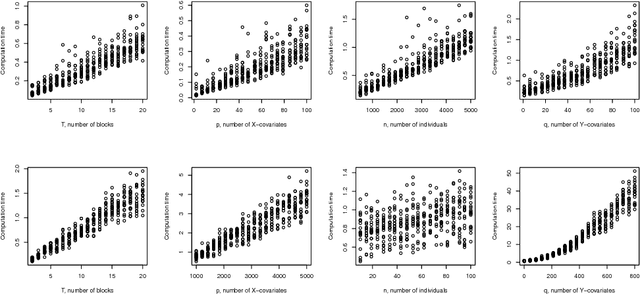
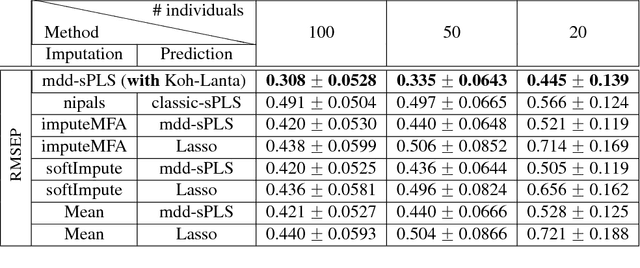
In the supervised high dimensional settings with a large number of variables and a low number of individuals, one objective is to select the relevant variables and thus to reduce the dimension. That subspace selection is often managed with supervised tools. However, some data can be missing, compromising the validity of the sub-space selection. We propose a Partial Least Square (PLS) based method, called Multi-block Data-Driven sparse PLS mdd-sPLS, allowing jointly variable selection and subspace estimation while training and testing missing data imputation through a new algorithm called Koh-Lanta. This method was challenged through simulations against existing methods such as mean imputation, nipals, softImpute and imputeMFA. In the context of supervised analysis of high dimensional data, the proposed method shows the lowest prediction error of the response variables. So far this is the only method combining data imputation and response variable prediction. The superiority of the supervised multi-block mdd-sPLS method increases with the intra-block and inter-block correlations. The application to a real data-set from a rVSV-ZEBOV Ebola vaccine trial revealed interesting and biologically relevant results. The method is implemented in a R-package available on the CRAN and a Python-package available on pypi.

ClustGeo: an R package for hierarchical clustering with spatial constraints

In this paper, we propose a Ward-like hierarchical clustering algorithm including spatial/geographical constraints. Two dissimilarity matrices $D_0$ and $D_1$ are inputted, along with a mixing parameter $\alpha \in [0,1]$. The dissimilarities can be non-Euclidean and the weights of the observations can be non-uniform. The first matrix gives the dissimilarities in the "feature space" and the second matrix gives the dissimilarities in the "constraint space". The criterion minimized at each stage is a convex combination of the homogeneity criterion calculated with $D_0$ and the homogeneity criterion calculated with $D_1$. The idea is then to determine a value of $\alpha$ which increases the spatial contiguity without deteriorating too much the quality of the solution based on the variables of interest i.e. those of the feature space. This procedure is illustrated on a real dataset using the R package ClustGeo.

On central tendency and dispersion measures for intervals and hypercubes

The uncertainty or the variability of the data may be treated by considering, rather than a single value for each data, the interval of values in which it may fall. This paper studies the derivation of basic description statistics for interval-valued datasets. We propose a geometrical approach in the determination of summary statistics (central tendency and dispersion measures) for interval-valued variables.